Let f(x)=∫x0(t−1)(t−2)2dt, then find a point of minimum.
Video Solution
Text Solution
f(x)=x∫0(t−1)(t−2)2dt
f'(x)=(x−1)(x−2)2
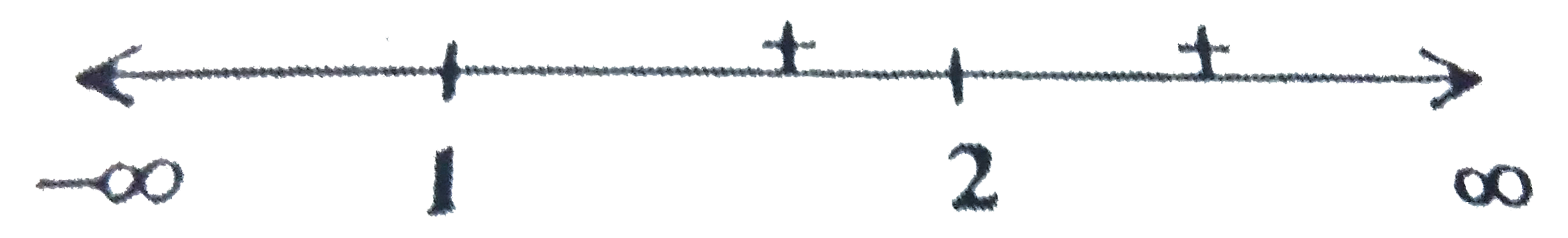
⇒=1 is the point of minimum
f(1)=1∫0(t3−5t2+8t−4)dt=14−53+4−4=−1712. Hence (1,−1712) is a point of minimum.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - DEFINITE INTEGRATION & ITS APPLICATION EXERCISE - Self practive problem106videos
DEFINITE INTEGRATION & ITS APPLICATION
- BOOK - RESONANCE ENGLISHCHAPTER - DEFINITE INTEGRATION & ITS APPLICATION EXERCISE - Exercise 164videos
DEFINITE INTEGRATION & ITS APPLICATION
- BOOK - RESONANCE ENGLISHCHAPTER - COMBINATORICSEXERCISE - Exercise-2 (Part-II: Previously Asked Question of RMO)5videos
COMBINATORICS
- BOOK - RESONANCE ENGLISHCHAPTER - DPPEXERCISE - QUESTION656videos
DPP
Similar Questions
If f(x)=∫x2dt1+t4, then
View SolutionLet f(x)=∫x0|2t−3|dt, then f is
View SolutionLet f(x)=∫x0et(t−1)(t−2)dt. Then, f decreases in the interval
View SolutionLet f(x)=1x2∫x4(4t2−2f'(t))dt then find 9f'(4)
View SolutionGiven a function g, continous everywhere such that g(1)=5and∫10g(t)dt=2. If f(x)=12∫x0(x−t)2g(t)dt, then find the value of f'(1)+f''(1).
View SolutionLet f(x)=∫x13t1+t2dt, where x>0, Then
View SolutionLet f(x)=∫x−2|t+1|dt, then
View SolutionLet f(x)=∫x2f(t2−3t+4)dt. Then
View SolutionIf f(x)=∫x2+1x2e−t2dt , then find the interval in which f(x) increases.
View SolutionConsider the function f(x)andg(x), both defined from R→R
f(x)=x32+1−x∫x0g(t)dtandg(x)=x−∫10f(t)dt, then
The number of points of intersection of f(x)andg(x) is/are:View Solution

