Let f:R→(0,1) be a continuous function. Then, which of the following function(s) has (have) the value of zero at some point in the interval (0,1)?
ex−x∫0f(t)sintdt
f(x)+π2∫0f(t)sintdt
x−π2−x∫0costdt
x9−f(x)
The correct Answer is:CD
Step by step video & image solution for Let f:Rto(0,1) be a continuous function. Then, which of the following function(s) has (have) the value of zero at some point in the interval (0,1)? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- DEFINITE INTEGRATION & ITS APPLICATION BOOK - RESONANCE ENGLISHCHAPTER - DEFINITE INTEGRATION & ITS APPLICATION EXERCISE - Exercise 3 Part - II
25 Videos
- DEFINITE INTEGRATION & ITS APPLICATION BOOK - RESONANCE ENGLISHCHAPTER - DEFINITE INTEGRATION & ITS APPLICATION EXERCISE - High Level Problem
26 Videos
- DEFINITE INTEGRATION & ITS APPLICATION BOOK - RESONANCE ENGLISHCHAPTER - DEFINITE INTEGRATION & ITS APPLICATION EXERCISE - Exercise 2 Part - IV
6 Videos
- COMBINATORICSBOOK - RESONANCE ENGLISHCHAPTER - COMBINATORICSEXERCISE - Exercise-2 (Part-II: Previously Asked Question of RMO)
5 Videos
- DPPBOOK - RESONANCE ENGLISHCHAPTER - DPPEXERCISE - QUESTION
656 Videos
Similar Questions
Let f:R−−→0,1 be a continuous function. Then, which of the following function (s) has (have) the value zero at some point in the interval (0,1)? ex−∫x0f(t)sintdt (b) f(x)+∫π20f(t)sintdt x−∫π2−x0f(t)costdt (d) x9−f(x)
View SolutionWhich of the following functions (s) has/have the range equals [-1, 1]?
View Solution
Knowledge Check
Which of the following functions have zeros -1, 1, and 4?
Af(x)=(x−4)(1+x2)Bf(x)=(x+4)(1−x2)Cf(x)=(x−1)(x2−3x−4)Df(x)=(x−1)(x2+3x−4)
Which of the following graphs represent function whose derivatives have a maximum in the interval (0,1) ?
View SolutionLet f:R→R be a continuous function which satisfies f(x)= ∫x0f(t)dt. Then the value of f(1n5) is______
View SolutionLet f,g:[−1,2]→ be continuous functions which are twice differentiable on the interval (-1,2). Let the values of f and g at the points and 2 be as given in the following table:
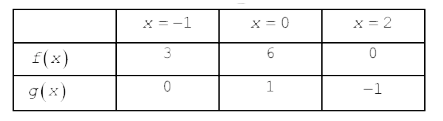
In each of the intervals (-1,0) and (0, 2) the function (f-3g) never vanishes. Then the correct statements(s) is(are) :
View SolutionVerify Lagrange's Mean Value theorem for the following functions in the given intervals
f(x) = ex in the interval [0,1].
View SolutionLet f:[0,1]→[0,1] be a continuous function. Then prove that f(x)=x for at least one 0≤x≤1.
View SolutionVerify Lagrange's Mean Value theorem for the following functions in the given intervals
f(x) = tan−1x in the interval [0,1]
View SolutionLet f be a continuous function on R such that f(14n)=sinenen2+n2n2+1 Then the value of f(0) is
View Solution
