(i) Find whether f(x)=x+cosx is one-one.
(ii) Identify whether the function f(x)=−x3+3x2−2x+4 for f:R→R is ONTO or INTO
(iii) f(x)=x2−2x,[0,3]→A. Find whether f(x) is injective or not. Also find the set A if f(x) is surjective
Video Solution
Text Solution
(i) The domain of f(x) is R . f'(x)=1−sinx
∴F'(x)≥0∀xε complete domain and equality holds at discrete points only
∴f(x) is strictly increasing on R. Hence f(x) is one-one.
(ii) As range ≡ codomain, therefore given function is ONTO
(iii) f'(x)=2(x−1),0≤x≤3
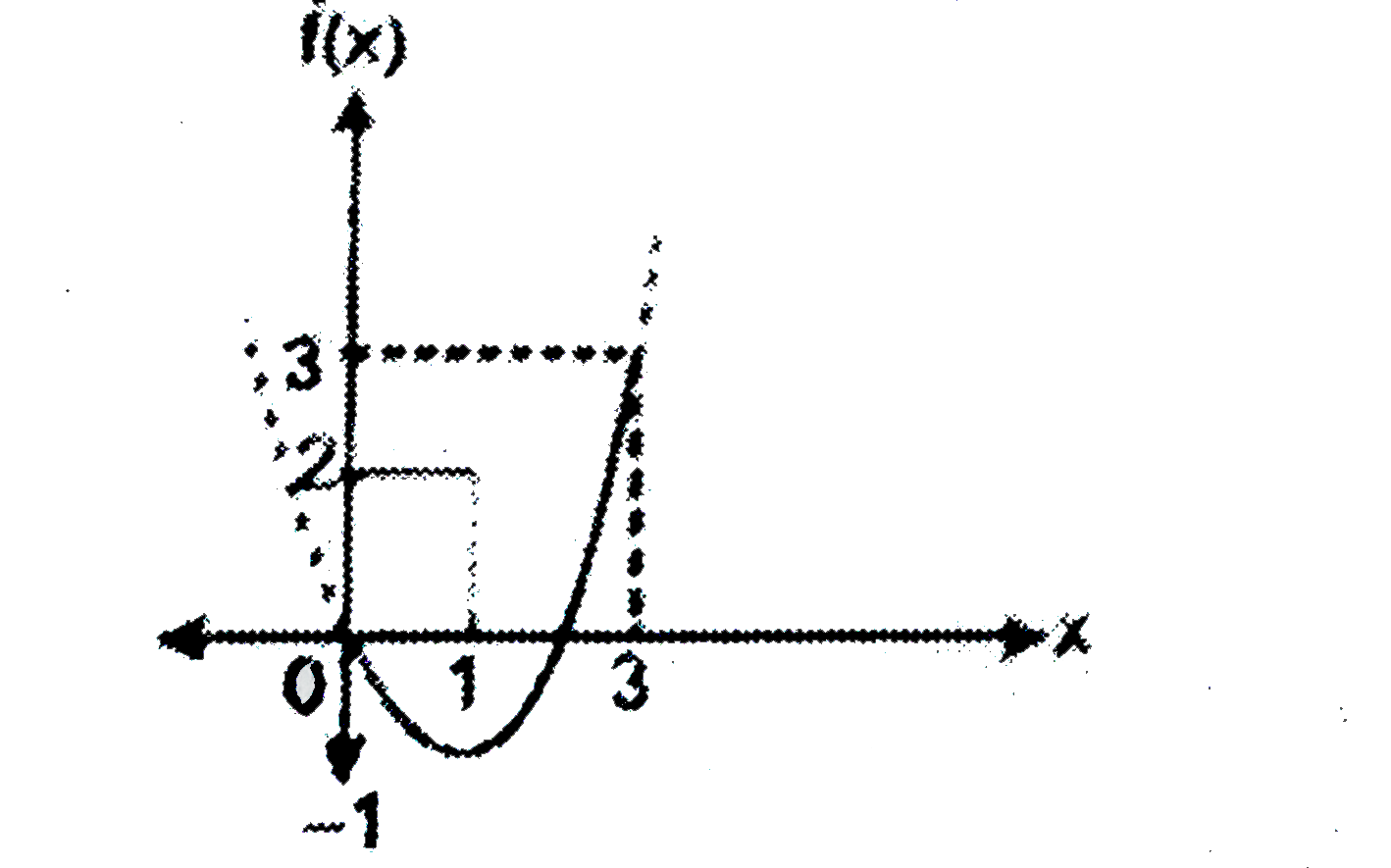
∴f'(x)={−ve0≤x<1+ve1<x<3
∴f(x) is non monotonic. Hence it is not injective.
For f(x) to be surjective, A should be equal to its range. By graph range is [−1,3]
∴A≡[−1,3]
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - RELATION, FUNCTION & ITFEXERCISE - SUBJECTIVE_TYPE179videos
RELATION, FUNCTION & ITF
- BOOK - RESONANCE ENGLISHCHAPTER - RELATION, FUNCTION & ITFEXERCISE - SCQ_TYPE96videos
RELATION, FUNCTION & ITF
- BOOK - RESONANCE ENGLISHCHAPTER - NUMBER THEORYEXERCISE - Exercise -2 (PART - II)4videos
NUMBER THEORY
- BOOK - RESONANCE ENGLISHCHAPTER - SEQUENCE & SERIES EXERCISE - EXERCISE -2 (PART-II : PREVIOUSLY ASKED QUESTION OF RMO)3videos
SEQUENCE & SERIES
Similar Questions
Find whether f(x)=x3 forms a mapping or not.
View SolutionLet f(x)=x3−3x2+2x Find f'(x)
View SolutionDetermine whether f(x)=2x+34 for f:R→R is invertible or not . If so find it.
View SolutionShow that the function f(x)=3x+2 is one-one and onto
View SolutionFind whether the following functions are one-one or not.
f:R→R, defined by f(x)=x3,x∈RView SolutionFind whether f(x) is one-one or many-one & into or onto if f:D->R where D is its domain. f(x)=34x2−cosπx
View SolutionIf (x) {x2x≠0,4x=0 then find whether f(x) is constinuosus at x=0
View SolutionLet f:[−π/2,π/2]→[−1,1] where f(x)=sinx. Find whether f(x) is one-one or not.
View SolutionLet f(x)=x4−x3 then find f '(2)
View SolutionExamine whether the following functions are one-one, many-one or into, onto f(x)=x2+lnx
View Solution

