In case of earth
gravitational field is zero both at centre and infinity
gravitational potential is zero, both at centre and infinity
gravitational potential is same both at centre and infinity but not zero
gravitational potential is minimum at the centre
The correct Answer is:A, D
To solve the question regarding the gravitational field and gravitational potential in the case of Earth, we will analyze both concepts step by step.
Step 1: Understanding Gravitational Field at Different Points
- The gravitational field (g) at a point is defined as the force experienced by a unit mass placed at that point.
- At the center of the Earth, the gravitational field is zero. This is because the mass of the Earth exerts equal gravitational forces in all directions, resulting in a net force of zero.
- At a depth d below the surface of the Earth, the gravitational field can be calculated considering only the mass of the Earth that is at a radius less than r. The mass outside this radius does not contribute to the gravitational field at that point.
Hint for Step 1: Remember that inside a uniform spherical shell, the gravitational field is zero.
Step 2: Gravitational Field at Infinity
- The gravitational field at infinity is also zero. This is because as the distance r from the mass increases, the gravitational field g is given by the formula:
g=GMr2
As r approaches infinity, g approaches zero.
Hint for Step 2: Think about how gravitational force diminishes with distance.
Step 3: Gravitational Potential at the Center of the Earth
- Gravitational potential (V) is defined as the work done in bringing a unit mass from infinity to that point.
- The formula for gravitational potential at a distance r from the center of a mass M is:
V=−GMr
- At the center of the Earth, the gravitational potential can be derived from integrating the gravitational field. It is given by:
V=−3GM2R
where R is the radius of the Earth. This value is negative, indicating that it is a bound system.
Hint for Step 3: Remember that gravitational potential is always negative and reaches its minimum at the center.
Step 4: Conclusion on Gravitational Potential
- Since the gravitational potential is negative and reaches its minimum value at the center of the Earth, we conclude that the gravitational potential is minimum at the center.
- Therefore, the correct options are:
- Gravitational field is zero at the center and at infinity.
- Gravitational potential is minimum at the center.
Final Answer:
- Gravitational field is zero at the center and at infinity (Option A).
- Gravitational potential is minimum at the center (Option D).
- The gravitational field (g) at a point is defined as the force experienced by a unit mass placed at that point.
- At the center of the Earth, the gravitational field is zero. This is because the mass of the Earth exerts equal gravitational forces in all directions, resulting in a net force of zero.
- At a depth d below the surface of the Earth, the gravitational field can be calculated considering only the mass of the Earth that is at a radius less than r. The mass outside this radius does not contribute to the gravitational field at that point.
- The gravitational field at infinity is also zero. This is because as the distance r from the mass increases, the gravitational field g is given by the formula:
g=GMr2
As r approaches infinity, g approaches zero.
- Gravitational potential (V) is defined as the work done in bringing a unit mass from infinity to that point.
- The formula for gravitational potential at a distance r from the center of a mass M is:
V=−GMr
- At the center of the Earth, the gravitational potential can be derived from integrating the gravitational field. It is given by:
V=−3GM2R
where R is the radius of the Earth. This value is negative, indicating that it is a bound system.
- Since the gravitational potential is negative and reaches its minimum value at the center of the Earth, we conclude that the gravitational potential is minimum at the center.
- Therefore, the correct options are:
- Gravitational field is zero at the center and at infinity.
- Gravitational potential is minimum at the center.
- Gravitational field is zero at the center and at infinity (Option A).
- Gravitational potential is minimum at the center (Option D).
Topper's Solved these Questions
- GRAVITATIONBOOK - RESONANCE ENGLISHCHAPTER - GRAVITATIONEXERCISE - EXERCISE-2 PART-4
9 Videos
- GRAVITATIONBOOK - RESONANCE ENGLISHCHAPTER - GRAVITATIONEXERCISE - EXERCISE-3 PART-1
15 Videos
- GRAVITATIONBOOK - RESONANCE ENGLISHCHAPTER - GRAVITATIONEXERCISE - EXERCISE-2 PART-2
10 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Advance level Problems
35 Videos
- NUCLEAR PHYSICSBOOK - RESONANCE ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - Advanced level solutions
16 Videos
Similar Questions
Assertion : A particle is projected upwards with speed υ and it goes to a heigth h. If we double the speed then it will move to height 4h.
Reason : In case of earth, acceleration due to gravity g varies as
g∝1r2 (for r≥R)
View SolutionEarthquakes generate sound waves inside the earth. In case of the earth, both transverse (S) and longitudinal (P) waves can propagate. Typically, the speed of S waves is about 4.5 km s−1 and that of P waves is 8.0 km s−1. A seismograph records both P and S waves from an earthquake. this difference helps us to find the distanec of the point of origin of the earthquake. this point is called the epicenter.
Q. If only 2 seismograph readings are available, how may probable locations of an epicentre could be detected?
View SolutionEarthquakes generate sound waves inside the earth. In case of the earth, both transverse (S) and longitudinal (P) waves can propagate. Typically, the speed of S waves is about 4.5 km s−1 and that of P waves is 8.0 km s−1. A seismograph records both P and S waves from an earthquake. this difference helps us to find the distanec of the point of origin of the earthquake. this point is called the epicenter.
Q. if at the location of a seismograph the P waevs arrive 2 minute earlier, the distance of the epicenter from the location of the seismograph is
View SolutionEarthquakes generate sound waves inside the earth. In case of the earth, both transverse (S) and longitudinal (P) waves can propagate. Typically, the speed of S waves is about 4.5 km s−1 and that of P waves is 8.0 km s−1. A seismograph records both P and S waves from an earthquake. this difference helps us to find the distanec of the point of origin of the earthquake. this point is called the epicenter.
Q. The reading of the time lag between the arrival of S and P waves gives us the distance of the epicenter from the location of a seismograph. the readings of what minimum number of seismographs would be necessary to pinpoint the location of an epicenter?
View SolutionIn case of second's pendulum, match the following (consider shape of earth also)
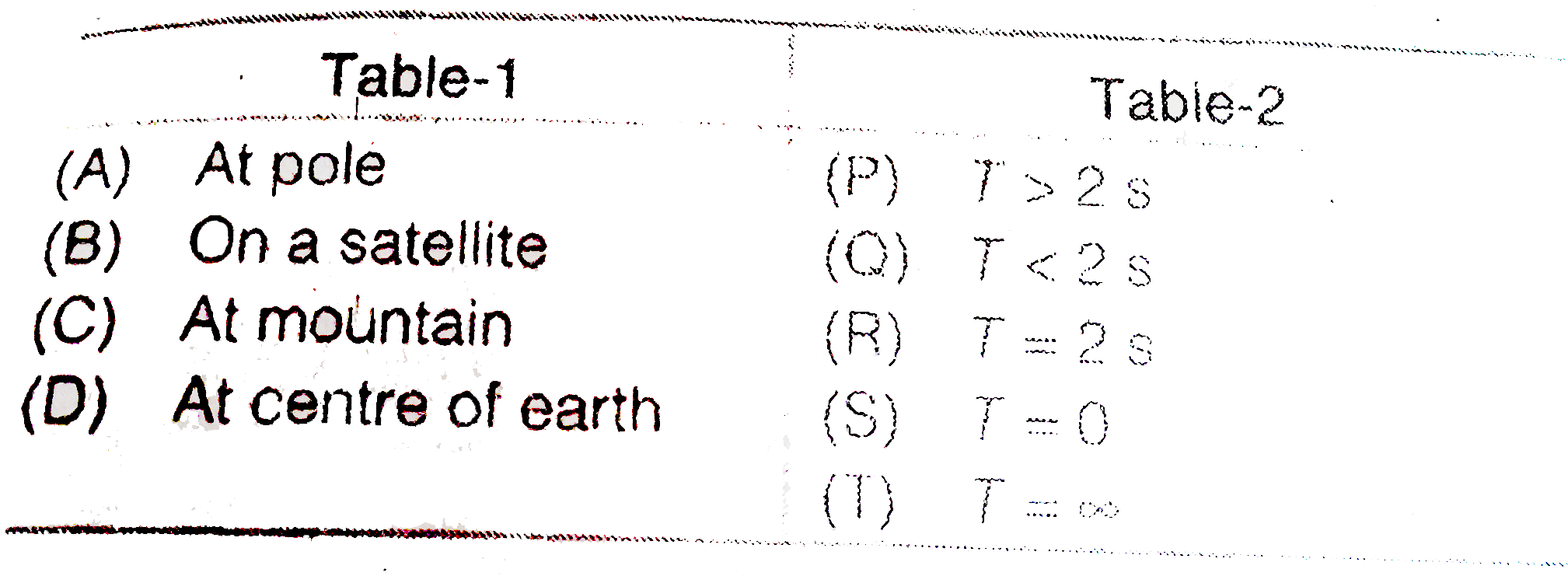
View SolutionA TV transmission tower of antenna is at a height of 20 m. How much service area can it cover if the receiving antenna is (i) at ground level (ii) at a height of 25 m ? Calculate the percentage increase in area covered in case (ii) relative to case (i). Radius of earth =6.4×106m
View SolutionAt what height above the earth's surface the acceleration due to gravity will be 1/9 th of its value at the earth’s surface? Radius of earth is 6400 km.
View SolutionIf the satellite is stopped suddenly in its orbit which is at a distnace = radius of earth from earth’s surface and allowed to fall freely into the earth, the speed with which it hits the surface of earth will be -
View SolutionA tunnel is dug across the diameter of earth. A ball is released from the surface of Earth into the tunnel. The velocity of ball when it is at a distance R2 from centre of earth is (where R = radius of Earth and M = mass of Earth)
View SolutionIn case of a liquid
View Solution
