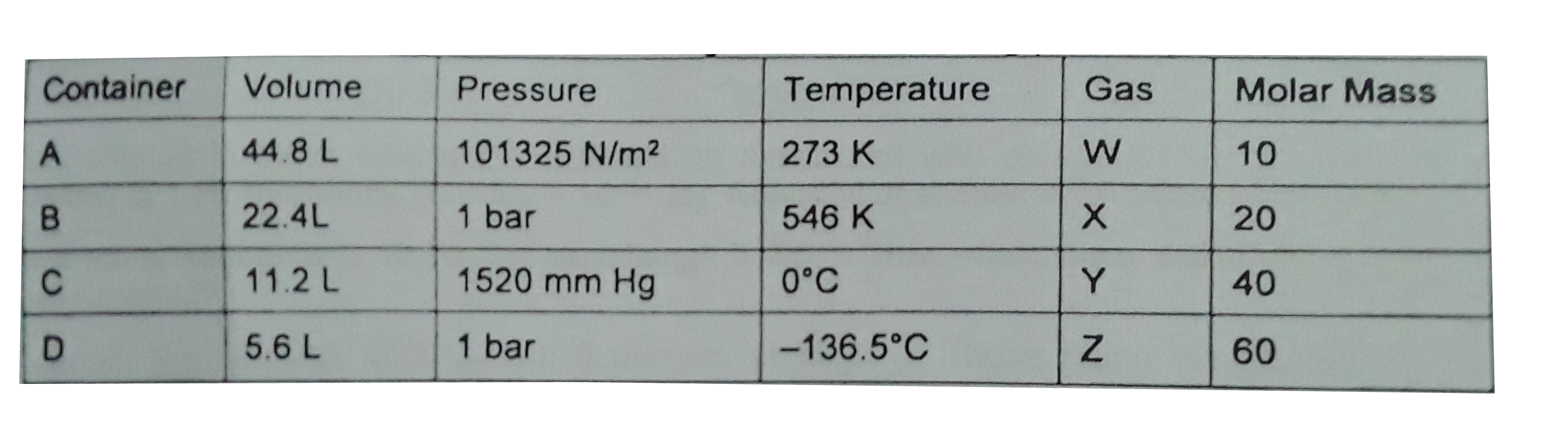
Three different containers contain three different gases with following parameter
Container 1 of 67.2 L containing gas X at 0∘C and 1.01325 bar
Container 2 of 44. 8L contaning gas Y at 546 K & 760 mm of Hg
Container 3 of 89.6 L containing Z gas at 101325 N/m2&0∘C
Now they are mixed in single container. If gram molecular mass of X ,Y & Z are 10 g/mol, 20 g/mol and 40 g/mol respectively Then which of the following statements are correct
Mole percent of gas Z in final mixture is 50%
Average molecular mass of final mixture is 26.25 amu
Mole ratio of gas X and gas Z is 3:4
Number of molecules present in final mixture is equal to number of atoms in 320 g Ca
To solve the problem, we will follow these steps:
Step 1: Calculate the number of moles of each gas using the Ideal Gas Law.
The Ideal Gas Law is given by the equation:
PV=nRT
Where:
- P = pressure (in atm)
- V = volume (in liters)
- n = number of moles
- R = universal gas constant = 0.0821 L·atm/(K·mol)
- T = temperature (in Kelvin)
For Gas X:
- Volume (V) = 67.2 L
- Pressure (P) = 1.01325 bar = 1 atm (since 1 bar ≈ 1 atm)
- Temperature (T) = 0°C = 273 K
Using the formula:
nX=PVRT=(1atm)(67.2L)(0.0821L⋅atm/(K⋅mol))(273K)
Calculating this gives:
nX=67.222.414≈3moles
For Gas Y:
- Volume (V) = 44.8 L
- Pressure (P) = 760 mmHg = 1 atm
- Temperature (T) = 546 K
Using the formula:
nY=PVRT=(1atm)(44.8L)(0.0821L⋅atm/(K⋅mol))(546K)
Calculating this gives:
nY≈1mole
For Gas Z:
- Volume (V) = 89.6 L
- Pressure (P) = 101325 N/m² = 1 atm
- Temperature (T) = 0°C = 273 K
Using the formula:
nZ=PVRT=(1atm)(89.6L)(0.0821L⋅atm/(K⋅mol))(273K)
Calculating this gives:
nZ=89.622.414≈4moles
Step 2: Calculate the total number of moles.
ntotal=nX+nY+nZ=3+1+4=8moles
Step 3: Calculate the mole percentages of each gas.
- Mole percentage of gas X:
Mole percent of X=(nXntotal)×100=(38)×100=37.5%
- Mole percentage of gas Y:
Mole percent of Y=(nYntotal)×100=(18)×100=12.5%
- Mole percentage of gas Z:
Mole percent of Z=(nZntotal)×100=(48)×100=50%
Step 4: Calculate the average molecular mass of the mixture.
Using the formula:
Average molecular mass=(nX⋅MX)+(nY⋅MY)+(nZ⋅MZ)ntotal
Where:
- MX=10g/mol
- MY=20g/mol
- MZ=40g/mol
Calculating:
Average molecular mass=(3⋅10)+(1⋅20)+(4⋅40)8=30+20+1608=2108=26.25g/mol
Step 5: Determine the mole ratio of gas X to gas Z.
Mole ratio of X to Z=nXnZ=34or3:4
Step 6: Calculate the number of molecules in the final mixture.
Using Avogadro's number (6.022×1023):
Total number of molecules=ntotal×NA=8×6.022×1023
Step 7: Calculate the number of moles of calcium in 320 g.
Moles of Ca=320g40g/mol=8moles
Thus, the number of atoms in 320 g of calcium is also 8×6.022×1023.
Conclusion:
All statements provided in the question are true:
1. Mole percent of Z is 50%.
2. Average molecular mass is 26.25 g/mol.
3. Mole ratio of X to Z is 3:4.
4. Number of molecules in the final mixture equals the number of atoms in 320 g of calcium.
PV=nRT
Where:
- P = pressure (in atm)
- V = volume (in liters)
- n = number of moles
- R = universal gas constant = 0.0821 L·atm/(K·mol)
- T = temperature (in Kelvin)
- Volume (V) = 67.2 L
- Pressure (P) = 1.01325 bar = 1 atm (since 1 bar ≈ 1 atm)
- Temperature (T) = 0°C = 273 K
nX=PVRT=(1atm)(67.2L)(0.0821L⋅atm/(K⋅mol))(273K)
Calculating this gives:
nX=67.222.414≈3moles
- Volume (V) = 44.8 L
- Pressure (P) = 760 mmHg = 1 atm
- Temperature (T) = 546 K
nY=PVRT=(1atm)(44.8L)(0.0821L⋅atm/(K⋅mol))(546K)
Calculating this gives:
nY≈1mole
- Volume (V) = 89.6 L
- Pressure (P) = 101325 N/m² = 1 atm
- Temperature (T) = 0°C = 273 K
nZ=PVRT=(1atm)(89.6L)(0.0821L⋅atm/(K⋅mol))(273K)
Calculating this gives:
nZ=89.622.414≈4moles
ntotal=nX+nY+nZ=3+1+4=8moles
- Mole percentage of gas X:
Mole percent of X=(nXntotal)×100=(38)×100=37.5%
Mole percent of Y=(nYntotal)×100=(18)×100=12.5%
Mole percent of Z=(nZntotal)×100=(48)×100=50%
Using the formula:
Average molecular mass=(nX⋅MX)+(nY⋅MY)+(nZ⋅MZ)ntotal
Where:
- MX=10g/mol
- MY=20g/mol
- MZ=40g/mol
Average molecular mass=(3⋅10)+(1⋅20)+(4⋅40)8=30+20+1608=2108=26.25g/mol
Mole ratio of X to Z=nXnZ=34or3:4
Using Avogadro's number (6.022×1023):
Total number of molecules=ntotal×NA=8×6.022×1023
Moles of Ca=320g40g/mol=8moles
Thus, the number of atoms in 320 g of calcium is also 8×6.022×1023.
All statements provided in the question are true:
1. Mole percent of Z is 50%.
2. Average molecular mass is 26.25 g/mol.
3. Mole ratio of X to Z is 3:4.
4. Number of molecules in the final mixture equals the number of atoms in 320 g of calcium.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Gober gas contains mainly
ACH4 and O2BCH4 and O2CCH4 and H2DCH4 and SO2
Producer gas contains
View SolutionGas containing double bond.
View SolutionA bulb contains 1.6g of O2 contains.
View SolutionGrapes placed in three beakers X ,Y and Z containing different type of solutions are shown in figures.
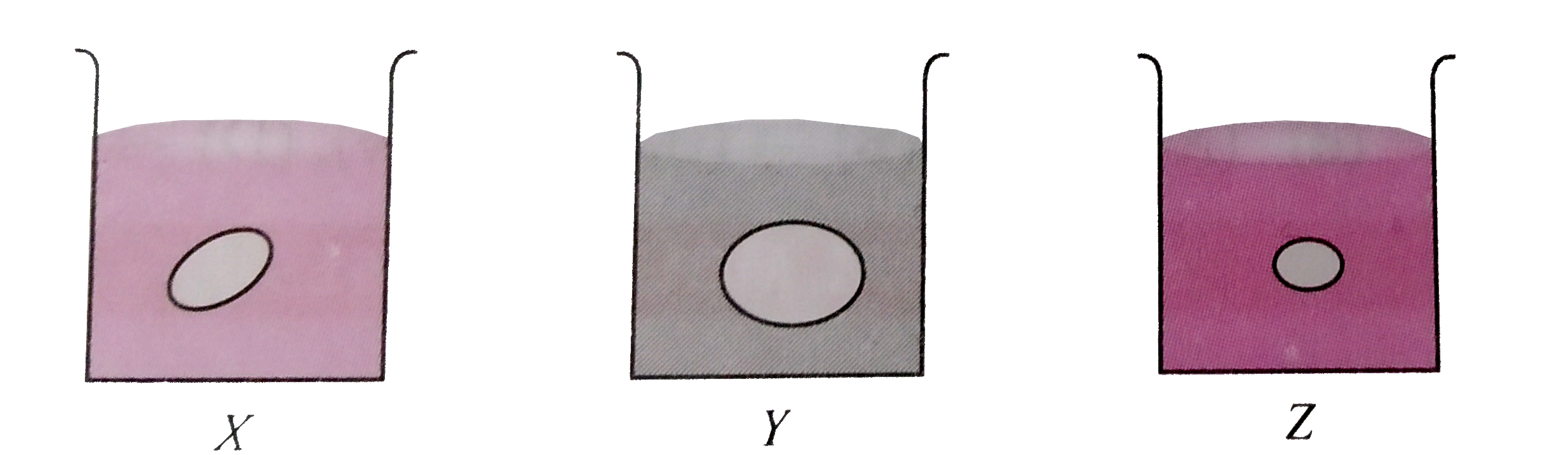
If beaker X contains water, Y and Z contain
View SolutionThe pressure of sodium vapour in a 1.0 L container is 10 torr at 1000∘C. How many atoms are in the container?
View SolutionMarsh gas mainly contains:
View SolutionMarsh gas mainly contains:
View Solution