Find the sum of number of geometrical isomers for following complexes.
(a)[CoCl2Br2]2− (b)[Rh(en)3]3+ (c)[Cr(en)2Br2]+
(d)[Pt en Cl_(2)] "
The correct Answer is:4(0+0+2+0+2=4)
To find the sum of the number of geometrical isomers for the given complexes, we will analyze each complex one by one.
Step 1: Analyze the complex (CoCl2Br2)2−
- Type of complex: Octahedral
- Ligands: 2 Chloride (Cl) and 2 Bromide (Br)
- Geometrical isomers: For octahedral complexes with two pairs of different ligands, we can have cis and trans isomers.
- Possible isomers: The two Cl and two Br can be arranged in two ways:
- Cis isomer: Both Cl ligands are adjacent.
- Trans isomer: Cl ligands are opposite each other.
Thus, the number of geometrical isomers for (CoCl2Br2)2− is 2.
Step 2: Analyze the complex (Rh(en)3)3+
- Type of complex: Octahedral
- Ligands: 3 Ethylenediamine (en) ligands
- Geometrical isomers: Since ethylenediamine is a bidentate ligand, all three ligands are identical.
- Possible isomers: There are no geometrical isomers possible because all ligands are the same.
Thus, the number of geometrical isomers for (Rh(en)3)3+ is 0.
Step 3: Analyze the complex (Cr(en)2Br2)+
- Type of complex: Octahedral
- Ligands: 2 Ethylenediamine (en) and 2 Bromide (Br)
- Geometrical isomers: Similar to the first complex, we can have cis and trans arrangements.
- Possible isomers:
- Cis isomer: Both Br ligands are adjacent.
- Trans isomer: Br ligands are opposite each other.
Thus, the number of geometrical isomers for (Cr(en)2Br2)+ is 2.
Step 4: Analyze the complex (Pt(en)Cl2)
- Type of complex: Square planar
- Ligands: 1 Ethylenediamine (en) and 2 Chloride (Cl)
- Geometrical isomers: For square planar complexes with two different ligands, we can have:
- Cis isomer: Both Cl ligands are adjacent.
- Trans isomer: Cl ligands are opposite each other.
Thus, the number of geometrical isomers for (Pt(en)Cl2) is 2.
Final Calculation
Now, we sum up the number of geometrical isomers from all complexes:
- (CoCl2Br2)2−: 2
- (Rh(en)3)3+: 0
- (Cr(en)2Br2)+: 2
- (Pt(en)Cl2): 2
Total = 2 + 0 + 2 + 2 = 6
Conclusion
The sum of the number of geometrical isomers for the given complexes is 6.
---
- Ligands: 2 Chloride (Cl) and 2 Bromide (Br)
- Geometrical isomers: For octahedral complexes with two pairs of different ligands, we can have cis and trans isomers.
- Possible isomers: The two Cl and two Br can be arranged in two ways:
- Cis isomer: Both Cl ligands are adjacent.
- Trans isomer: Cl ligands are opposite each other.
Thus, the number of geometrical isomers for (CoCl2Br2)2− is 2.
- Ligands: 3 Ethylenediamine (en) ligands
- Geometrical isomers: Since ethylenediamine is a bidentate ligand, all three ligands are identical.
- Possible isomers: There are no geometrical isomers possible because all ligands are the same.
- Ligands: 2 Ethylenediamine (en) and 2 Bromide (Br)
- Geometrical isomers: Similar to the first complex, we can have cis and trans arrangements.
- Possible isomers:
- Cis isomer: Both Br ligands are adjacent.
- Trans isomer: Br ligands are opposite each other.
- Ligands: 1 Ethylenediamine (en) and 2 Chloride (Cl)
- Geometrical isomers: For square planar complexes with two different ligands, we can have:
- Cis isomer: Both Cl ligands are adjacent.
- Trans isomer: Cl ligands are opposite each other.
- (Rh(en)3)3+: 0
- (Cr(en)2Br2)+: 2
- (Pt(en)Cl2): 2
Topper's Solved these Questions
- COORDINATION COMPOUNDSBOOK - RESONANCE ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Exercise-2 Part-III: One of More than One Options Correct Type
12 Videos
- COORDINATION COMPOUNDSBOOK - RESONANCE ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Exercise-2 Part-IV: Comprehension -1
5 Videos
- COORDINATION COMPOUNDSBOOK - RESONANCE ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Exercise-2 Part-I: Only one option correct Type
12 Videos
- CHEMISTRY IN EVERYDAY LIFEBOOK - RESONANCE ENGLISHCHAPTER - CHEMISTRY IN EVERYDAY LIFEEXERCISE - ORGANIC CHEMISTRY(Chemistry in every day life)
31 Videos
- D & F BLOCK ELEMENTSBOOK - RESONANCE ENGLISHCHAPTER - D & F BLOCK ELEMENTSEXERCISE - ORGANIC CHEMISTRY(Aldehydes , Ketones, Carboxylic acid)
15 Videos
Similar Questions
Find the number of geometrical isomers in [Co(en)(Pn)(NO2)2]
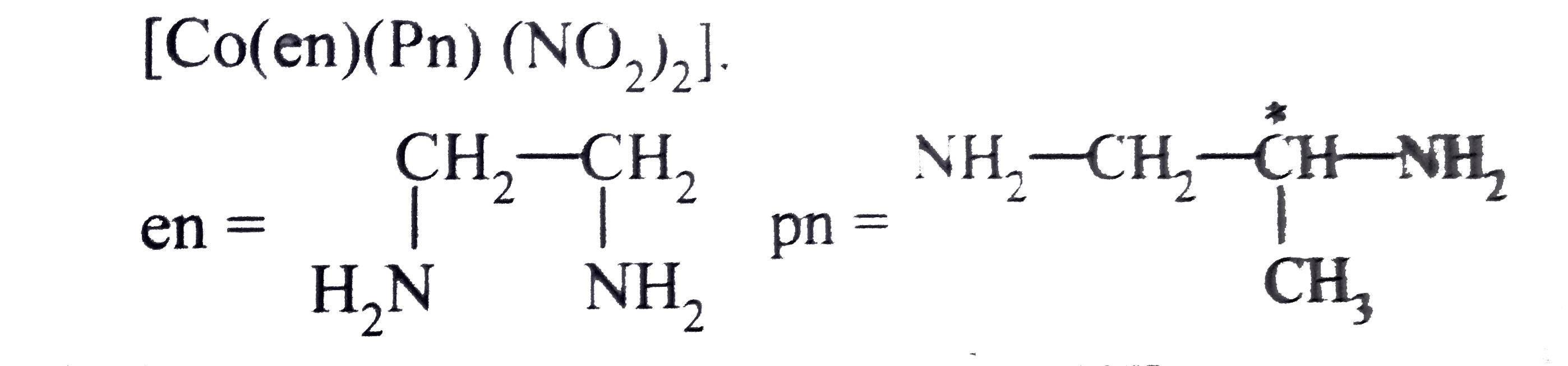 .
.
View SolutionDraw structures of geometrical isomers possible for [Co(en)CI2Br2]Θ .
View SolutionDraw the geometrical isomers of but-2-ene.
View SolutionThe possible geometric isomers of the complex , [Co(en)Br2Cl2]− is :
View SolutionDraw the geometrical isomers of hept−2−en−5−yne ?
View SolutionThe number of geometrical isomers for octahedral [CoCl4(NH3)2]−, square planar [AgBr2Cl2]− and [PtCl2(en)] are:-
View SolutionTotal number of space (stereo) isomers of complex ion [Cr(gly)(en)2]2+ are . . . . . . . .
View SolutionName of the following compounds
(a) [Cr(en)2CI2]CI
(b ) [Pt(Py)4][PtCI4]
View SolutionTotal number of stereoisomers in [Co(en)2Br2]+ complex are
View SolutionThe type of isomerism shown by the complex [CoCl2(en)2] is
View Solution
