Cholesterol, when isolated from natural sources, is obtained as a single enantiomer. The observed rotation α of a 0.3 g sample of cholesterol in 5mL of chloroform solution contained in a 10 cm polarimeter tube is −0.78∘. Calculate the specific rotation of cholesterol. A sample of synthetic cholesterol was prepared consisting entirely of (+)-cholesterol. This synthetic (+)-cholesterol was mixed with some natural (-)-cholesterol. The mixture had a specific rotation [α]20D of −13∘. What fraction of the mixture was (+)-cholesterol ?
To solve the problem step by step, we will first calculate the specific rotation of cholesterol and then determine the fraction of the mixture that was (+)-cholesterol.
Step 1: Calculate the Specific Rotation of Cholesterol
The formula for specific rotation (α) is given by:
(α)=αl⋅c
Where:
- α = observed rotation (in degrees)
- l = path length of the polarimeter tube (in decimeters)
- c = concentration of the solution (in grams per milliliter)
Given Data:
- α=−0.78∘
- l=10cm=1dm
- Sample mass = 0.3 g
- Volume of solution = 5 mL
Calculate Concentration c:
c=mass of samplevolume of solution=0.3g5mL=0.06g/mL
Substitute Values into the Specific Rotation Formula:
(α)=−0.78∘1dm⋅0.06g/mL=−0.780.06=−13∘
Step 2: Calculate the Fraction of (+)-Cholesterol in the Mixture
Now we know that the specific rotation of pure (+)-cholesterol is (−39∘) and the observed rotation of the mixture is (−13∘).
Using the formula for enantiomeric excess:
Observed Rotation=(f+⋅(α)+)+(f−⋅(α)−)f++f−
Where:
- f+ = fraction of (+)-cholesterol
- f− = fraction of (-)-cholesterol
- (α)+ = specific rotation of (+)-cholesterol = +39∘
- (α)− = specific rotation of (-)-cholesterol = −39∘
Since f++f−=1, we can express f− as 1−f+.
Substituting into the equation:
−13=f+⋅39+(1−f+)⋅(−39)
Simplifying the equation:
−13=39f+−39+39f+
−13+39=78f+
26=78f+
f+=2678=13
Conclusion
The fraction of the mixture that was (+)-cholesterol is 13 or approximately 33.33%.
- α = observed rotation (in degrees)
- l = path length of the polarimeter tube (in decimeters)
- c = concentration of the solution (in grams per milliliter)
- α=−0.78∘
- l=10cm=1dm
- Sample mass = 0.3 g
- Volume of solution = 5 mL
c=mass of samplevolume of solution=0.3g5mL=0.06g/mL
(α)=−0.78∘1dm⋅0.06g/mL=−0.780.06=−13∘
- f+ = fraction of (+)-cholesterol
- f− = fraction of (-)-cholesterol
- (α)+ = specific rotation of (+)-cholesterol = +39∘
- (α)− = specific rotation of (-)-cholesterol = −39∘
−13+39=78f+
26=78f+
f+=2678=13
Topper's Solved these Questions
- STEREOISOMERISMBOOK - RESONANCE ENGLISHCHAPTER - STEREOISOMERISMEXERCISE - EXERCISE (PART 1 SECTION (A))
5 Videos
- STEREOISOMERISMBOOK - RESONANCE ENGLISHCHAPTER - STEREOISOMERISMEXERCISE - EXERCISE (PART 1 SECTION (B))
2 Videos
- SOLUTIONSBOOK - RESONANCE ENGLISHCHAPTER - SOLUTIONSEXERCISE - Advabced Level Problems (PART-2)
35 Videos
- STRUCTURAL IDENTIFICATIONBOOK - RESONANCE ENGLISHCHAPTER - STRUCTURAL IDENTIFICATIONEXERCISE - Advanced level Problems (Part-III)
12 Videos
Similar Questions
Knowledge Check
The observed rotation of 2.0 gm of a compound in 10 mL solution in
a 25 cm long polarimeter tube is +13.4∘ . The specific
rotation of compound is :A+30.2∘B−26.8∘C+26.8∘D+40.2∘How many chiral centers are present in cholesterol?
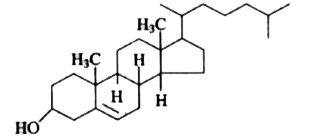 A7B8C9D5
A7B8C9D5
Cholesterol is the precursor of
View SolutionThe observed rotation of 2.0 gm of a compound in 10 mL solution in
a 25 cm long polarimeter tube is +13.4∘ . The specific
rotation of compound is :
View SolutionHigh cholesterol patients are advised to use
View SolutionHigh cholesterol patients are advised to use
View SolutionPure cholesterol has a specific rotation of -32. A sample of cholesterol prepared in the lab has a specific rotation of -8. The enatiomeric excess of the sample of chloresterol is x%. X is :
View SolutionCholesterol is related to which type of molecule.
View Solution
