When 50 g of a nonvolatile solute is dissolved in a certain quantity of solvent, the elevation of boiling point is 2.0 K. What will be the elevation of boiling point when 30 g of solute is dissolved in the same amount of the same solvent?
The correct Answer is:1.2 K
To solve the problem of boiling point elevation when different amounts of a non-volatile solute are dissolved in the same solvent, we can follow these steps:
1. Understanding the Formula: The elevation of boiling point (ΔTb) is given by the formula:
ΔTb=Kb⋅m
where Kb is the ebullioscopic constant of the solvent and m is the molality of the solution.
2. Calculate Molality for 50 g of Solute:
Let's denote the molar mass of the solute as M. The molality (m) can be calculated as:
m=moles of solutemass of solvent in kg
Given that 50 g of solute is dissolved, the number of moles of solute is:
moles=50gM
Assuming we have 1 kg of solvent, the molality when 50 g of solute is used becomes:
m50=50/M1=50M
3. Calculate the Elevation of Boiling Point for 50 g:
Using the boiling point elevation formula:
ΔTb=Kb⋅50M
We know that ΔTb for 50 g is 2.0 K, so we can write:
2.0=Kb⋅50M(1)
4. Calculate Molality for 30 g of Solute:
Now, for 30 g of solute, the number of moles is:
moles=30gM
The molality when 30 g of solute is used is:
m30=30/M1=30M
5. Calculate the Elevation of Boiling Point for 30 g:
Using the same boiling point elevation formula:
ΔTb=Kb⋅30M(2)
6. Relate the Two Elevations:
We can relate the two equations (1) and (2):
ΔTb (for 30 g)ΔTb (for 50 g)=m30m50
Substituting the values:
ΔTb (for 30 g)2.0=30/M50/M
Simplifying gives:
ΔTb (for 30 g)2.0=3050=35
7. Calculate ΔTb for 30 g:
Rearranging gives:
ΔTb (for 30 g)=2.0⋅35=1.2K
Final Answer:
The elevation of boiling point when 30 g of solute is dissolved in the same amount of solvent is 1.2 K.
ΔTb=Kb⋅m
where Kb is the ebullioscopic constant of the solvent and m is the molality of the solution.
Let's denote the molar mass of the solute as M. The molality (m) can be calculated as:
m=moles of solutemass of solvent in kg
Given that 50 g of solute is dissolved, the number of moles of solute is:
moles=50gM
Assuming we have 1 kg of solvent, the molality when 50 g of solute is used becomes:
m50=50/M1=50M
Using the boiling point elevation formula:
ΔTb=Kb⋅50M
We know that ΔTb for 50 g is 2.0 K, so we can write:
2.0=Kb⋅50M(1)
Now, for 30 g of solute, the number of moles is:
moles=30gM
The molality when 30 g of solute is used is:
m30=30/M1=30M
Using the same boiling point elevation formula:
ΔTb=Kb⋅30M(2)
We can relate the two equations (1) and (2):
ΔTb (for 30 g)ΔTb (for 50 g)=m30m50
Substituting the values:
ΔTb (for 30 g)2.0=30/M50/M
Simplifying gives:
ΔTb (for 30 g)2.0=3050=35
Rearranging gives:
ΔTb (for 30 g)=2.0⋅35=1.2K
The elevation of boiling point when 30 g of solute is dissolved in the same amount of solvent is 1.2 K.
Similar Questions
Knowledge Check
When a solute is dissolved in a solvent
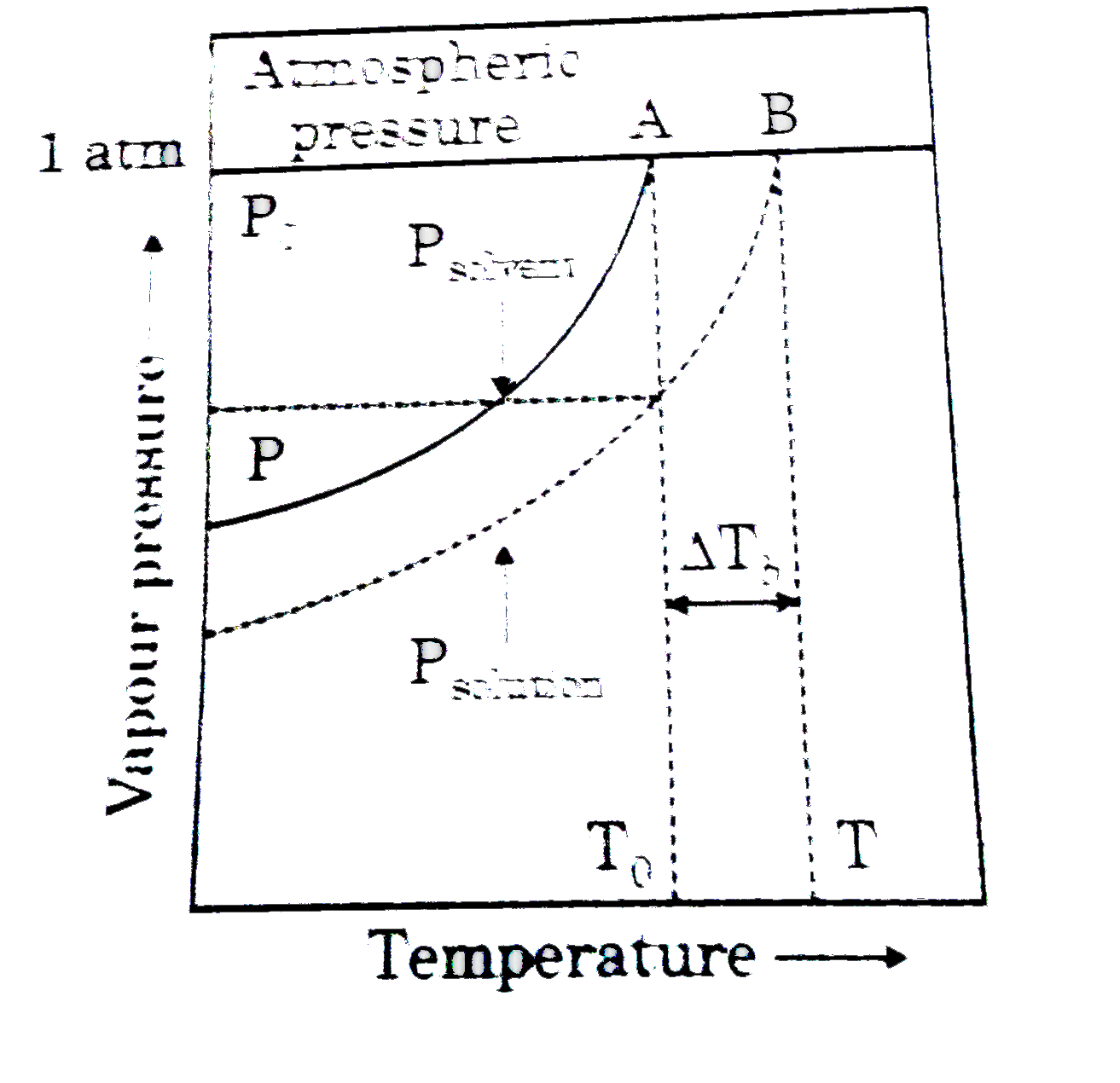
Avapoure pressure of the solvent is increasedBfreezing point of the solution becomes more than that of solventCboiling point of the solution becomes lower thant that of solventDvapour pressure of the solvent is decreasedFigure explains elevation in boiling point when a non-volatile solute is added to a solvent. Variation of vapour pressure with temperaure and elevation in boiling point is marked.
Given that ΔTb is the elevation in boiling point of the solvent in a solution of molarity m then limm→0(ΔTbm) is equal to:AKb (molal elevation constant)BLv (latent heat of vaporisation)CΔS (entropy change)Dx (mole fraction of solute)The elevation in boiling point for one molal solution of a solute in a solvent is called
ACryoscopic constantBBoiling point constantCMolal Ebullioscopic constantDNone
If for the sucrose solution elevation in boiling point is 0.1∘Cthen what will be the boiling point of NaCl solution for same molal concentration
View SolutionIf for a sucrose, elevation in boiling point is 1.0∘C, then what will be the boiling point of NaCl solution for same molal concentration?
View SolutionTo determine the elevation of boiling point more accurately. The solvent area should take
View SolutionIf 1 g of solute (molar mass = 50gmol−1) is dissolved in 50 g of solvent and the elevation in boiling point is 1 K. The molar boiling constant of the solvent is
View SolutionIf the mass of a nonvolatil, nonelectrolyte dissolved in a solvent is doubled but that of solvent is quadrupled, the elevation in boiling point of the solvent will be
View Solution
