Show that the perpendicular bisectors of the sides of a triangle are concurrent.
Step by step video solution for Show that the perpendicular bisectors of the sides of a triangle are concurrent. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- QUESTION BANK 2021Book:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:QUESTION BANK 2021Exercise:LINE AND PLANE
Explore 40 Videos
- QUESTION BANK 2021Book:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:QUESTION BANK 2021Exercise:LINEAR PROGRAMMING PROBLEMS
Explore 30 Videos
- QUESTION BANK 2021Book:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:QUESTION BANK 2021Exercise:PAIRS OF LINES
Explore 21 Videos
- PROBABILITY DISTRIBUTIONBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:PROBABILITY DISTRIBUTIONExercise:MULTIPLE CHOICE QUESTIONS
Explore 9 Videos
- THREE DIMENSIONAL GEOMETRY Book:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:THREE DIMENSIONAL GEOMETRY Exercise:MULTIPLE CHOICE QUESTIONS
Explore 8 Videos
Similar Questions
Knowledge Check
The point at which the perpendicular bisectors of the sides of a triangle intersect is known as ________.
Acircum-centreBorthocenterCcentroidDin-centerThe point at which the perpendicular bisectors of the sides of a triangle intersect is known as ________.
Acircum-centreBorthocentereCcentroidDin-centre
Prove that the internal bisectors of the angles of a triangle are concurrent
View SolutionProve that the perpendicular bisectors of the sides of a cyclic quadrilateral are concurrent.
View SolutionConsider the following statements :
1. The point of intersection of the perpendicular bisectors of the sides of a triangle may lie outside the triangle.
2. The point of intersection of the perpendiculars drawn from the vertices to the opposite sides of a triangle may lie on two sides.
Which of the above statements is/are correct ?
View SolutionThe number of triangles that can be formed by the perpendiculars bisectors of the sides of a triangle is
View SolutionThe equations of the perpendicular bisectors of the sides AB&AC of a triangle ABC are x−y+5=0&x+2y=0. find the equation of the bc
View SolutionA, B and C are three points on a circle. Prove that the perpendicular bisectors of AB, BC and CA are concurrent.
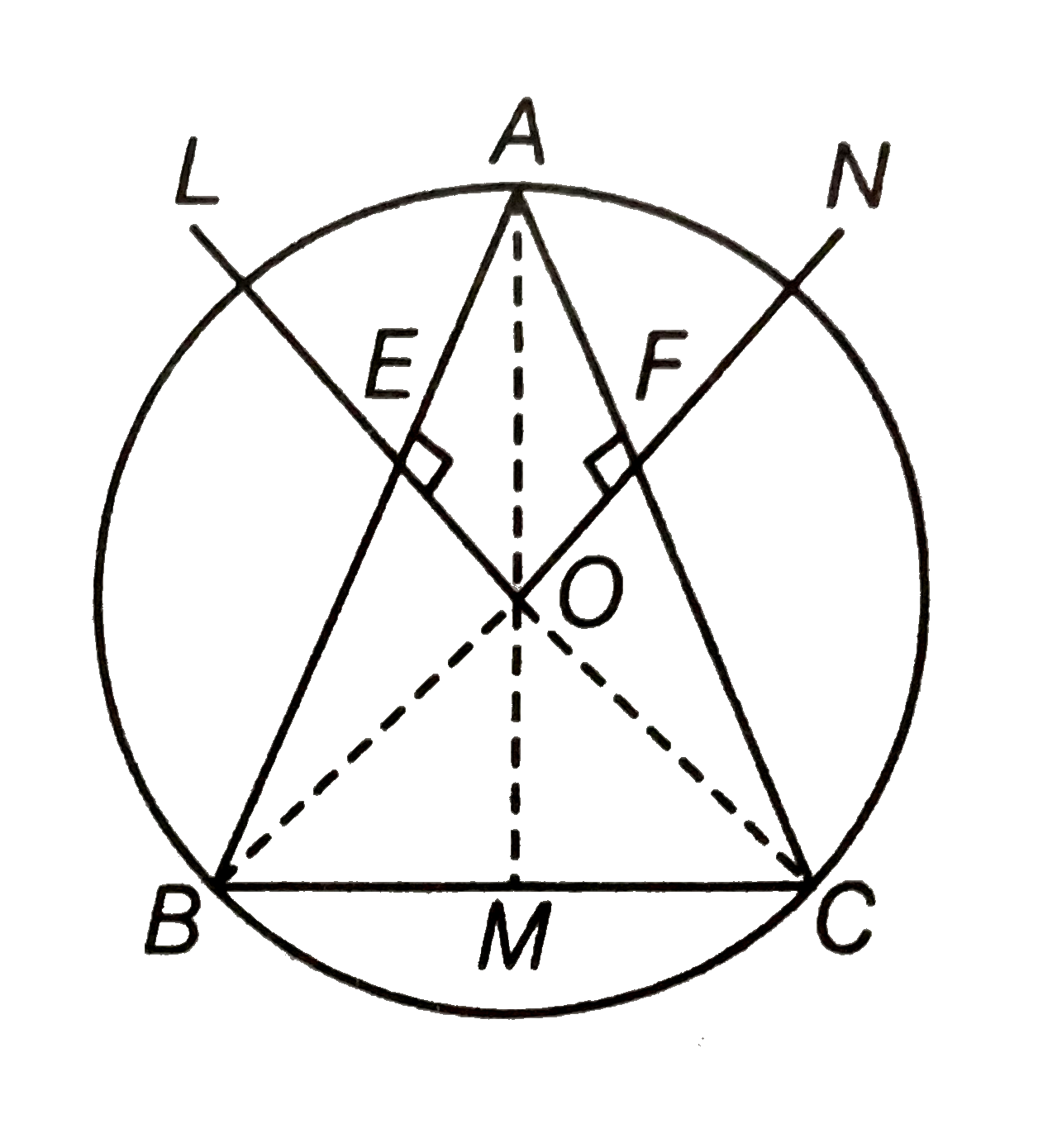
View Solution