For the random variable X , if V(X) = 4 , E(X) = 3 , then E(X2) is …
9
13
12
7
The correct Answer is:B
To find E(X2) given that V(X)=4 and E(X)=3, we can use the relationship between variance, expected value, and the expected value of the square of a random variable. The formula we will use is:
V(X)=E(X2)−(E(X))2
1. Write Down Given Values:
- Variance V(X)=4
- Expected value E(X)=3
2. Substitute into the Variance Formula:
V(X)=E(X2)−(E(X))2
Substituting the known values:
4=E(X2)−(3)2
3. Calculate (E(X))2:
(E(X))2=32=9
4. Rewrite the Equation:
Substitute (E(X))2 back into the equation:
4=E(X2)−9
5. Solve for E(X2):
Rearranging the equation gives:
E(X2)=4+9
E(X2)=13
Final Answer:
E(X2)=13
---
- Variance V(X)=4
- Expected value E(X)=3
V(X)=E(X2)−(E(X))2
Substituting the known values:
4=E(X2)−(3)2
(E(X))2=32=9
Substitute (E(X))2 back into the equation:
4=E(X2)−9
Rearranging the equation gives:
E(X2)=4+9
E(X2)=13
E(X2)=13
Topper's Solved these Questions
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - BINOMIAL DISTRIBUTION
20 Videos
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - DIFFERENTIAL EQUATIONS
38 Videos
- PROBABILITY DISTRIBUTIONBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - MULTIPLE CHOICE QUESTIONS
9 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - MULTIPLE CHOICE QUESTIONS
8 Videos
Similar Questions
Knowledge Check
For a random variable X, if E(X) = 5 and V(X) = 6, then E(X2) is equal to
A19B31C61D11For a random variable X, if Var (X) = 4 and E(X2)=13, the value of E(X) is
A3B4C5D6For a random variable X, if E(X2) = 31 , Var(X) = 6, then E(X)=.........
A2B4C5D25
For a random variable X, E(X)=3andE(X2)=11. Then, variance X is
View SolutionFor a random variable X, E(X)=3 and E(X2)=11 Then, variance of X is
View SolutionIf the probability mass function of a discrete random variable X is
P(x)=Cx3,x=1,2,3
=0, otherwise
Then E(X)=
View SolutionThe probability distribution of a random variable X is given as follows:
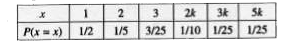
If E(X)=2.3 , then value of k is
View SolutionIf the probability mass function of a discrete random variable X is P(x)=Cx3,x=1,2,3=0, otherwise. Then, E(X) is equal to
View Solution