A rocket of mass M is launched vertically from the surface of the earth with an initial speed . Assuming the radius of the earth to be R and negligible air resistance, the maximum height attained by the rocket above the surface of the earth is where X is:
To solve the problem, we will use the principle of conservation of mechanical energy. The total mechanical energy of the rocket at launch will be equal to the total mechanical energy at the maximum height it reaches.
1. Identify Initial Conditions:
- The rocket is launched from the surface of the Earth with an initial speed V=√gR2.
- The mass of the rocket is M.
- The radius of the Earth is R.
2. Write the Conservation of Energy Equation:
At the launch point (initial state), the total mechanical energy is the sum of kinetic energy (KE) and gravitational potential energy (PE):
Initial Energy=KEi+PEi=12MV2−GMmR
At maximum height h (final state), the velocity is zero, and the potential energy is:
Final Energy=KEf+PEf=0−GMmR+h
3. Set Initial Energy Equal to Final Energy:
12MV2−GMmR=−GMmR+h
4. Substitute the Initial Velocity:
Substitute V=√gR2 into the kinetic energy term:
KEi=12M⎛⎝√gR2⎞⎠2=12M⋅gR2=MgR4
Thus, the equation becomes:
MgR4−GMmR=−GMmR+h
5. Simplify the Equation:
Rearranging gives:
MgR4=GMmR−GMmR+h
Factor out GMm:
MgR4=GMm(1R−1R+h)
6. Combine the Terms:
The right-hand side can be simplified:
1R−1R+h=(R+h)−RR(R+h)=hR(R+h)
Thus, we have:
MgR4=GMm⋅hR(R+h)
7. Cancel M and Rearrange:
Cancel M from both sides (assuming M≠0):
gR4=GhR(R+h)
Rearranging gives:
gR2(R+h)=4Gh
8. Solve for h:
Expanding and rearranging:
gR3+gR2h=4Gh
Rearranging gives:
gR3=h(4G−gR2)
Thus:
h=gR34G−gR2
9. Substituting g=GMR2:
Substitute g back into the equation:
h=gR34GMR2−gR2=gR34GM−gR4R2=gR54GM−gR4
10. Finding X:
The maximum height h can be expressed as h=RX. From the equation, we can deduce that X=3.
Final Answer:
The value of X is 3.
- The rocket is launched from the surface of the Earth with an initial speed V=√gR2.
- The mass of the rocket is M.
- The radius of the Earth is R.
At the launch point (initial state), the total mechanical energy is the sum of kinetic energy (KE) and gravitational potential energy (PE):
Initial Energy=KEi+PEi=12MV2−GMmR
At maximum height h (final state), the velocity is zero, and the potential energy is:
Final Energy=KEf+PEf=0−GMmR+h
12MV2−GMmR=−GMmR+h
Substitute V=√gR2 into the kinetic energy term:
KEi=12M⎛⎝√gR2⎞⎠2=12M⋅gR2=MgR4
Thus, the equation becomes:
MgR4−GMmR=−GMmR+h
Rearranging gives:
MgR4=GMmR−GMmR+h
Factor out GMm:
MgR4=GMm(1R−1R+h)
The right-hand side can be simplified:
1R−1R+h=(R+h)−RR(R+h)=hR(R+h)
Thus, we have:
MgR4=GMm⋅hR(R+h)
Cancel M from both sides (assuming M≠0):
gR4=GhR(R+h)
Rearranging gives:
gR2(R+h)=4Gh
Expanding and rearranging:
gR3+gR2h=4Gh
Rearranging gives:
gR3=h(4G−gR2)
Thus:
h=gR34G−gR2
Substitute g back into the equation:
h=gR34GMR2−gR2=gR34GM−gR4R2=gR54GM−gR4
The maximum height h can be expressed as h=RX. From the equation, we can deduce that X=3.
The value of X is 3.
Topper's Solved these Questions
- JEE MAIN REVISION TEST - 26 (2020)BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST - 26 (2020)EXERCISE - PHYSICS (SECTION 2)
5 Videos
- JEE MAIN REVISION TEST - 25 | JEE - 2020BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST - 25 | JEE - 2020EXERCISE - PHYSICS
25 Videos
- JEE MAIN REVISION TEST - 27 - JEE -2020BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST - 27 - JEE -2020EXERCISE - PHYSICS (SECTION 2)
5 Videos
Similar Questions
A rocket is launched vertical from the surface of the earth of radius R with an initial speed v. If atmospheric resistance is neglected, then maximum height attained by the rocket is
View SolutionA body is projected vertically upwards from the surface of the earth with a velocity equal to half of escape velocity of the earth. If R is radius of the earth, maximum height attained by the body from the surface of the earth is
View Solution
Knowledge Check
A rocket is launched vertically from the surface of earth with an initial velocity v. How far above the surface of earth it will go? Neglect the air resistance.
AREv2gRE−v2BREv2gRE+v2CREv22gRE−v2DREv22gRE+v2
A body is thrown from the surface of the earth with velocity (gRe)/12, where Re is the radius of the earth at some angle from the vertical. If the maximum height reached by the body is Re/4, then the angle of projection with the vertical is
View SolutionAssume that a frictionless tunnel is made in the earth along its diameter, a particle in projected in the tunnel from the surface of the earth with an initial speed v0=√gR, where g is the acceleration due to gravity on the earth's surface & R is the radius of the earth, if time taken by the particle to reach the centre of the earth is πn√4Rg the value of n is?
View SolutionA body is projected vertically upwards from the surface of earth with a velocity equal to half the escape velocity. If R be the radius of earth, maximum height attained by the body from the surface of earth is Rn. Find the value of n.
View SolutionA particle is projected vertically upwards the surface of the earth (radius Re) with a speed equal to one fourth of escape velocity what is the maximum height attained by it from the surface of the earth?
View SolutionA rocket is launched upward from the earth surface whose velocity time graph shown in figure. Then maximum height attained by the rocket is :
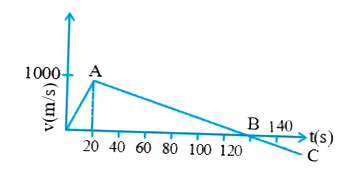
View SolutionA particle is projected vertically with speed V from the surface of the earth . Maximum height attained by the particle , in term of the radius of earth R,V and g is ( V < escape velocity , g is the acceleration due to gravity on the surface of the earth )
View SolutionIf a body of mass m is raised to height 2 R from the earths surface, then the change in potential energy of the body is (R is the radius of earth)
View Solution
