AB is a vertical pole with B at the ground level and and A at the top .A man finds that the angle of elevation of the point A from a certain point C on the ground is 60∘ . He moves away from the pole along the line BC to a point D such that CD = 7 m .From D . the angle of elevation of the point A is 45∘ .Find the height of the pole .
7√321√3+1m
7√321√3−1m
7√32(√3+1)m
7√32(√3−1)m
The correct Answer is:C
To find the height of the pole AB, we will follow these steps:
Step 1: Set Up the Problem
Let the height of the pole AB be H. The point C is where the angle of elevation to point A is 60∘, and the point D is where the angle of elevation to point A is 45∘. The distance CD is given as 7 m.
Step 2: Use Triangle ABC
From point C, we can use the tangent of the angle of elevation:
tan(60∘)=HBC
Since tan(60∘)=√3, we have:
√3=HBC
This gives us our first equation:
H=BC⋅√3(1)
Step 3: Use Triangle ABD
From point D, we can use the tangent of the angle of elevation:
tan(45∘)=HBD
Since tan(45∘)=1, we have:
1=HBD
This gives us:
H=BD(2)
Now, we know that BD=BC+CD, and since CD=7 m, we can write:
BD=BC+7(3)
Step 4: Substitute Equation (3) into Equation (2)
From equation (2):
H=BC+7
Now we can substitute equation (1) into this:
BC⋅√3=BC+7
Step 5: Rearrange the Equation
Rearranging gives:
BC⋅√3−BC=7
Factoring out BC:
BC(√3−1)=7
Thus, we can solve for BC:
BC=7√3−1(4)
Step 6: Substitute Back to Find H
Now substitute equation (4) back into equation (1):
H=(7√3−1)⋅√3
This simplifies to:
H=7√3√3−1
Step 7: Rationalize the Denominator
To rationalize the denominator:
H=7√3(√3+1)(√3−1)(√3+1)=7√3(√3+1)3−1=7√3(√3+1)2
Final Answer
Thus, the height of the pole H is:
H=7√3(√3+1)2
---
Let the height of the pole AB be H. The point C is where the angle of elevation to point A is 60∘, and the point D is where the angle of elevation to point A is 45∘. The distance CD is given as 7 m.
From point C, we can use the tangent of the angle of elevation:
tan(60∘)=HBC
Since tan(60∘)=√3, we have:
√3=HBC
This gives us our first equation:
H=BC⋅√3(1)
From point D, we can use the tangent of the angle of elevation:
tan(45∘)=HBD
Since tan(45∘)=1, we have:
1=HBD
This gives us:
H=BD(2)
Now, we know that BD=BC+CD, and since CD=7 m, we can write:
BD=BC+7(3)
From equation (2):
H=BC+7
Now we can substitute equation (1) into this:
BC⋅√3=BC+7
Rearranging gives:
BC⋅√3−BC=7
Factoring out BC:
BC(√3−1)=7
Thus, we can solve for BC:
BC=7√3−1(4)
Now substitute equation (4) back into equation (1):
H=(7√3−1)⋅√3
This simplifies to:
H=7√3√3−1
To rationalize the denominator:
H=7√3(√3+1)(√3−1)(√3+1)=7√3(√3+1)3−1=7√3(√3+1)2
Thus, the height of the pole H is:
H=7√3(√3+1)2
Topper's Solved these Questions
- PROPERTIES OF TRIANGLEBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF TRIANGLEEXERCISE - JEE Advanced (Archive)
50 Videos
- PROPERTIES OF TRIANGLEBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF TRIANGLEEXERCISE - Level - 2
55 Videos
- PROBABILITYBOOK - VMC MODULES ENGLISHCHAPTER - PROBABILITYEXERCISE - JEE ADVANCED (ARCHIVE)
102 Videos
- QUADRATIC EQUATIONS & INEQUATIONS BOOK - VMC MODULES ENGLISHCHAPTER - QUADRATIC EQUATIONS & INEQUATIONS EXERCISE - JEE Advance ( Archive )
20 Videos
Similar Questions
AB is a vertical pole with B at the ground level and A at the top. A man finds that the angle of elevation of the point A from a certain point C on the ground is 60o . He moves away from the pole along the line BC to a point D such that CD=7m . From D the angle of elevation of the point A is 45o . Then the height of the pole is (1) 7√321√3−1m (2) 7√32√3+1m (3) 7√32√3−1m (4) 7√32√3+1m
View SolutionThe angle of elevation of the top of a tower from a point on the ground is 60∘. which is 25 m away from the foot of the tower. Find the height of the tower
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower is 30∘ . Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.

View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionIn figure , the angle of elevation of the top of a tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m, Find the distance of the point from the foot of the power .
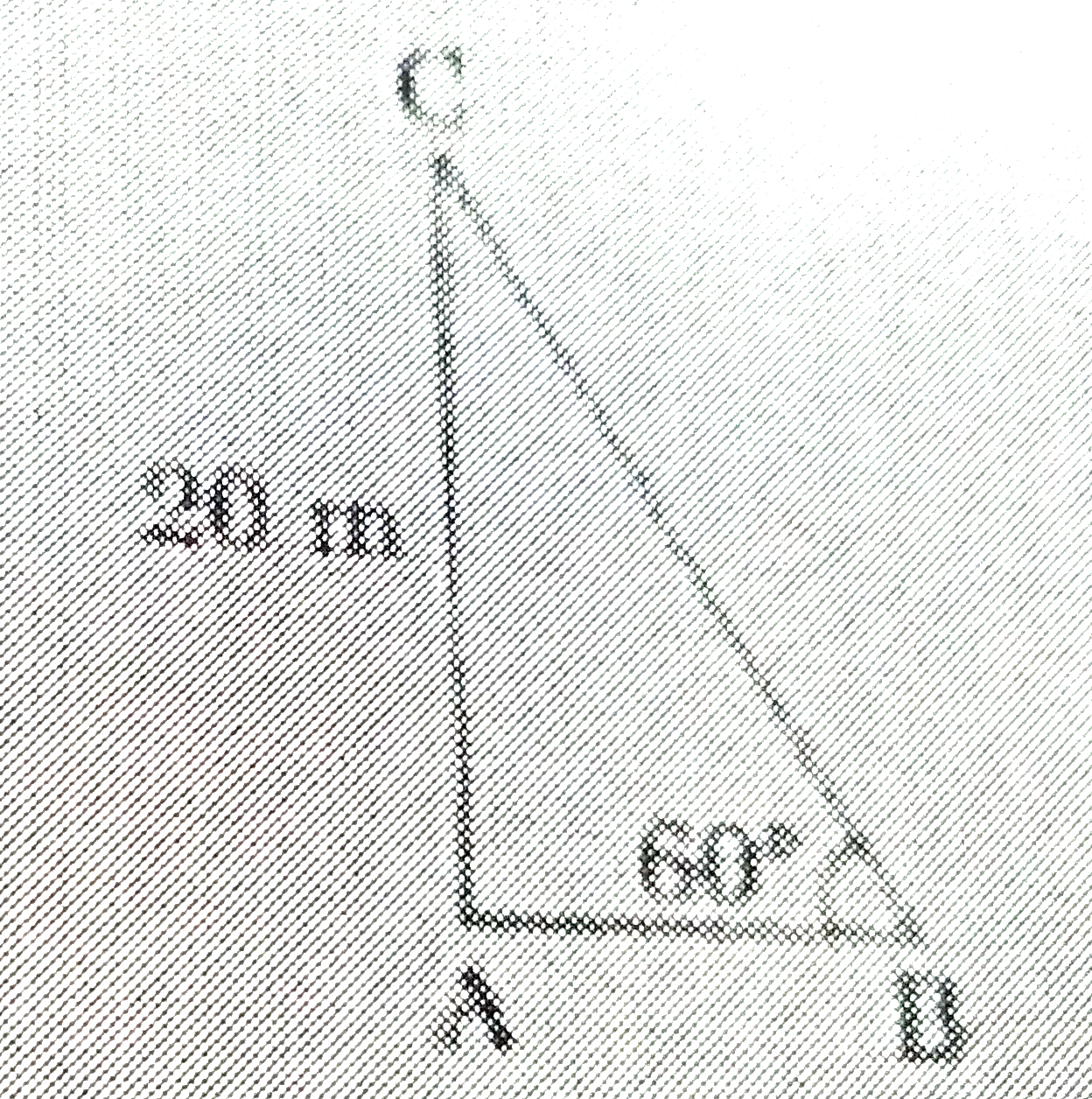
View Solution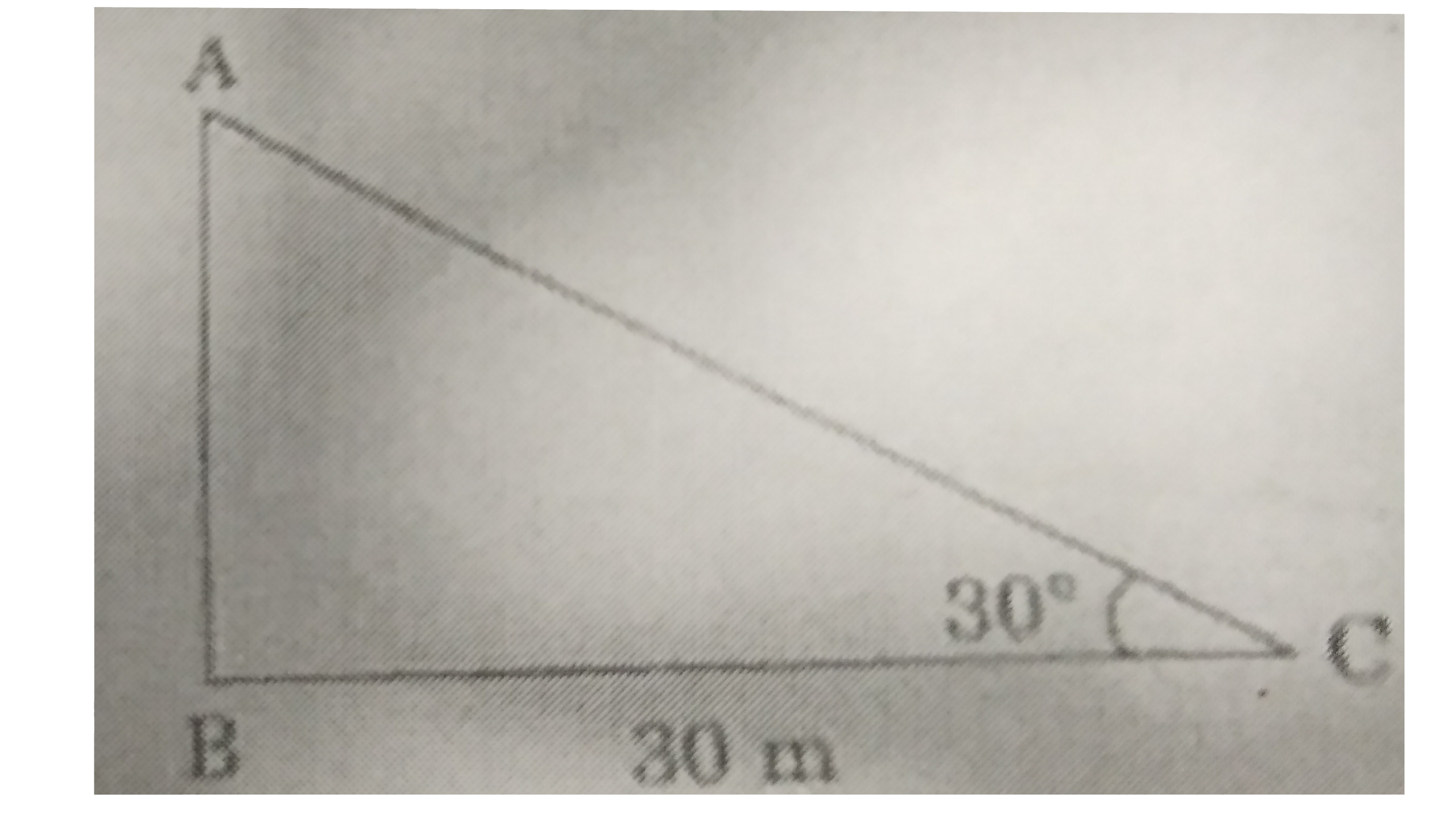
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point 40 m away from its foot is 60∘. Find the height of the tower.
View Solution
