Let z=x+iy be a complex number where x and y are integers. Then ther area of the rectangle whose vertices are the roots of the equaiton ¯zz3+z¯z3=350.
48
32
40
80
The correct Answer is:A
Step by step video & image solution for Let z = x + iy be a complex number where x and y are integers. Then ther area of the rectangle whose vertices are the roots of the equaiton barz z^(3) + zbarz^(3) = 350. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- COMPLEX NUMBERS BOOK - VMC MODULES ENGLISHCHAPTER - COMPLEX NUMBERS EXERCISE - NUMRICAL VALUE TYPE FOR JEE MAIN
14 Videos
- CIRCLESBOOK - VMC MODULES ENGLISHCHAPTER - CIRCLESEXERCISE - JEE ADVANCED ( ARCHIVE )
68 Videos
- CONIC SECTIONSBOOK - VMC MODULES ENGLISHCHAPTER - CONIC SECTIONSEXERCISE - JEE ADVANCED ARCHIVE
76 Videos
Similar Questions
Let z=x+iy be a complex number where xandy are integers. Then, the area of the rectangle whose vertices are the roots of the equation zz¯ ^3+zbar z^3=350 is (a)48 (b) 32 (c) 40 (d) 80
View SolutionLet z=x+iy be a complex number where xandy are integers. Then, the area of the rectangle whose vertices are the roots of the equation zz3+zz3=350 is 48 (b) 32 (c) 40 (d) 80
View SolutionIf z is a complex number, then the area of the triangle (in sq. units) whose vertices are the roots of the equation z3+iz2+2i=0 is equal to (where, i2=−1)
View SolutionThe number of solutions of the equation z3+¯z=0, is
View SolutionFind the circumstance of the triangle whose vertices are given by the complex numbers z1,z2 and z3.
View SolutionIf z is a complex number such that |z|=2, then the area (in sq. units) of the triangle whose vertices are given by z,−izandiz−z is equal to
View SolutionFind the complex number z if z¯z=2andz+¯z=2
View SolutionFour particles, each with mass m are arranged symmetrically about the origin on the x axis. A fifth particle, with mass M, is on the y axis. The direction of the gravitational force on M is:
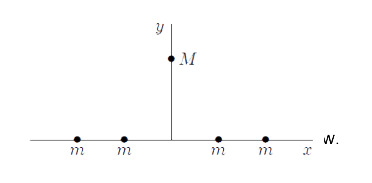
View SolutionIf z=x+iy is any complex number and |z−1|=|z+1| then show that |z|=y.
View SolutionNumber of complex numbers satisfying z3=¯z is
View Solution
