A discrete random variable X has the probability distribution as given below
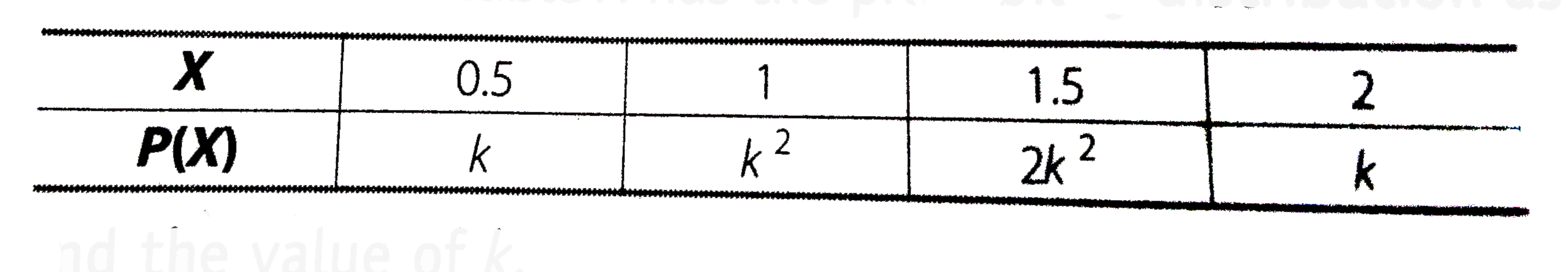
(i) Find the value of k.
(ii) Determine the mean of the distribution.
The correct Answer is:(i)13(ii)2318
Step by step video & image solution for A discrete random variable X has the probability distribution as given below (i) Find the value of k. (ii) Determine the mean of the distribution. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROBABILITYBOOK - VMC MODULES ENGLISHCHAPTER - PROBABILITYEXERCISE - LEVEL - 1
95 Videos
- PROBABILITYBOOK - VMC MODULES ENGLISHCHAPTER - PROBABILITYEXERCISE - LEVEL - 2
49 Videos
- PERMUTATION & COMBINATIONBOOK - VMC MODULES ENGLISHCHAPTER - PERMUTATION & COMBINATIONEXERCISE - JEE ARCHIVE
50 Videos
- PROPERTIES OF TRIANGLEBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF TRIANGLEEXERCISE - JEE Advanced (Archive)
50 Videos
Similar Questions
A random variable X has the following distribution

View SolutionA discrete random variable X has the probability distribution given below: X:0.511.52 P(X):kk22k2k Find the value of k. (ii) Determine the mean of the distribution.
View SolutionA random variable X has the following probability distribution :

determine the value of a
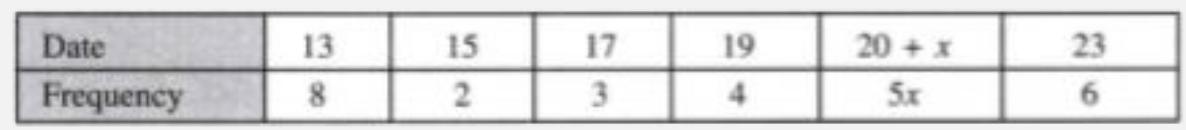
View SolutionFind the value of x, if the mean of the following distribution is 18.
View SolutionA random variable 'X' has the following probability distribution :

The values of k is
View Solution
is the probability distribution of a random variable X. Find the variance of X.
View Solution
is the probability distribution of a random variable X. Find the variance of X.
View SolutionFind the probability distribution of the maximum of two scores obtained when a die is thrown twice. Determine also the mean of the distribution.
View SolutionFind the probability distribution of the maximum of two scores obtained when a die is thrown twice. Determine also the mean of the distribution.
View SolutionA random variable X has the following distribution .

The value of k and P(X<4) are equal to
View Solution
