A committee of five is to be chosen from a group of 9 people. The probability that a certain married couple will either serve together or not at all is
1/2
5/9
4/9
2/3
The correct Answer is:C
To solve the problem of finding the probability that a certain married couple will either serve together or not at all in a committee of five chosen from a group of 9 people, we can follow these steps:
Step 1: Understand the Total Number of Ways to Choose the Committee
First, we need to determine the total number of ways to choose a committee of 5 from 9 people. This can be calculated using the combination formula:
Total ways=(nr)=n!r!(n−r)!
where n is the total number of people and r is the number of people to choose.
For our case:
Total ways=(95)=9!5!(9−5)!=9!5!4!=126
Step 2: Calculate the Number of Favorable Outcomes
Next, we need to calculate the number of favorable outcomes where the married couple either serves together or not at all.
Case 1: The Couple Serves Together
If the couple serves together, we can treat them as a single unit or "block." This means we now have 8 units to choose from (the couple as one unit and the other 7 individuals).
We need to choose 4 more members from the remaining 7 people:
Ways when couple serves together=(73)=7!3!(7−3)!=35
Case 2: The Couple Does Not Serve at All
If the couple does not serve at all, we need to choose all 5 members from the remaining 7 people:
Ways when couple does not serve=(75)=7!5!(7−5)!=21
Step 3: Combine the Favorable Outcomes
Now, we can combine the two cases to find the total number of favorable outcomes:
Total favorable outcomes=Ways when couple serves together+Ways when couple does not serve=35+21=56
Step 4: Calculate the Probability
Finally, we can calculate the probability that the couple will either serve together or not at all:
Probability=Total favorable outcomesTotal ways=56126=49
Final Answer
The probability that the married couple will either serve together or not at all is 49.
---
First, we need to determine the total number of ways to choose a committee of 5 from 9 people. This can be calculated using the combination formula:
Total ways=(95)=9!5!(9−5)!=9!5!4!=126
Next, we need to calculate the number of favorable outcomes where the married couple either serves together or not at all.
If the couple serves together, we can treat them as a single unit or "block." This means we now have 8 units to choose from (the couple as one unit and the other 7 individuals).
Ways when couple serves together=(73)=7!3!(7−3)!=35
If the couple does not serve at all, we need to choose all 5 members from the remaining 7 people:
Ways when couple does not serve=(75)=7!5!(7−5)!=21
Now, we can combine the two cases to find the total number of favorable outcomes:
Total favorable outcomes=Ways when couple serves together+Ways when couple does not serve=35+21=56
Finally, we can calculate the probability that the couple will either serve together or not at all:
Probability=Total favorable outcomesTotal ways=56126=49
The probability that the married couple will either serve together or not at all is 49.
Topper's Solved these Questions
- PROBABILITYBOOK - VMC MODULES ENGLISHCHAPTER - PROBABILITYEXERCISE - LEVEL - 2
49 Videos
- PROBABILITYBOOK - VMC MODULES ENGLISHCHAPTER - PROBABILITYEXERCISE - NUMERICAL VALUE TYPE FOR JEE MAIN
15 Videos
- PROBABILITYBOOK - VMC MODULES ENGLISHCHAPTER - PROBABILITYEXERCISE - JEE ADVANCED (ARCHIVE)
102 Videos
- PERMUTATION & COMBINATIONBOOK - VMC MODULES ENGLISHCHAPTER - PERMUTATION & COMBINATIONEXERCISE - JEE ARCHIVE
50 Videos
- PROPERTIES OF TRIANGLEBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF TRIANGLEEXERCISE - JEE Advanced (Archive)
50 Videos
Similar Questions
A committee of 5 is to be formed from a group of 10 people consisting 4 single men 4 single women and a married couple. The committee is to consist of a chairman , who must be a single man 2, other men and 2 women,
How many of these would include the married couples?
View SolutionA committee of 5 is to be formed from a group of 10 people consisting 4 single men 4 single women and a married couple. The committee is to consist of a chairman , who must be a single man 2, other men and 2 women,
Find the total number of committes possible.
View SolutionA committee of 4 persons has to be chosen from 8 boys and 6 girls, consisting of at least one girl. Find the probability that the committee consists of more girls than boys.
View SolutionA committtee of three people is to be chosen from 4 married couples. The number of committees that can be made such that it consists one woman and two men except that a husbnd and wife both cannot serve on the committee is (A) 2 (B) 4 (C) 8 (D) 12
View Solution4 people are selected randomly out of six married couple. Find the probability that exactly one married couple is formed exactly two married couple are formed the do not form a married couple.
View SolutionA committee of three persons is to be randomly selected from a group of three men and two women and the chair person will be randomly selected from 2 woman and 1 men for the committee. The probability that the committee will have exactly two woman and one man and that the chair person will be a woman, is
View SolutionConsider a twon with n people. A person spreads a rumour to a second, who in turn repeats it to a third and so on. Supppose that at each stage, the recipient of the rumour is chosen at random from the remaining (n-1) people. The probability that the rumour will be repeated n times without being repeated to the originator is
View SolutionFrom the employees of a company, 5 persons are selected to represent them in the managing committee of the company. Particulars of five persons are as follows:
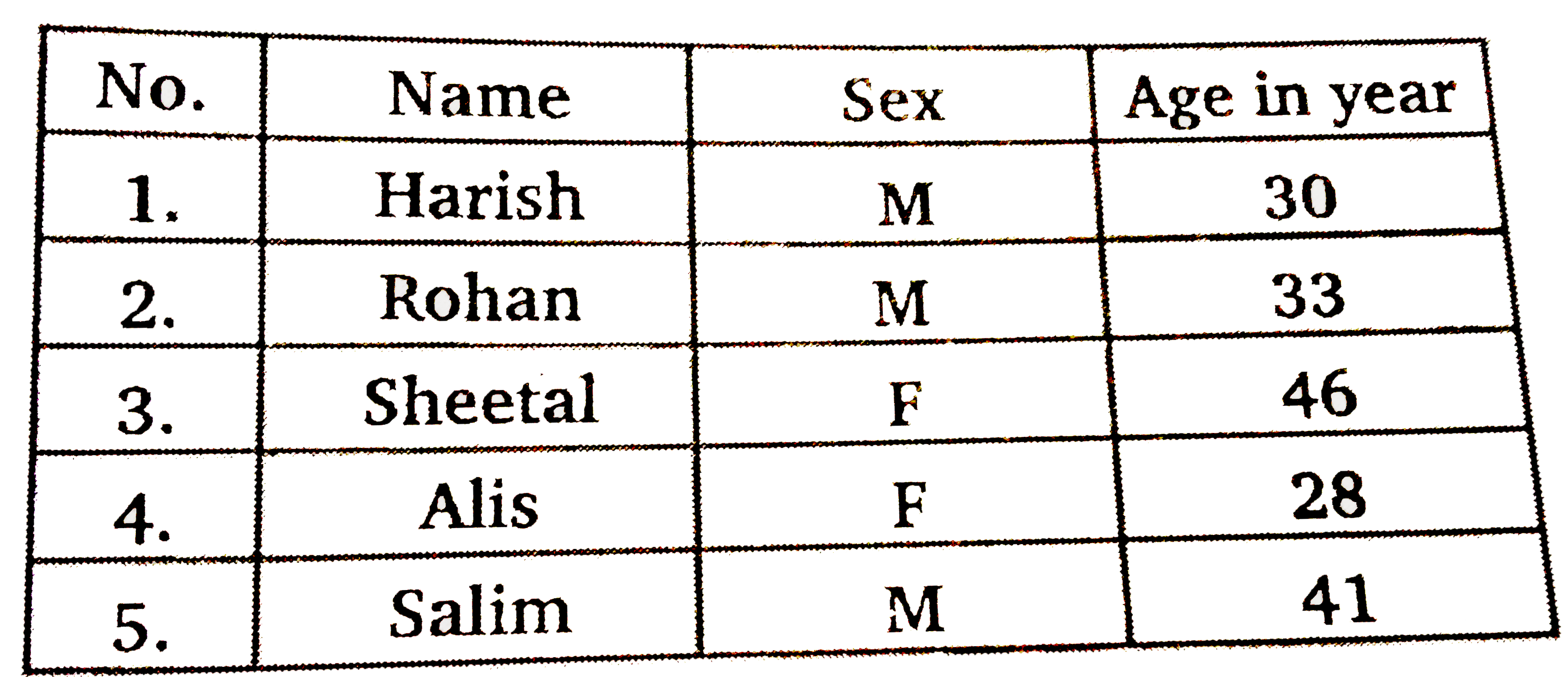
A person is selected at random from this group to act as a spokeperson. What is the probability that the spokesperson wil be either male or over 35 years?
View SolutionFrom the employees of a company 5 persons are elected to represent them n the managing committee of the company. Particulars of the five persons are as follows: S. No. Person Age (in years) 1 2 3 4 5 Male Male Female Female Male 30 33 46 28 41 A person is selected at random from this group to act as a spokesperson. What is the probability that the spokesperson will be either male or over 35 years?
View SolutionFrom the employees of a company, 5 persons are selected to represent them in the managing committee of the company. Particulars of five persons are as follows:S.No. Name Sex Age in years1. Harish M 302. Rohan M 333. Sheetal F 464. Alis F 285. Salim M 41A person is selected at random from this group to act as a spokesperson. What is the probability that the spokesperson will be either male or over 35 years?
View Solution
