A spring balance is attached to the ceiling of a lift. A man hangs his bag on the spring and the spring reads 49N, when the lift is stationary. If the lift moves downward with an acceleration of 5m/22, the reading of the spring balance will be
49 N
24 N
74 N
15 N
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Understand the initial condition
When the lift is stationary, the reading of the spring balance is equal to the weight of the bag. The weight (W) of the bag can be expressed as:
W=mg
where m is the mass of the bag and g is the acceleration due to gravity (approximately 9.8m/s2).
Step 2: Calculate the mass of the bag
Given that the spring balance reads 49N when the lift is stationary, we can set up the equation:
mg=49N
Now, we can solve for m:
m=49g=499.8
Calculating this gives:
m=5kg
Step 3: Analyze the situation when the lift is moving downward
When the lift is moving downward with an acceleration a=5m/s2, the apparent weight (R') of the bag can be calculated using the formula:
R′=mg−ma
Here, mg is the weight of the bag and ma is the force due to the acceleration of the lift.
Step 4: Substitute the known values
We already calculated m=5kg. Now substituting the values into the equation:
R′=mg−ma
R′=(5kg⋅9.8m/s2)−(5kg⋅5m/s2)
Calculating mg:
mg=49N
Calculating ma:
ma=25N
Now substituting these values back into the equation:
R′=49N−25N
R′=24N
Step 5: Conclusion
The reading of the spring balance when the lift is moving downward with an acceleration of 5m/s2 is 24N.
---
When the lift is stationary, the reading of the spring balance is equal to the weight of the bag. The weight (W) of the bag can be expressed as:
W=mg
where m is the mass of the bag and g is the acceleration due to gravity (approximately 9.8m/s2).
Given that the spring balance reads 49N when the lift is stationary, we can set up the equation:
mg=49N
Now, we can solve for m:
m=49g=499.8
Calculating this gives:
m=5kg
When the lift is moving downward with an acceleration a=5m/s2, the apparent weight (R') of the bag can be calculated using the formula:
R′=mg−ma
Here, mg is the weight of the bag and ma is the force due to the acceleration of the lift.
We already calculated m=5kg. Now substituting the values into the equation:
R′=mg−ma
R′=(5kg⋅9.8m/s2)−(5kg⋅5m/s2)
Calculating mg:
mg=49N
Calculating ma:
ma=25N
Now substituting these values back into the equation:
R′=49N−25N
R′=24N
The reading of the spring balance when the lift is moving downward with an acceleration of 5m/s2 is 24N.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - DYNAMICS OF A PARTICLEEXERCISE - JEE Advance (Archive) Level - II (SINGLE OPTION CORRECT TYPE )31videos
DYNAMICS OF A PARTICLE
- BOOK - VMC MODULES ENGLISHCHAPTER - DYNAMICS OF A PARTICLEEXERCISE - LEVEL 2 ( NUMERICAL TYPE FOR JEE MAIN )16videos
DYNAMICS OF A PARTICLE
- BOOK - VMC MODULES ENGLISHCHAPTER - DC CIRCUITEXERCISE - JEE ADVANCED ARCHIVE68videos
DC CIRCUIT
- BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - IN-CHAPTER EXERCISE-G10videos
ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENT
Similar Questions
The reading in the spring balance is
 View Solution
View SolutionThe reading in the spring balance is:
 View Solution
View SolutionWhat is the reading of the spring balance in the following device?
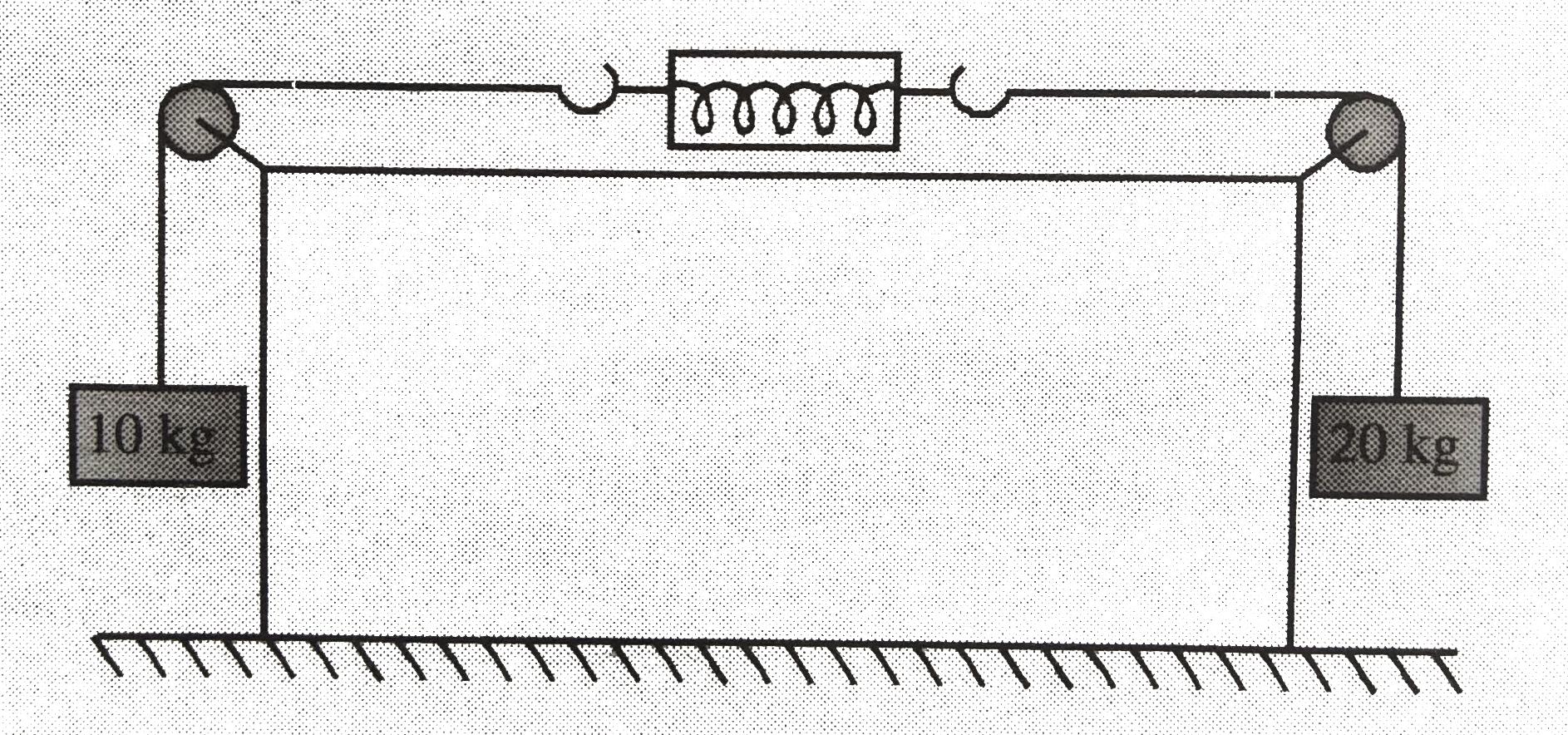 View Solution
View SolutionFor the arrangement shown in the figure, the reading of spring balance is
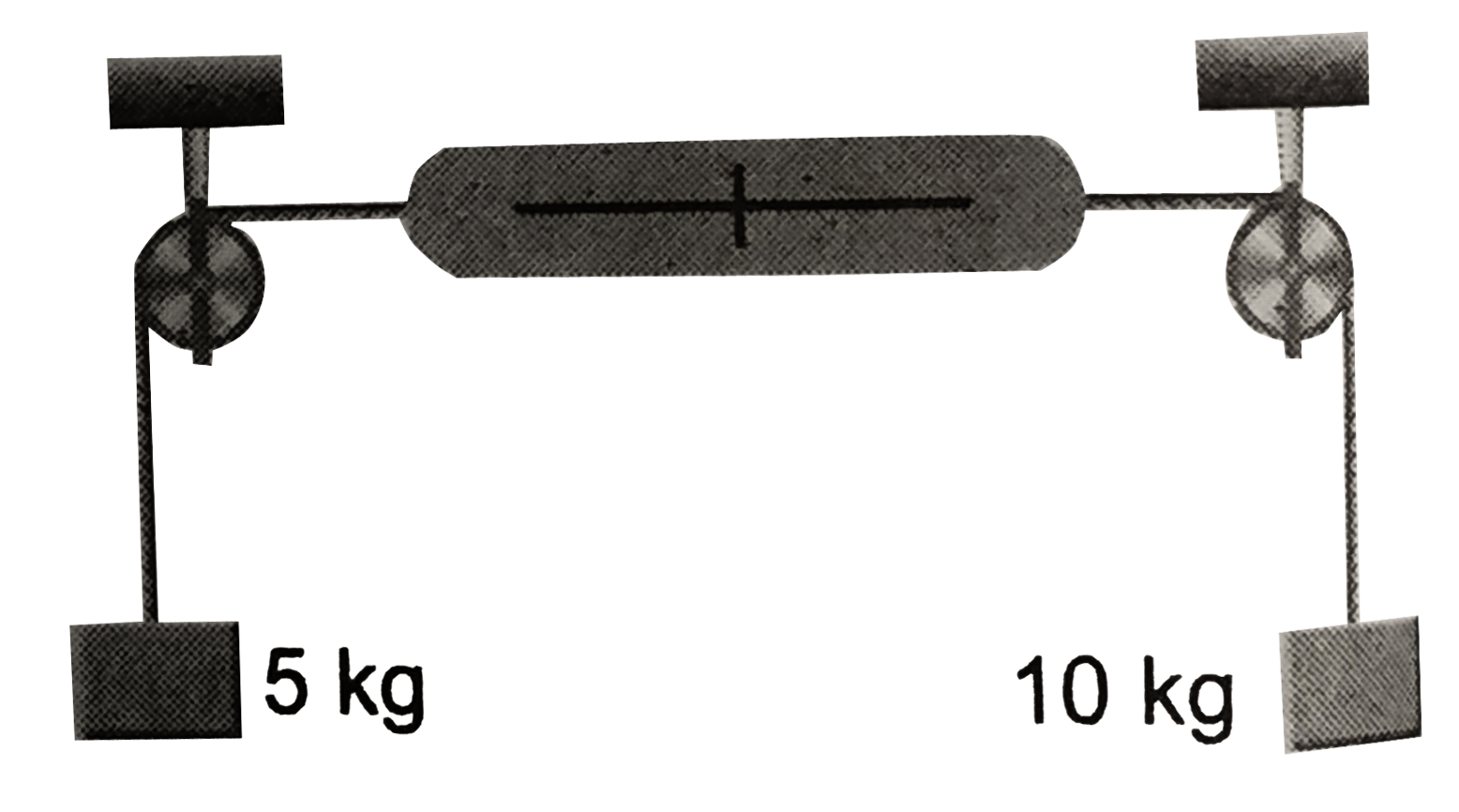 View Solution
View SolutionA man of mass 70 kg stands on a weighing scale in a lift which is moving downward with the uniform acceleration of 5ms2 . The reading on the weighing scale is
View SolutionA body of mass 50 kg is suspended using a spring balance inside a lift at rest. If the lift startts falling freely, the reading of the spring balance is
View SolutionTime period of a spring pendulum when lift moves downward with constant velocity v is T second. When the lift moves upward with constant acceleration=g3 , the time period will be
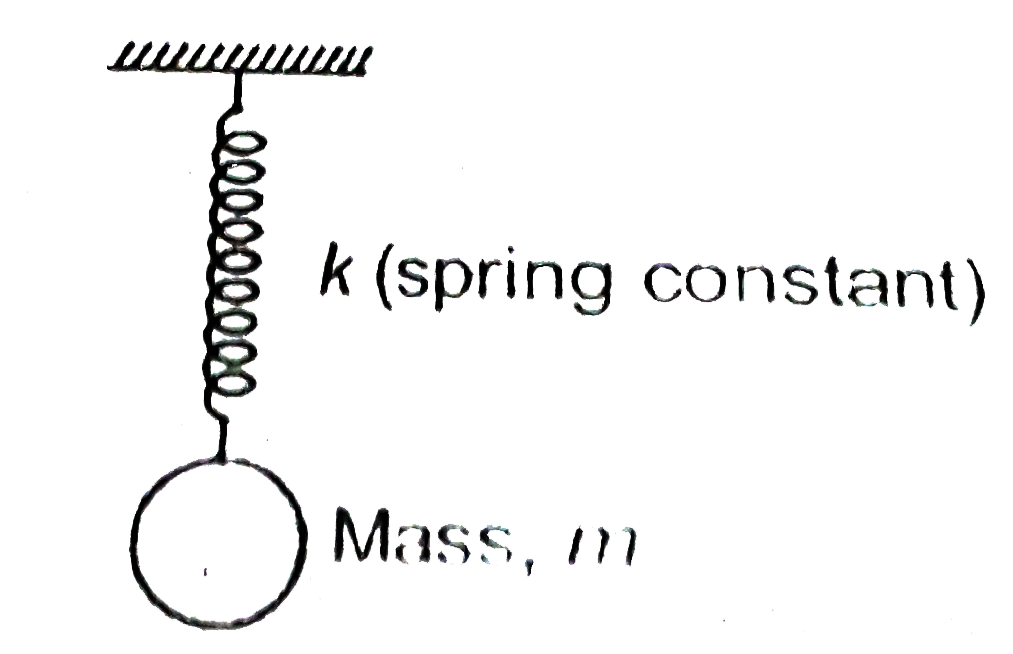 View Solution
View SolutionFind the reading of the massless spring balance in the given condition
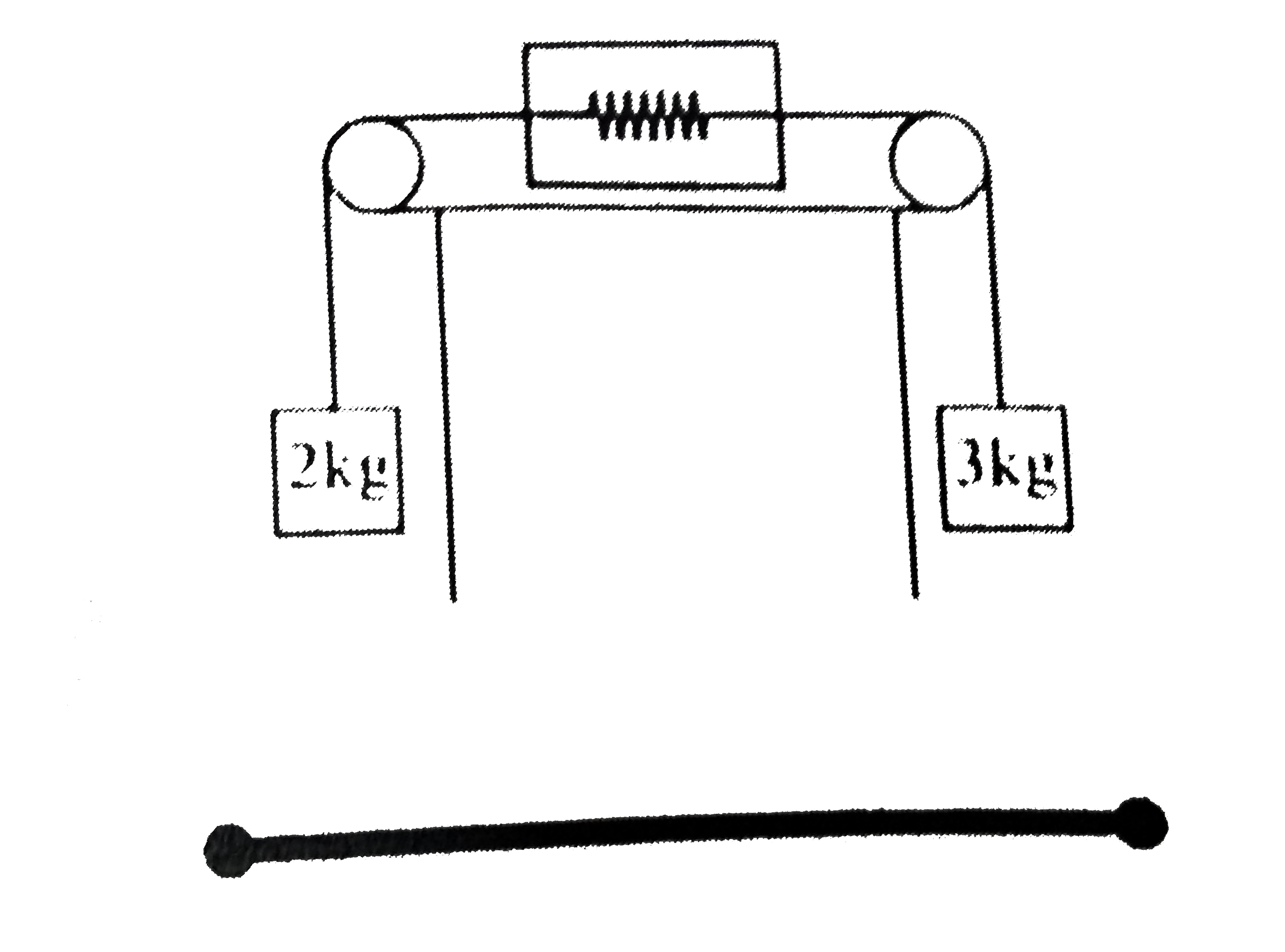 View Solution
View SolutionA 5 kg mass attached to a spring scale rests on a frictionless , horizontal surface as in figure . The spring scale, attached to the front end of a box car, reads 18 N when the car is in motion .
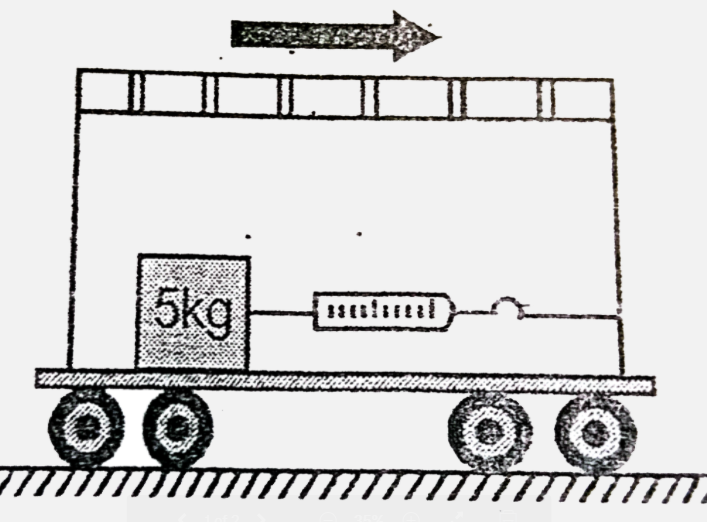
(a) If the spring reads zero when the car is at rest , determine acceleration of the car while it is in motion .
(b) What will the spring scale read if the car moves with constant velocity ?
(c ) Describe the forces on the mass as observed by someone in the car and by someone at rest outside the car.View SolutionThe mass of man when standing on the lift is 60 kg. The weight when the lift is moving upwards with acceration 4.9ms−2 is
View Solution

