If CpandCv denoted the specific heats of unit mass of nitrogen at constant pressure and volume respectively, then
Cp−CV=R/28
Cp−Cv==R/14
Cp−Cv=R
Cp−Cv=28R
The correct Answer is:A
To solve the problem regarding the specific heats of nitrogen at constant pressure and volume, we follow these steps:
1. Understanding the Relationship:
The relationship between the specific heats at constant pressure (Cp) and at constant volume (Cv) for an ideal gas is given by the equation:
Cp−Cv=R
where R is the universal gas constant.
2. Converting Units:
The problem states that we need to express the specific heats in terms of Joules per kilogram Kelvin (J/kg·K). The gas constant R is typically given in Joules per mole Kelvin (J/mol·K).
3. Using Molar Mass:
To convert R from J/mol·K to J/kg·K, we need to divide R by the molar mass of nitrogen. The molar mass of nitrogen (N2) is approximately 28 g/mol, which is equivalent to 0.028 kg/mol.
4. Setting Up the Equation:
By substituting the molar mass into the equation, we have:
Cp−Cv=RM
where M is the molar mass of nitrogen in kg. Thus, we can rewrite the equation as:
Cp−Cv=R0.028
5. Final Expression:
Therefore, the final expression relating Cp and Cv for nitrogen in J/kg·K is:
Cp−Cv=R28
Conclusion:
The relationship between the specific heats of nitrogen at constant pressure and volume is:
Cp−Cv=R28
The relationship between the specific heats at constant pressure (Cp) and at constant volume (Cv) for an ideal gas is given by the equation:
Cp−Cv=R
where R is the universal gas constant.
The problem states that we need to express the specific heats in terms of Joules per kilogram Kelvin (J/kg·K). The gas constant R is typically given in Joules per mole Kelvin (J/mol·K).
To convert R from J/mol·K to J/kg·K, we need to divide R by the molar mass of nitrogen. The molar mass of nitrogen (N2) is approximately 28 g/mol, which is equivalent to 0.028 kg/mol.
By substituting the molar mass into the equation, we have:
Cp−Cv=RM
where M is the molar mass of nitrogen in kg. Thus, we can rewrite the equation as:
Cp−Cv=R0.028
Therefore, the final expression relating Cp and Cv for nitrogen in J/kg·K is:
Cp−Cv=R28
The relationship between the specific heats of nitrogen at constant pressure and volume is:
Cp−Cv=R28
Topper's Solved these Questions
- GASEOUS STATE & THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE )
111 Videos
- GASEOUS STATE & THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - Level - 2
40 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE
1 Videos
- GRAVITATIONBOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) TRUE/FALSE
1 Videos
Similar Questions
If CPandCV denote the specific heats of nitrogen per unit mass at constant pressure and constant volume respectively, then
View SolutionCvandCp denote the molar specific heat capacities of a gas at constant volume and constant pressure, respectively. Then
View SolutionCvandCp denote the molar specific heat capacities of a gas at constant volume and constant pressure, respectively. Then
View SolutionCvandCp denote the molar specific heat capacities of a gas at constant volume and constant pressure, respectively. Then
View SolutionCvandCp denote the molar specific heat capacities of a gas at constant volume and constant pressure, respectively. Then
View SolutionIf for hydrogen CP−CV=m and for nitrogen CP−CV=n, where CP and CV refer to specific heats per unit mass respectively at constant pressure and constant volume, the relation between m and n is (molecular weight of hydrogen = 2 and molecular weight or nitrogen = 14)
View SolutionIf for hydrogen CP−CV=m and for nitrogen CP−CV=n, where CP and CV refer to specific heats per unit mass respectively at constant pressure and constant volume, the relation between m and n is (molecular weight of hydrogen = 2 and molecular weight or nitrogen = 14)
View SolutionWhen water is heated from 0∘C to 4∘C and CP and CV are its specific heats at constant pressure and constant volume respectively , then :-
View SolutionIf CpandCv denote the specific heats (per unit mass of an ideal gas of molecular weight M), then
where R is the molar gas constant.
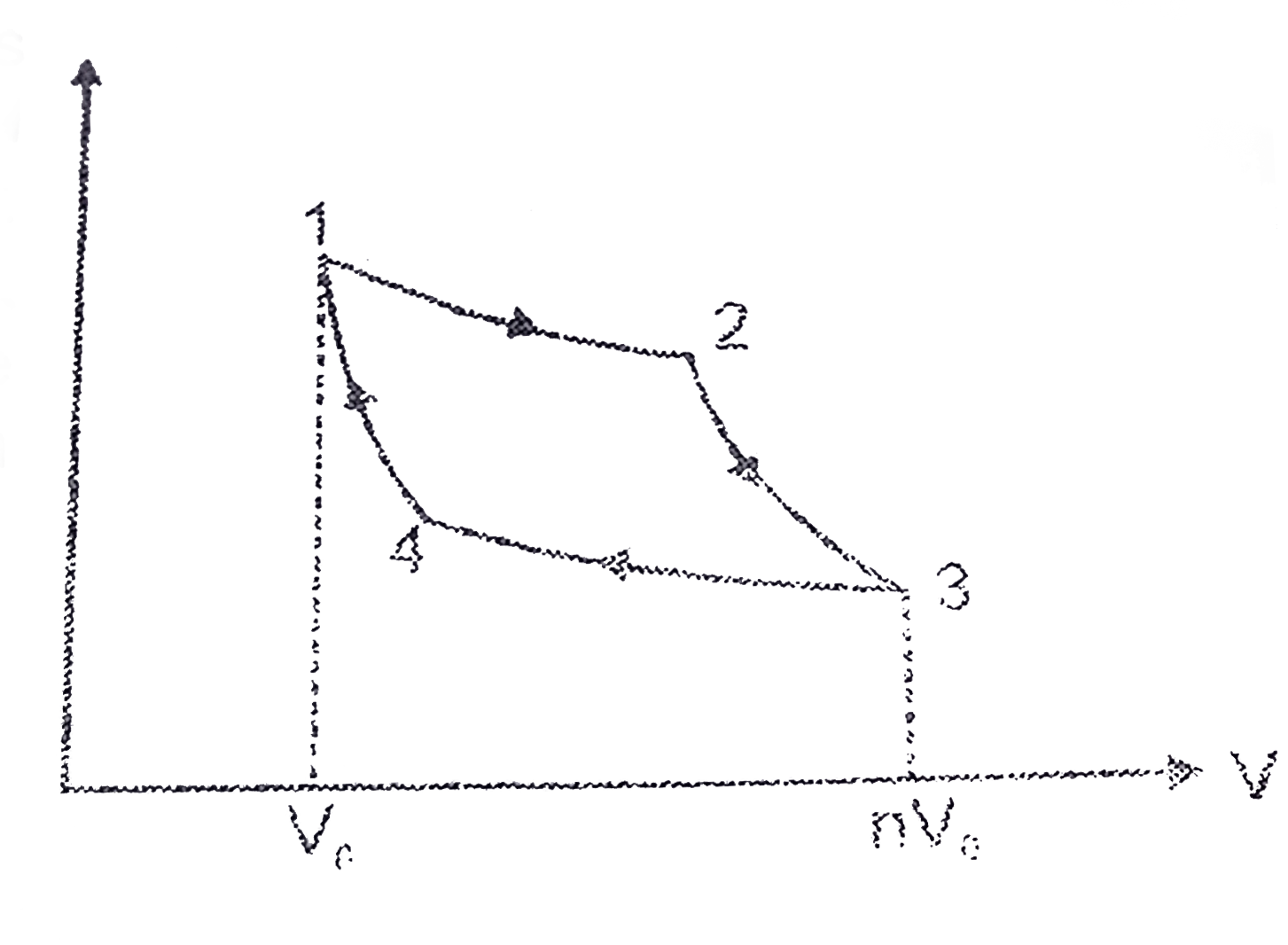
View SolutionA Carnot engine cycle is shown in the Fig. (2). The cycle runs between temperatures TH=αTL=T0(α>T). Minimum and maximum volume at state 1 and states 3 are V0 and nV0 respectively. The cycle uses one mole of an ideal gas with CP/CV=γ. Here CP and CV are the specific heats at constant pressure and volume respectively. You must express all answers in terms of the given parameters {α,n,T0,V0?} and universal gas constant R.
(a) Find P,V,T for all the states
(b) Calculate the work done by the engine in each process: W12,W23,W34,W41.
(c) Calculate Q, the heat absorbed in the cycle.
View Solution
