One end of a metal rod is kept in a furnace. In steady state, the temperature of the rod
Increases
Decreases
Is uniform
is non uniform
The correct Answer is:D
To solve the question about the temperature distribution in a metal rod with one end placed in a furnace, we can follow these steps:
1. Understanding the Setup:
- We have a metal rod with one end (let's call it end A) placed in a furnace, which is at a higher temperature (let's denote this temperature as TH).
- The other end of the rod (let's call it end B) is at a lower temperature (denote this as TL).
2. Steady State Condition:
- The term "steady state" implies that the temperatures at both ends of the rod have stabilized and are constant over time. This means that the temperature at end A is TH and the temperature at end B is TL.
3. Temperature Gradient:
- Since one end of the rod is in contact with a heat source (the furnace) and the other end is cooler, there will be a temperature gradient along the length of the rod. This means that the temperature will change from TH at end A to TL at end B.
4. Non-Uniform Temperature Distribution:
- The temperature will not be the same throughout the rod; instead, it will vary between TH and TL. Therefore, the temperature distribution is non-uniform.
5. Conclusion:
- Based on the analysis, we conclude that in steady state, the temperature of the rod is non-uniform.
Final Answer:
The temperature of the rod is non-uniform.
---
- We have a metal rod with one end (let's call it end A) placed in a furnace, which is at a higher temperature (let's denote this temperature as TH).
- The other end of the rod (let's call it end B) is at a lower temperature (denote this as TL).
- The term "steady state" implies that the temperatures at both ends of the rod have stabilized and are constant over time. This means that the temperature at end A is TH and the temperature at end B is TL.
- Since one end of the rod is in contact with a heat source (the furnace) and the other end is cooler, there will be a temperature gradient along the length of the rod. This means that the temperature will change from TH at end A to TL at end B.
- The temperature will not be the same throughout the rod; instead, it will vary between TH and TL. Therefore, the temperature distribution is non-uniform.
- Based on the analysis, we conclude that in steady state, the temperature of the rod is non-uniform.
The temperature of the rod is non-uniform.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Level -250videos
PROPERTIES OF MATTER
- BOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Main (Archive) Level - 148videos
PROPERTIES OF MATTER
- BOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced (Archive) Level - 2 (MATRIX MATCH TYPE)1videos
PROPERTIES OF MATTER
- BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-K10videos
MOVING CHARGES & MAGNETISM
- BOOK - VMC MODULES ENGLISHCHAPTER - QUIZEXERCISE - PHYSICS30videos
QUIZ
Similar Questions
Assertion : Heat is supplied at constant rate from one end of a conducting rod. In steady state, temperature of all points of the rod becomes uniform.
Reason : In steady state, temperature of rod does not increase.View Solution
Two ends of a rod of uniform cross sectional area are kept at temperature 3T0 and T0 as shown. Thermal conductivity of rod varies as k=αT, (where α is a constant and T is absolute temperature). In steady state, the temperature of the middle section of the rod isView SolutionA rod is heated at one end as shown in figure. In steady state temperature of different sections becomes constant but not same. Why so?
 . View Solution
. View SolutionOne end of a rod length 20cm is inserted in a furnace at 800K. The sides of the rod are covered with an insulating material and the other end emits radiation like a blackbody. The temperature of this end is 750K in the steady state. The temperature of the surrounding air is 300K. Assuming radiation to be the only important mode of energy transfer between the surrounding and the open end of the rod, find the thermal conductivity of the rod. Stefan constant σ=6.0×10−1Wm−2K−4 .
View SolutionOne end of a rod length 20cm is inserted in a furnace at 800K. The sides of the rod are covered with an insulating material and the other end emits radiation like a blackbody. The temperature of this end is 750K in the steady state. The temperature of the surrounding air is 300K. Assuming radiation to be the only important mode of energy transfer between the surrounding and the open end of the rod, find the thermal conductivity of the rod. Stefan constant σ=6.0×10−1Wm−2K−4 .
View SolutionOne end of a rod of length 20cm is inserted in a furnace at 800K The sides of the rod are covered with an insulating material and the other end emits radiation like a black body. The temperature of this end is 750K in the steady state The temperature of the surrounding air is 300K Assuming radiation to be the only important mode of energy transfer between the surrounding and the open end of the rod, find the thermal conductivity of the rod Stefan's constant σ=6.0×10−8Wm−2K−4 .
View SolutionA brass rod of length 1m is fixed to a vertical wall at one end, with the other end keeping free to expand. When the temperature of the rod is increased by 120∘C, the length increases by 3cm. What is the strain?
View SolutionSix identical conducting rods are connected as shown in figure. In steady state temperature of point ϕ1 is fixed at 100∘C and temperature of ϕ6 at −80∘C

Q. What is the temperature of ϕ3?View SolutionSix identical conducting rods are connected as shown in figure. In steady state temperature of point ϕ1 is fixed at 100∘C and temperature of ϕ6 at −80∘C
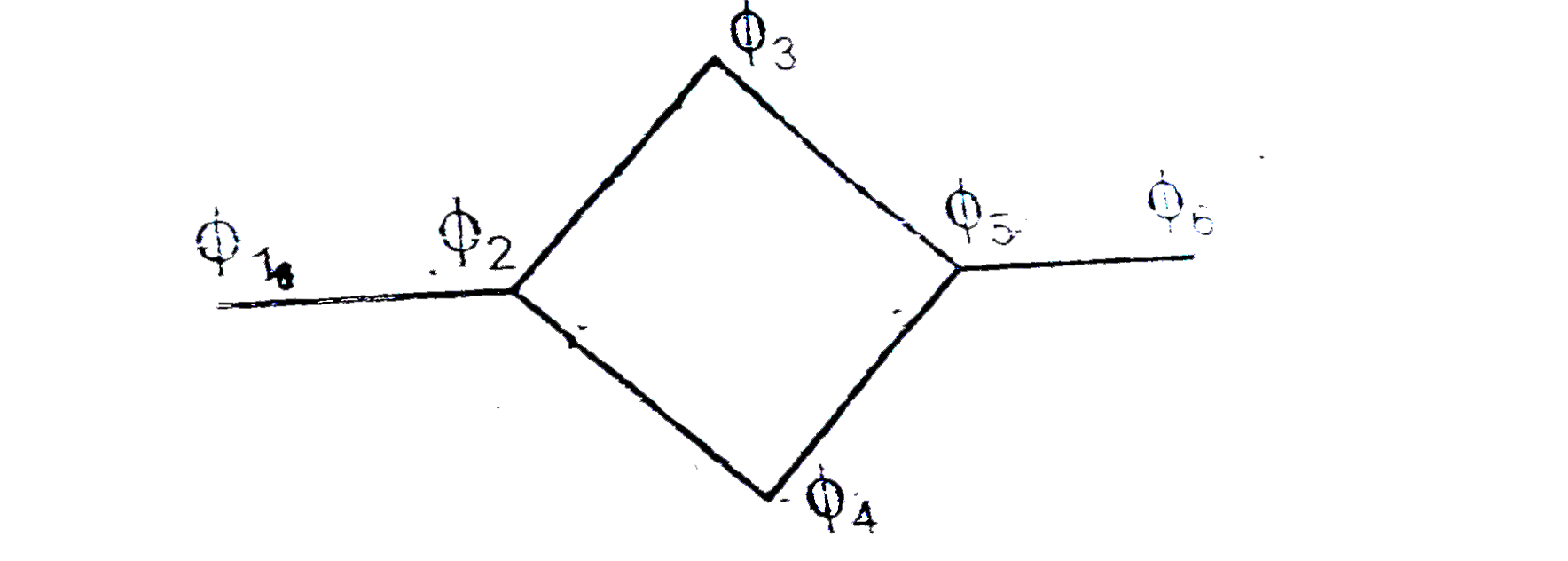
Q. What is the temperature of ϕ5?View SolutionSix identical conducting rods are connected as shown in figure. In steady state temperature of point ϕ1 is fixed at 100∘C and temperature of ϕ6 at −80∘C
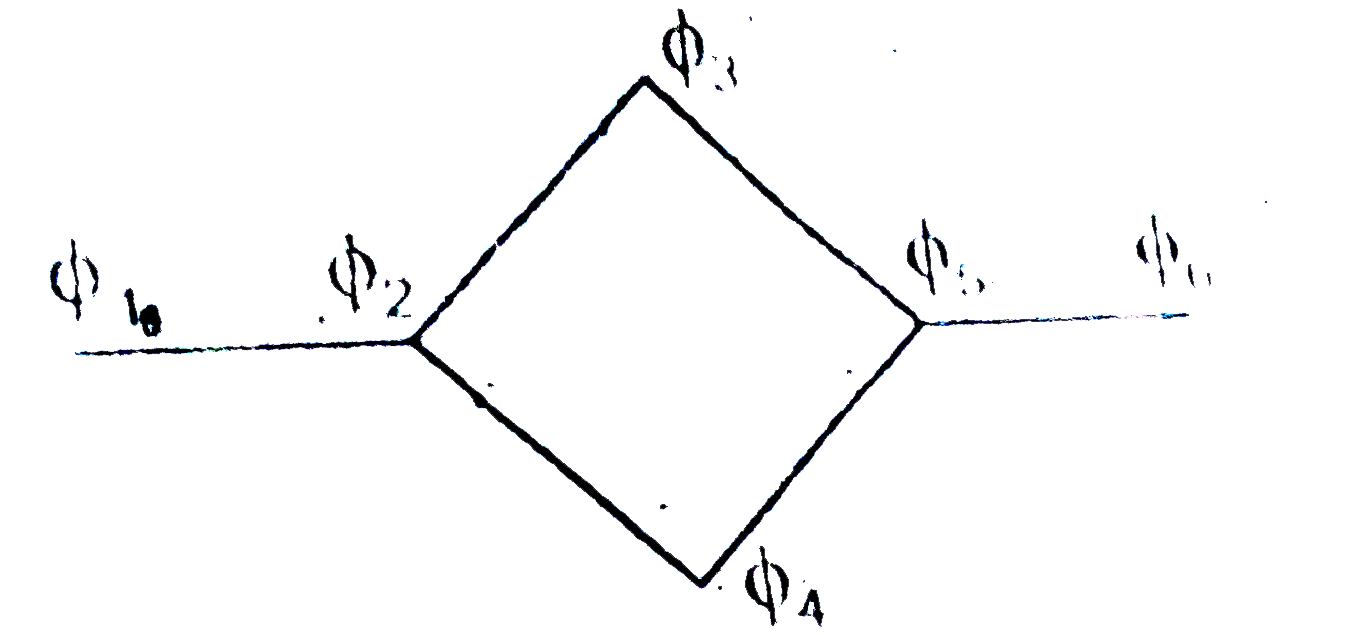
Q. What is the temperature of ϕ2?View Solution

