One component of a solution follows Raoult's over the entire range 0≤x1≤1. The second component must follow Raoult's law in the range when x2 is
close to zero
close to 1
0≤x2≤0.5
0≤X2≤1
To solve the problem, we need to analyze the conditions given about the two components of the solution and their adherence to Raoult's law.
1. Understanding Raoult's Law: Raoult's law states that the partial vapor pressure of each component in an ideal solution is equal to the vapor pressure of the pure component multiplied by its mole fraction in the solution. For component A, we denote its mole fraction as x1 and for component B as x2.
2. Given Information: We know that one component (let's say component A) follows Raoult's law over the entire range of mole fractions from 0 to 1. This means that for all values of x1 (from 0 to 1), the behavior of A is ideal.
3. Mole Fraction Relationship: The mole fractions of the two components must satisfy the relationship:
x1+x2=1
where x1 is the mole fraction of component A and x2 is the mole fraction of component B.
4. Analyzing Component B: Since x1 ranges from 0 to 1, x2 must range from 1 to 0. If component A follows Raoult's law for all values of x1, we need to determine the range of x2 for component B to also follow Raoult's law.
5. Conclusion about Component B: For component B to follow Raoult's law, it must be true that x2 is in the range where it can still behave ideally. Since x1 can take any value from 0 to 1, x2 must be able to take values from 0 to 1 as well. However, the problem states that the second component must follow Raoult's law only in a certain range.
6. Final Determination: Since x1 can be 1 (pure A) and 0 (pure B), the second component (B) must follow Raoult's law when x2 is in the range of 0 to 1. However, if x1 is at extremes (0 or 1), x2 will be at the opposite extreme (1 or 0). Thus, for component B to follow Raoult's law, it must hold true when x2 is closer to 0, meaning it can only follow Raoult's law when it is not in the pure state.
Final Answer:
The second component must follow Raoult's law in the range when x2 is closer to 0.
x1+x2=1
where x1 is the mole fraction of component A and x2 is the mole fraction of component B.
The second component must follow Raoult's law in the range when x2 is closer to 0.
Topper's Solved these Questions
- THEORY OF SOLUTIONSBOOK - VMC MODULES ENGLISHCHAPTER - THEORY OF SOLUTIONSEXERCISE - LEVEL 2
65 Videos
- THEORY OF SOLUTIONSBOOK - VMC MODULES ENGLISHCHAPTER - THEORY OF SOLUTIONSEXERCISE - JEE Main (Archive)
57 Videos
- THEORY OF SOLUTIONSBOOK - VMC MODULES ENGLISHCHAPTER - THEORY OF SOLUTIONSEXERCISE - JEE Advanced (Archive)
31 Videos
- THE SOLID STATEBOOK - VMC MODULES ENGLISHCHAPTER - THE SOLID STATEEXERCISE - EXERCISE-J
10 Videos
- THERMOCHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - THERMOCHEMISTRYEXERCISE - JEE ADVANCED (ARCHIVE)
31 Videos
Similar Questions
Knowledge Check
What are the conditions for an ideal solution which obeys Raoult's law over the entire range of concentration ?
AΔmixH=0,ΔmixV=0,PTotal=p∘AxA+p∘BxBBΔmixH=+ve,ΔmixV=0,PTotal=p∘AxA+p∘BxBCΔmixH=0,ΔmixV=+ve,PTotal=p∘AxA+p∘BxBDΔmixH=0,ΔmixV=0,PTotal=p∘BxBWhich of the following solutions is an example of negative deviation from Raoult's law ?
AAcetone +EthanolBCarbon tetrachloride + ChloroformCAcetone + ChloroformDWater + Ethanol
Statement - If one component obeyed Raoult's law over a certain range of composition, the other component would not obey Henry's law in that range.
Explanation - Raoult's law is a special case of Henry's law.
View Solution1.The range of f(x)=x2+x+1 is
View SolutionIn an ideal solution of two liquids, each component obey Raoult's law.
View SolutionState Raoult's law for the solution containing volatile components. What is the similarity .
between Raoult's law and Henry's law ?
View SolutionState Raoult's law for a binary solution containing volatile components.
View SolutionBased on the given diagram, which of the following statements regarding the homogenous solution of two volatile liquids are correct? (1) Plots AD and BC show that Raoult's law is obeyed for the solution in which B is a solvent and A is the solute and as well as for that in which A is solvent and B is solute. (2) Plot CD shows that Dalton's law of partial pressures is obeyed by the binary solution of components A and B. (3) EF + EG = EH; and AC and BD correspond to the vapour pressure of the pure solvents A and B respectively.
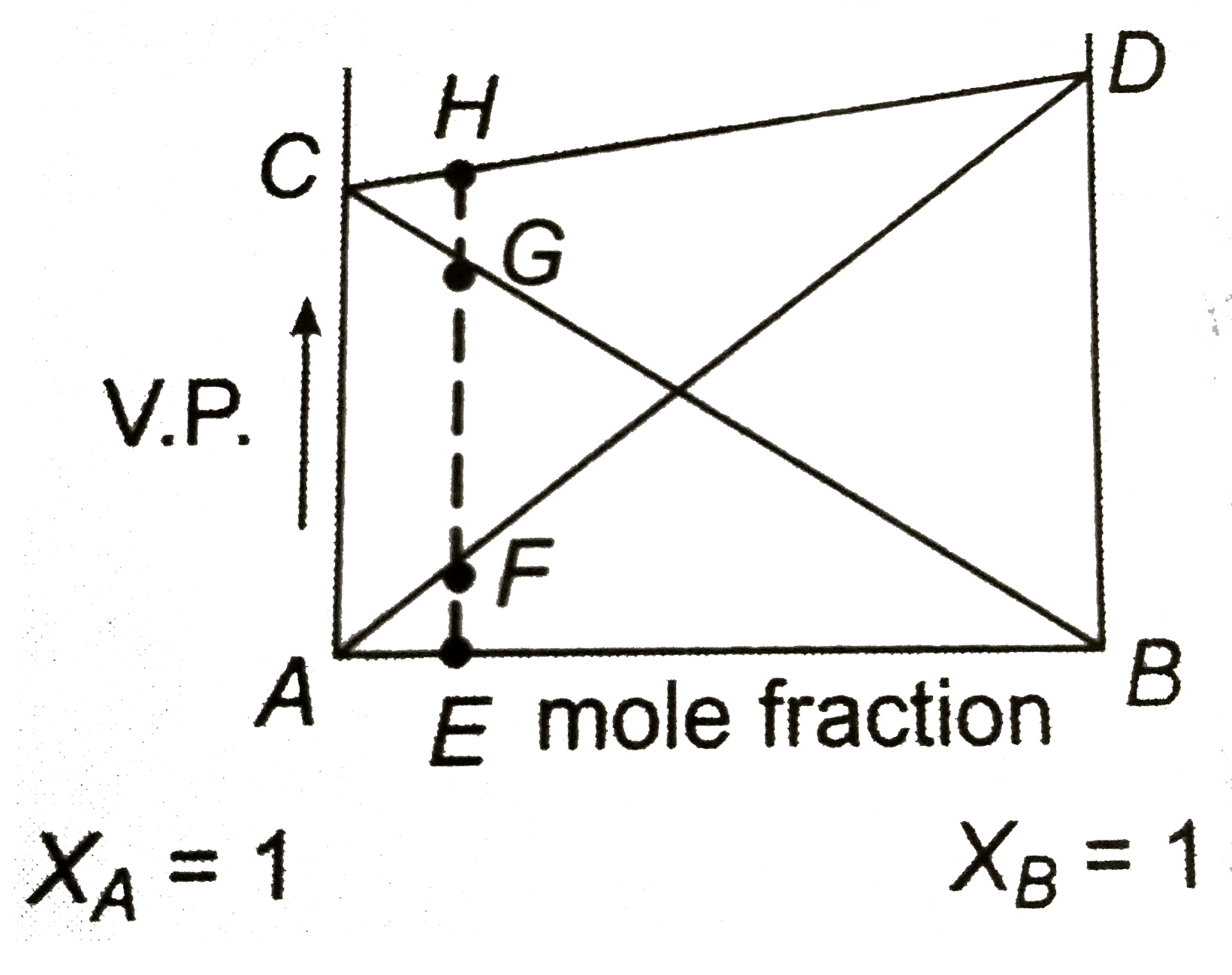
View Solution
