For a reaction between gaseous compounds, 2A+B→C+D , the reaction rate law is rate k[A][B]. If the volume of the container is made 1/4th of the initial, then what will be the rate of reaction as compared to the initial rate?
16 times
4 times
18 times
116 times
The correct Answer is:A
To solve the problem, we need to analyze how the change in volume affects the concentrations of the reactants and subsequently the rate of the reaction.
1. Understand the Reaction and Rate Law:
The reaction is given as:
2A+B→C+D
The rate law for this reaction is:
Rate=k(A)(B)
where k is the rate constant, and (A) and (B) are the concentrations of reactants A and B, respectively.
2. Determine the Effect of Volume Change:
It is stated that the volume of the container is reduced to 14 of its initial volume. If the initial volume is V, the new volume V′ becomes:
V′=V4
3. Calculate Concentrations:
Concentration is defined as the number of moles of a substance divided by the volume of the container. If the number of moles of A and B remains constant, the new concentrations can be calculated as follows:
(A)′=nAV′=nAV4=4nAV=4(A)
(B)′=nBV′=nBV4=4nBV=4(B)
Thus, both concentrations of A and B increase by a factor of 4.
4. Substitute New Concentrations into the Rate Law:
The new rate of reaction Rate′ can be expressed as:
Rate′=k(A)′(B)′=k(4(A))(4(B))=16k(A)(B)
5. Compare the New Rate to the Initial Rate:
The initial rate Rate is:
Rate=k(A)(B)
Therefore, we can express the new rate in terms of the initial rate:
Rate′=16×Rate
Conclusion:
The rate of the reaction, when the volume is reduced to one-fourth of its initial value, becomes 16 times the initial rate.
The reaction is given as:
2A+B→C+D
The rate law for this reaction is:
Rate=k(A)(B)
where k is the rate constant, and (A) and (B) are the concentrations of reactants A and B, respectively.
It is stated that the volume of the container is reduced to 14 of its initial volume. If the initial volume is V, the new volume V′ becomes:
V′=V4
Concentration is defined as the number of moles of a substance divided by the volume of the container. If the number of moles of A and B remains constant, the new concentrations can be calculated as follows:
(A)′=nAV′=nAV4=4nAV=4(A)
(B)′=nBV′=nBV4=4nBV=4(B)
Thus, both concentrations of A and B increase by a factor of 4.
The new rate of reaction Rate′ can be expressed as:
Rate′=k(A)′(B)′=k(4(A))(4(B))=16k(A)(B)
The initial rate Rate is:
Rate=k(A)(B)
Therefore, we can express the new rate in terms of the initial rate:
Rate′=16×Rate
The rate of the reaction, when the volume is reduced to one-fourth of its initial value, becomes 16 times the initial rate.
Topper's Solved these Questions
- CHEMICAL KINETICS BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL KINETICS EXERCISE - Level-2 ( Numerical Value Type for JEE Main )
15 Videos
- CHEMICAL KINETICS BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL KINETICS EXERCISE - JEE Main (Archive)
56 Videos
- CHEMICAL KINETICS BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL KINETICS EXERCISE - Level-1
75 Videos
- CHEMICAL EQUILIBRIUMBOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - IN-CHAPTER EXERCISE - G
10 Videos
- CHEMICAL THERMODYNAMICS BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL THERMODYNAMICS EXERCISE - IN - CHAPTER EXERCISE - L
10 Videos
Similar Questions
Conisder a gaseous reaction, the rate of which is given by k[A][B]. The volume of the reaction vessel containing these gases is suddenly reduced to 1/4th of the initial volume. The rate of the reaction as compared with original rate is
View SolutionIn the reaction 2A+B→A2B, Rate = k[A]^2 [ B ] if the concentration of A is doubled and that of B is halved, then the rate of reaction will:
View Solution
Knowledge Check
The rate of a gaseous reaction is given by the expresison k[A]2[B]3. The volume of the reaction vessel is suddenly reduced to one-half of the initial volume. The reaction rate relative to the original rate will be
A18aB12aC2aD32a
The rate expression for a reaction is rate =k[A]3/2[B]−1, the order of reaction is
View SolutionThe rate of a gaseous reaction is given by the expression k[A][B]. If The volume of reaction vessel is suddenly reduced to one-fourth of the initial volume, the reaction rate relative to the original rate will be :
View SolutionThe rate of a gaseous reaction is given by the expression k[A][B]. If The volume of reaction vessel is suddenly reduced to one-fourth of the initial volume, the reaction rate relative to the original rate will be :
View Solution(a) For a reaction ,A+B→Product the rate law is given rate =k[A]1[B]2 .What is the order of the reaction ?
(b) write the unit of rate constant k for the first order reaction
View SolutionIf the rate of reaction between A and B is given by rate =k[A][B]2, then the reaction is
View SolutionFor the reaction 2A+B→C, the values of ini concentrations are given in the table below. The rate law for the reaction is:
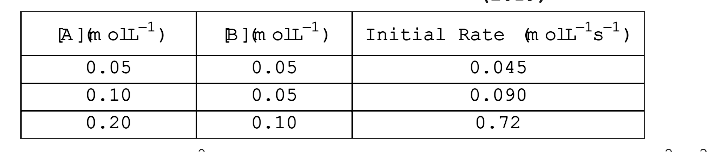
View SolutionThe rate law for reaction A+2B⇔C+2D will be :
View Solution
