For torsional oscillator, √I/C has dimensions of ? Where C is torsional constant and I is moment of inertia :
M0L0T−1
M0L0T1
M0L1T−1
M0L0T2
The correct Answer is:B
To find the dimensions of √IC for a torsional oscillator, we will break down the problem into steps.
Step 1: Identify the dimensions of moment of inertia I
The moment of inertia I is defined as:
I=m⋅r2
where m is mass and r is the radius (or distance from the axis of rotation).
The dimensions of I can be expressed as:
Dimension of I=(M)(L2)=ML2
Step 2: Identify the dimensions of the torsional constant C
The torsional constant C is defined as the ratio of torque τ to the angle of twist ϕ:
C=τϕ
Since ϕ (angle of twist) is dimensionless, the dimensions of C are the same as the dimensions of torque τ.
Torque is defined as:
τ=Force×Distance
The dimension of force is given by:
Force=(M)(L)(T−2)
Thus, the dimension of torque is:
Dimension of τ=(M)(L)(T−2)⋅(L)=ML2T−2
Therefore, the dimensions of C are:
Dimension of C=ML2T−2
Step 3: Calculate the dimensions of IC
Now we can find the dimensions of IC:
IC=ML2ML2T−2=ML2ML2⋅T2=T2
Step 4: Find the dimensions of √IC
Taking the square root of the dimensions we found:
√IC=√T2=T
Final Answer
Thus, the dimensions of √IC are:
Dimension of √IC=T
The moment of inertia I is defined as:
I=m⋅r2
where m is mass and r is the radius (or distance from the axis of rotation).
Dimension of I=(M)(L2)=ML2
The torsional constant C is defined as the ratio of torque τ to the angle of twist ϕ:
C=τϕ
Since ϕ (angle of twist) is dimensionless, the dimensions of C are the same as the dimensions of torque τ.
τ=Force×Distance
The dimension of force is given by:
Force=(M)(L)(T−2)
Thus, the dimension of torque is:
Dimension of τ=(M)(L)(T−2)⋅(L)=ML2T−2
Therefore, the dimensions of C are:
Dimension of C=ML2T−2
Now we can find the dimensions of IC:
IC=ML2ML2T−2=ML2ML2⋅T2=T2
Taking the square root of the dimensions we found:
√IC=√T2=T
Thus, the dimensions of √IC are:
Dimension of √IC=T
Topper's Solved these Questions
- UNITS, MEASUREMENTS & ERRORSBook:VMC MODULES ENGLISHChapter:UNITS, MEASUREMENTS & ERRORSExercise:Efficient
Explore 50 Videos
- UNITS, MEASUREMENTS & ERRORSBook:VMC MODULES ENGLISHChapter:UNITS, MEASUREMENTS & ERRORSExercise:Impeccable
Explore 51 Videos
- UNITS, MEASUREMENTS & ERRORSBook:VMC MODULES ENGLISHChapter:UNITS, MEASUREMENTS & ERRORSExercise:IN - CHAPTER EXERCISE - B
Explore 10 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBook:VMC MODULES ENGLISHChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTIONExercise:IMPECCABLE
Explore 56 Videos
- WAVE MOTIONBook:VMC MODULES ENGLISHChapter:WAVE MOTIONExercise:JEE ADVANCED ARCHIVE LEVEL 2 (TRUE FALSE TYPE)
Explore 4 Videos
Similar Questions
The ratio of the dimensions of plank's constant and that of the moment of inertia is the dimension of
View SolutionThe ratio of the dimensions of plank's constant and that of the moment of inertia is the dimension of
View SolutionThe ratio of the dimensions of plank's constant and that of the moment of inertia is the dimension of
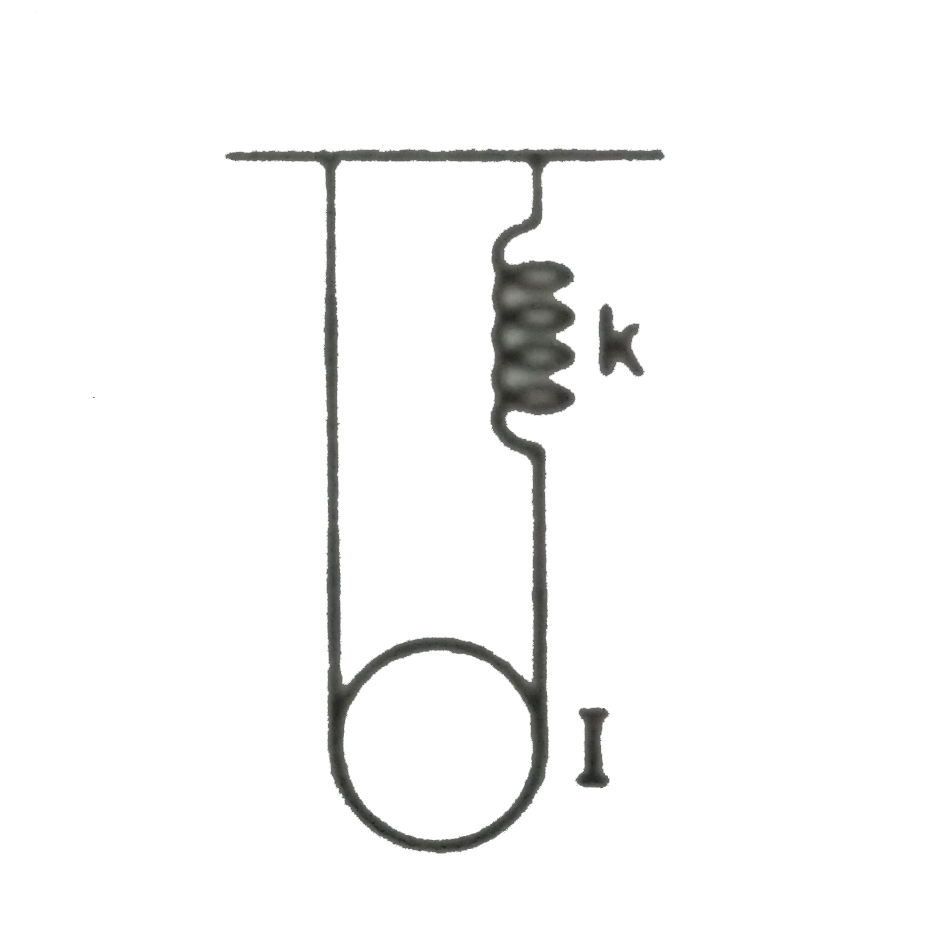
View SolutionThe pulley shown in figure has a moment of inertias I about its axis and mass m. find the time period of vertical oscillation of its centre of mass. The spring has spring constant k and the string does not slip over the pulley.
View SolutionA circular disc of mass 10kg is suspended by a wire attahced to its centre. The wire is twisted by rotating the disc and released. The period of torsional oscillations is found to be 1.5s. The radius of the disc is 15cm. Determing the torsional spring constant of the wire. (Torsional spring constant α is definied by the relation J=−αθ, where J is the restoring coubple and θ the angle of twist.
View SolutionA uniform disc of radius 5.0 cm and mass 200g is fixed at its centre to a metal wire, the other end of which is fixed with a clamp. The hanging disc is rotated about the wire through an angle and is released. If the disc makes torsional oscillations with time period 0.20s, find the torsional constant of the wire.
View SolutionThe moment of inertia of a uniform rod about a perpendicular axis passing through one end is I1. The same rod is bent into a ring and its moment of inertia about a diameter is I2. Then I1/I2 is
View SolutionA moving coil galvanometer experience torque =ki, where i is current. If N coils of area A each and moment of inertia I is kept in magnetic field B.
(i) If for current i deflection is π2, the torsional constant of spring is xBiNAπ. Find x2
(ii) If a charge Q is passed suddenly through the galvanometer, the maximum angle of deflection is Q√BNπAxI. Find x2
View SolutionA constant external torque τ acts for a very brief period △t on a rotating system having moment of inertia I then
View SolutionA moving coil galvanometer consists of a coil of N turns are area A suspended by a thin phosphor bronze strip in radial magnetic field B. The moment of inertia of the coil about the axis of rotation is l and c is the torsional constant of the phosphor bronze strip. When a current i is passed through the coil, it deffects through an angle θ
Magnitude of the torque experienced by the coil is independent of
View Solution
