A machine, which is 72 percent efficient, uses 36 joules of energy in lifting up 1kg mass through a certain distance. The mass is the allowed to fall through that distance. The velocity at the end of its fall is
6.6ms−1
7.2ms−1
8.1ms−1
9.2ms−1
The correct Answer is:B
To solve the problem step by step, we will follow these steps:
Step 1: Understand the Work Done by the Machine
The machine is 72% efficient and uses 36 joules of energy to lift a 1 kg mass. The work done by the machine can be calculated using the efficiency formula:
Efficiency=Useful Work OutputTotal Work Input×100
Given that the efficiency is 72%, we can rearrange the formula to find the useful work output (which is the potential energy gained by the mass):
Useful Work Output=Efficiency×Total Work Input
Step 2: Calculate the Useful Work Output
Substituting the values into the equation:
Useful Work Output=72100×36 joules
Calculating this gives:
Useful Work Output=0.72×36=25.92 joules
Step 3: Relate Work Done to Potential Energy
The work done on the mass is equal to the change in potential energy when it is lifted:
mgh=25.92 joules
Where:
- m=1 kg
- g=9.81 m/s2 (acceleration due to gravity)
- h is the height lifted.
Step 4: Solve for Height h
Rearranging the equation to find h:
h=25.92mg=25.921×9.81≈2.64 meters
Step 5: Calculate the Velocity at the End of the Fall
When the mass falls back down, the potential energy converts back into kinetic energy. The potential energy at the height h will equal the kinetic energy just before it hits the ground:
mgh=12mv2
Step 6: Cancel Mass and Solve for Velocity v
Since mass m appears on both sides, we can cancel it out:
gh=12v2
Rearranging gives:
v2=2gh
Substituting the values:
v2=2×9.81×2.64
Calculating this gives:
v2=51.8⟹v≈√51.8≈7.2 m/s
Final Answer
The velocity at the end of its fall is approximately 7.2 m/s.
---
The machine is 72% efficient and uses 36 joules of energy to lift a 1 kg mass. The work done by the machine can be calculated using the efficiency formula:
Substituting the values into the equation:
The work done on the mass is equal to the change in potential energy when it is lifted:
- m=1 kg
- g=9.81 m/s2 (acceleration due to gravity)
- h is the height lifted.
Rearranging the equation to find h:
When the mass falls back down, the potential energy converts back into kinetic energy. The potential energy at the height h will equal the kinetic energy just before it hits the ground:
Since mass m appears on both sides, we can cancel it out:
The velocity at the end of its fall is approximately 7.2 m/s.
Topper's Solved these Questions
- WORK ENERGY AND POWER BOOK - VMC MODULES ENGLISHCHAPTER - WORK ENERGY AND POWER EXERCISE - EFFICIENT
50 Videos
- WORK ENERGY AND POWER BOOK - VMC MODULES ENGLISHCHAPTER - WORK ENERGY AND POWER EXERCISE - IMPECCABLE
54 Videos
- WORK ENERGY AND POWER BOOK - VMC MODULES ENGLISHCHAPTER - WORK ENERGY AND POWER EXERCISE - IMPECCABLE
54 Videos
- WAVE MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - WAVE MOTIONEXERCISE - JEE ADVANCED ARCHIVE LEVEL 2 (TRUE FALSE TYPE)
4 Videos
Similar Questions
A machine which is 75 percent efficient, uses 12 joules of energy in lifting up a 1 kg mass through a certain distance. The mass is then allowed to fall through that distance. The velocity at the end of its fall is (in ms−1)
View SolutionA ball falls freely from rest. The ratio of the distance travelled in first, second, third and fourth second is
View SolutionOn a frictionless horizontal surface , assumed to be the x−y plane , a small trolley A is moving along a straight line parallel to the y−axis ( see figure) with a constant velocity of (√3−1)m/s . At a particular instant , when the line OA makes an angle of 45(∘) with the x− axis , a ball is thrown along the surface from the origin O. Its velocity makes an angle ϕ with the x− axis and it hits the trolley .
(a) The motion of the ball is observed from the frame of the trolley . Calculate the angle θ made by the velocity vector of the ball with the x−axis in this frame .
(b) Find the speed of the ball with respect to the surface , if ϕ=(4θ)/(3).

View SolutionA metal ball falls through water with terminal velocity 20 ms−1. When the ball is allowed to fall through vacuum, then
View SolutionA mass of 1kg carrying a charge of 2C is accelerated through a potential of 1V. The velocity acquired by it is
View SolutionA body freely falling from the rest has velocity v after it falls through a height h the distance it has to fall down for its velocity to become double is
View SolutionAn electron falls from rest through a vertical distance h in a uniform and vertically upward directed electric field E. the direction of electric field is now reversed, keeping its magnitude the same. A proton is allowed to fall from rest in it through the same vertical distance h.The time of fall of the electron, in comparison to the time of flal of the proton is
View SolutionA cord is wound round the circumference of a solid cylinder radius R and mass M. The axis of the cylinder is horizontal. A weight mg is attached to the end of the cord and falls from rest. After falling through a distance h.
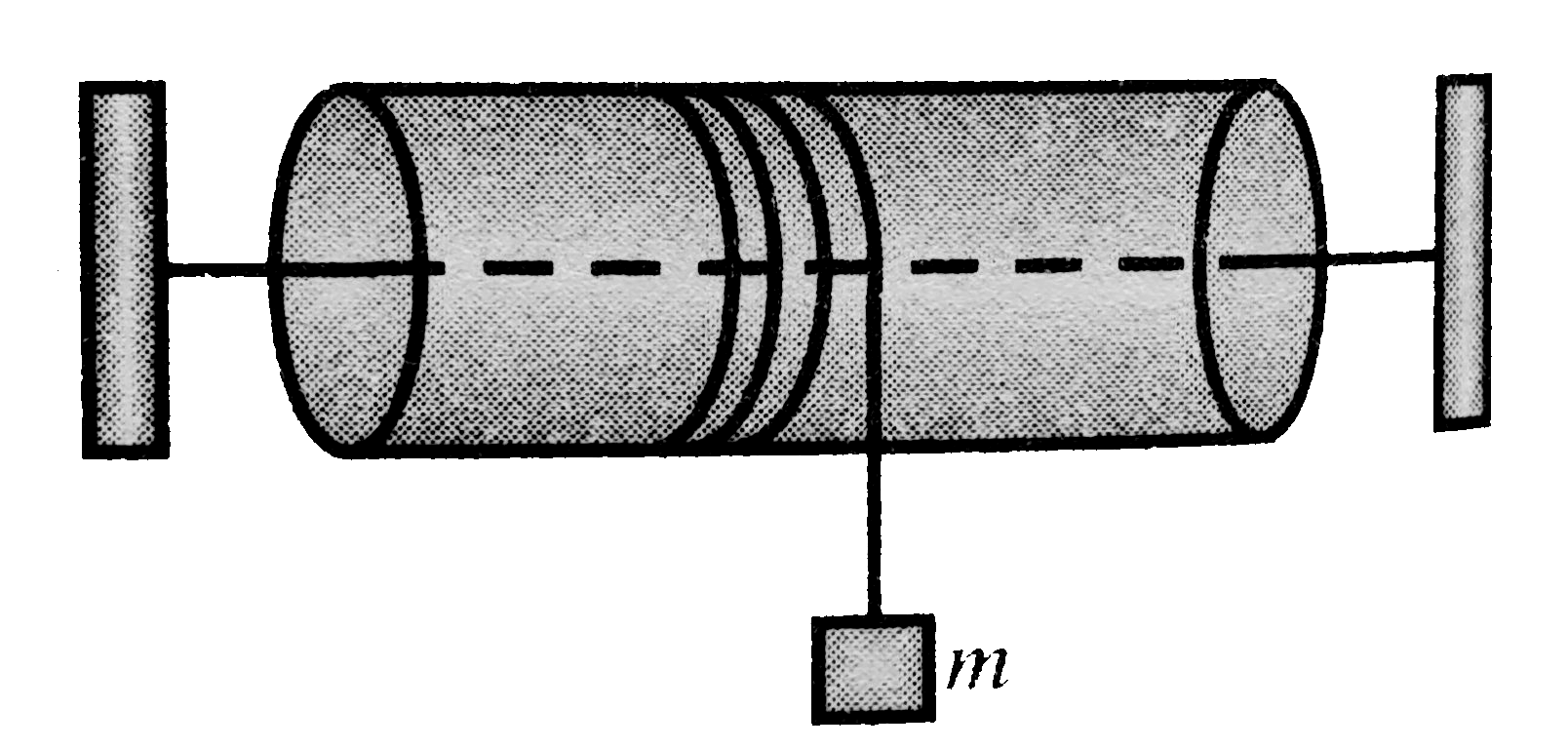
If the mass starts from rest and falls a distance h, then its speed at that instant is:
View SolutionA cord is wound around the circumference of wheel of radius r. The axis of the wheel is horizontal and MI is I. A weight mg is attached to the end of the cord and falls from rest. After falling through a distance h, the angular velocity of the wheel will be
View SolutionA heavy mass M resting on the ground is connected to a lighter mass m through a light inextensible string passing over an ideal pulley. The string connected to mass M is loose. Let lighter mass m be allowed to fall freely through a height h such that the string becomes taut. If t is the time from this instant onward when the heavier mass again makes contact with the ground and ΔE is change in kinetic energy then
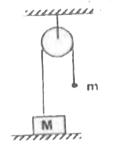
View Solution
