For one mole of an ideal gas, increasing the temperature from 10∘C to 20∘C
increases the average kinetic energy by two times
increases the rms velocity of √2 times
increases the rms velocity by two times
increases both the average kinetic energy and rms velocity, but not significantly
The correct Answer is:D
To solve the problem of determining the effect of increasing the temperature of one mole of an ideal gas from 10∘C to 20∘C, we will follow these steps:
Step 1: Convert Celsius to Kelvin
To analyze the behavior of the gas, we first need to convert the temperatures from Celsius to Kelvin. The conversion formula is:
T(K)=T(°C)+273
For 10∘C:
T1=10+273=283K
For 20∘C:
T2=20+273=293K
Step 2: Calculate Average Kinetic Energy
The average kinetic energy (E) of an ideal gas is given by the formula:
E=32kT
where k is the Boltzmann constant and T is the temperature in Kelvin.
For E1 at T1:
E1=32k(283)
For E2 at T2:
E2=32k(293)
Step 3: Find the Ratio of Average Kinetic Energies
To find the ratio of the average kinetic energies E2 to E1:
E2E1=32k(293)32k(283)=293283
Calculating this gives:
E2E1≈1.0354
This indicates that the average kinetic energy increases by approximately 3.54%.
Step 4: Calculate Root Mean Square Velocity
The root mean square velocity (vrms) is given by the formula:
vrms=√3RTM
where R is the universal gas constant, T is the temperature in Kelvin, and M is the molar mass.
For vrms1 at T1:
vrms1=√3R(283)M
For vrms2 at T2:
vrms2=√3R(293)M
Step 5: Find the Ratio of Root Mean Square Velocities
To find the ratio of vrms2 to vrms1:
vrms2vrms1=√3R(293)/M√3R(283)/M=√293283
Calculating this gives:
vrms2vrms1≈√1.0354≈1.0176
This indicates that the root mean square velocity increases by approximately 1.76%.
Conclusion
From the calculations:
- The average kinetic energy increases by approximately 3.54%.
- The root mean square velocity increases by approximately 1.76%.
Final Answer
Both the average kinetic energy and the root mean square velocity increase, but not by a factor of 2. The increases are approximately 1.0354 times for kinetic energy and 1.0176 times for root mean square velocity.
To analyze the behavior of the gas, we first need to convert the temperatures from Celsius to Kelvin. The conversion formula is:
T(K)=T(°C)+273
For 10∘C:
T1=10+273=283K
For 20∘C:
T2=20+273=293K
The average kinetic energy (E) of an ideal gas is given by the formula:
E=32kT
where k is the Boltzmann constant and T is the temperature in Kelvin.
E1=32k(283)
For E2 at T2:
E2=32k(293)
To find the ratio of the average kinetic energies E2 to E1:
E2E1=32k(293)32k(283)=293283
Calculating this gives:
E2E1≈1.0354
This indicates that the average kinetic energy increases by approximately 3.54%.
The root mean square velocity (vrms) is given by the formula:
vrms=√3RTM
where R is the universal gas constant, T is the temperature in Kelvin, and M is the molar mass.
vrms1=√3R(283)M
For vrms2 at T2:
vrms2=√3R(293)M
To find the ratio of vrms2 to vrms1:
vrms2vrms1=√3R(293)/M√3R(283)/M=√293283
Calculating this gives:
vrms2vrms1≈√1.0354≈1.0176
This indicates that the root mean square velocity increases by approximately 1.76%.
From the calculations:
- The average kinetic energy increases by approximately 3.54%.
- The root mean square velocity increases by approximately 1.76%.
Both the average kinetic energy and the root mean square velocity increase, but not by a factor of 2. The increases are approximately 1.0354 times for kinetic energy and 1.0176 times for root mean square velocity.
Topper's Solved these Questions
- STATES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - EFFICIENT
45 Videos
- SOME BASIC CONCEPTS OF CHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - SOME BASIC CONCEPTS OF CHEMISTRYEXERCISE - IN - CHAPTER EXERCISE - H
8 Videos
- STATES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - IN-CHAPTER EXERCISE-I
10 Videos
Similar Questions
Temperature of an ideal gas increases in:
View SolutionThe temperature of an ideal gas increases in an:
View Solution70 calories of heat is required to raise the temperature of 2 moles of an ideal gas at constant pressure from 40∘C to 45∘C (R=2cal/mol−.∘C). The gas may be
View SolutionInternal energy of two moles of an ideal gas at a temperature of 127∘Cis1200R. Then find the molar specific heat of the gas at constant pressure?
View SolutionIn an isothermal expansion of one mole of an ideal gas against vacuum from 10 litre to 100 litre at 27∘C, the quantity of heat absorbed by the gas is
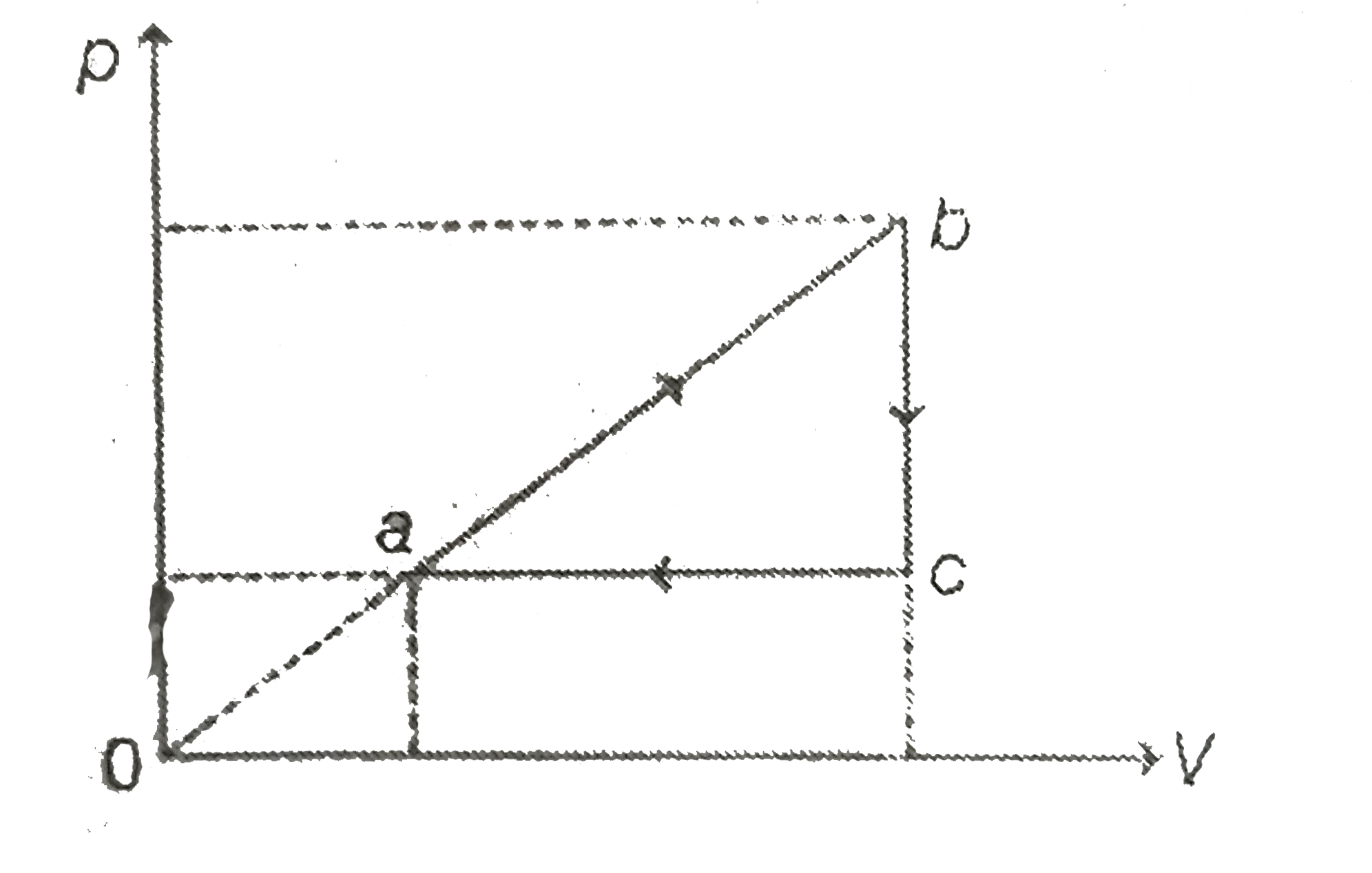
View SolutionFigure illustrates a cycle conducted with n moles of an ideal gas. In the states a and b the gas temperatures are Ta and Tb respectively. Temperature of the gas in the state C is ltbr)
View SolutionA rigid container of negligible heat capacity contains one mole of an ideal gas. The temperatures of the gas increases by 1∘C if 3.0 cal of heat is added to it. The gas may be
View SolutionFor the reversible isothermal expansion of one mole of an ideal gas at 300 K, from a volume of 10dm3 to 20dm3,ΔH is -
View SolutionFive moles of an ideal monoatomic gas with an initial temperature of 150∘C expand and in the process absorb 1500 J of heat and does 2500 J of work. The final temperature of the gas in .∘C is (ideal gas constant R=8.314JK−1mol−1)
View Solution70 calories of heat required to raise the temperature of 2 moles of an ideal gas at constant pressure from 30∘C→35∘C. The amount of heat required (in calories) to raise the temperature of the same gas through the same range (30∘C→35∘C) at constant volume is:
View Solution
