Consider thr reaction where Kp=0.497at 500K
PCl5(g)⇔PCl3(g)+Cl2(g)
If the htree gasses are mixed in a right container so that the partial pressure of each gas in initially 1 atm ,then which is correct observation ?
More PCl5 will be produced.
More PCl3 will be produced.
Equilibrium will be established when 50% reaction is complete
None of the above
The correct Answer is:A
To solve the problem step by step, we will analyze the given reaction and the equilibrium constant, and then determine the direction of the reaction based on the initial conditions.
Step 1: Write the Reaction and Given Data
The reaction given is:
PCl5(g)⇌PCl3(g)+Cl2(g)
We are provided with:
- Kp=0.497 at 500K
- Initial partial pressures of each gas: PPCl5=1atm,PPCl3=1atm,PCl2=1atm
Step 2: Calculate the Reaction Quotient Qp
The reaction quotient Qp is calculated using the same expression as Kp, but it is evaluated at the initial conditions:
Qp=PPCl3⋅PCl2PPCl5
Substituting the initial pressures:
Qp=(1atm)⋅(1atm)1atm=1
Step 3: Compare Qp with Kp
Now we compare Qp with Kp:
- Qp=1
- Kp=0.497
Since Qp>Kp, we conclude that the reaction will shift to the left (backward direction) to reach equilibrium.
Step 4: Determine the Direction of the Reaction
When Qp>Kp, the equilibrium shifts toward the reactants. Therefore, more PCl5 will be produced as the reaction proceeds in the backward direction.
Conclusion
The correct observation is that the equilibrium will shift to the left, resulting in the production of more PCl5.
Final Answer
The correct observation is that more PCl5 will be produced.
---
The reaction given is:
PCl5(g)⇌PCl3(g)+Cl2(g)
- Kp=0.497 at 500K
- Initial partial pressures of each gas: PPCl5=1atm,PPCl3=1atm,PCl2=1atm
The reaction quotient Qp is calculated using the same expression as Kp, but it is evaluated at the initial conditions:
Qp=PPCl3⋅PCl2PPCl5
Substituting the initial pressures:
Qp=(1atm)⋅(1atm)1atm=1
Now we compare Qp with Kp:
- Qp=1
- Kp=0.497
When Qp>Kp, the equilibrium shifts toward the reactants. Therefore, more PCl5 will be produced as the reaction proceeds in the backward direction.
The correct observation is that the equilibrium will shift to the left, resulting in the production of more PCl5.
The correct observation is that more PCl5 will be produced.
Topper's Solved these Questions
- CHEMICAL EQUILIBRIUMBOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Illustration
24 Videos
- CHEMICAL EQUILIBRIUMBOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - SOLVED EXAMPLES
20 Videos
- CHEMICAL EQUILIBRIUMBOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - EFFICIENT
50 Videos
- CHEMICAL BONDING-I & IIBOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL BONDING-I & IIEXERCISE - JEE Advanced (Archive)
98 Videos
- CHEMICAL KINETICS BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL KINETICS EXERCISE - JEE Advanced (Archive)
52 Videos
Similar Questions
Knowledge Check
For the reaction,
PCl5(g)⇔PCl3(g)+Cl2(g), the forward reaction at constant temperature is favoured by:Aa. introducing an inert gas at constant volumeBb.introducing Cl2 at constant volumeCc. introducing PCl5 at constant volumeDd. reducing the volume of the container.
For the reaction PCl5(g)⇔PCl3(g)+Cl2(g), the forward reaction at constant temperature favorrd by :
View SolutionConsider the reactions
(i)PCl5(g)⇔PCl3(g)+Cl2(g)
(ii)N2O4(g)⇔2NO2(g)
The addition of an inert gas at constant volume
View SolutionIn the dissociation of PCl5 as
PCl5(g)⇔PCl3(g)+Cl2(g)
If the degree of dissociation is α at equilibrium pressure P, then the equilibrium constant for the reaction is
View SolutionPCl5(g)⇔PCl3(g)+Cl2(g). If 1 mole PCl5 was put in a container of volume V litre and at equlibrium, x moles of it was decomposed, find its KpandKc at equlibrium pressure of P atm.
View SolutionFor the reaction PCl5(g)→PCl3(g)+Cl2(g) where ΔH and ΔE represents enthalpy change and internal energy change respectively.
View SolutionIn the reaction
PCl5(g)⇔PCl3(g)+Cl2(g)
a graph in plotted to show the variation of rate of forward and backward
reactions against time. Which of the following is correct?
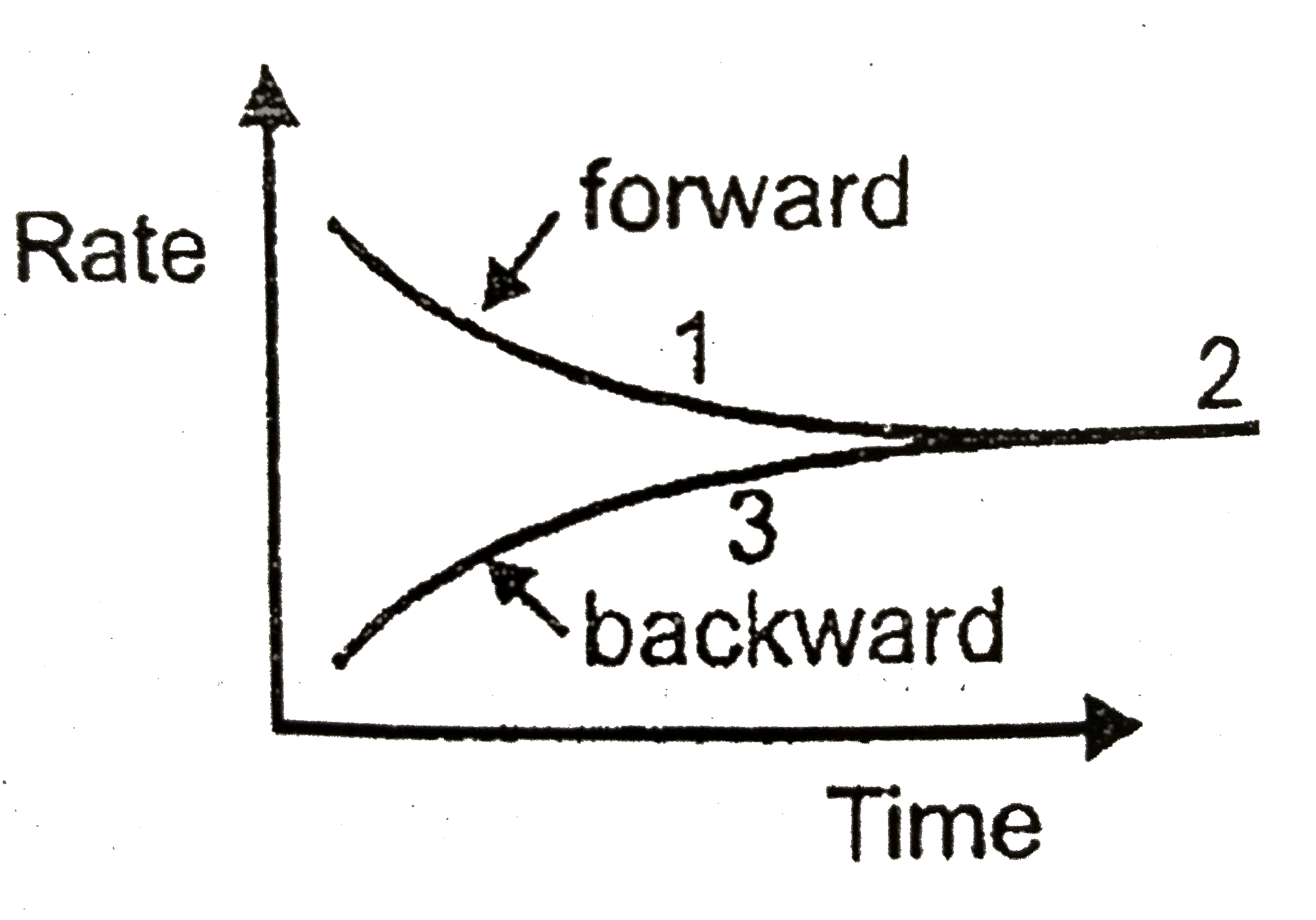
Q>K Q=K Q<K
View SolutionUnit of equilibrium constant Kp for the reaction PCl5(g)⇔PCl3(g)+Cl2(g) is
View Solution
