Close packing is maximum in the crystal which is
Simple cube
bcc
fcc
None of these
The correct Answer is:C
To determine which crystal structure has maximum close packing, we will analyze the packing efficiency of three types of crystal lattices: Simple Cubic (SC), Body-Centered Cubic (BCC), and Face-Centered Cubic (FCC).
1. Understanding Close Packing:
- Close packing refers to the arrangement of atoms in a crystal structure that maximizes the number of atoms in a given volume. This is quantified by the packing fraction, which is the ratio of the volume occupied by the atoms to the total volume of the unit cell.
2. Simple Cubic (SC) Structure:
- In a simple cubic structure, there are 8 atoms located at the corners of the cube.
- Each corner atom contributes 18 of its volume to the unit cell.
- Therefore, the total number of atoms in a simple cubic unit cell is:
Total atoms=8×18=1 atom
- The packing fraction for SC is approximately 52%.
3. Body-Centered Cubic (BCC) Structure:
- In a body-centered cubic structure, there are also 8 corner atoms, contributing 18 each, plus 1 atom at the center of the cube.
- The total number of atoms in a BCC unit cell is:
Total atoms=8×18+1=2 atoms
- The packing fraction for BCC is approximately 68%.
4. Face-Centered Cubic (FCC) Structure:
- In a face-centered cubic structure, there are 8 corner atoms (each contributing 18) and 6 face-centered atoms (each contributing 12).
- The total number of atoms in an FCC unit cell is:
Total atoms=8×18+6×12=1+3=4 atoms
- The packing fraction for FCC is approximately 74%.
5. Comparison of Packing Fractions:
- Simple Cubic: 52%
- Body-Centered Cubic: 68%
- Face-Centered Cubic: 74%
6. Conclusion:
- Among the three crystal structures analyzed, the Face-Centered Cubic (FCC) structure has the highest packing fraction (74%), indicating that it has the maximum close packing.
Final Answer:
The crystal structure with maximum close packing is Face-Centered Cubic (FCC).
- Close packing refers to the arrangement of atoms in a crystal structure that maximizes the number of atoms in a given volume. This is quantified by the packing fraction, which is the ratio of the volume occupied by the atoms to the total volume of the unit cell.
- In a simple cubic structure, there are 8 atoms located at the corners of the cube.
- Each corner atom contributes 18 of its volume to the unit cell.
- Therefore, the total number of atoms in a simple cubic unit cell is:
Total atoms=8×18=1 atom
- The packing fraction for SC is approximately 52%.
- In a body-centered cubic structure, there are also 8 corner atoms, contributing 18 each, plus 1 atom at the center of the cube.
- The total number of atoms in a BCC unit cell is:
Total atoms=8×18+1=2 atoms
- The packing fraction for BCC is approximately 68%.
- In a face-centered cubic structure, there are 8 corner atoms (each contributing 18) and 6 face-centered atoms (each contributing 12).
- The total number of atoms in an FCC unit cell is:
Total atoms=8×18+6×12=1+3=4 atoms
- The packing fraction for FCC is approximately 74%.
- Simple Cubic: 52%
- Body-Centered Cubic: 68%
- Face-Centered Cubic: 74%
- Among the three crystal structures analyzed, the Face-Centered Cubic (FCC) structure has the highest packing fraction (74%), indicating that it has the maximum close packing.
The crystal structure with maximum close packing is Face-Centered Cubic (FCC).
Topper's Solved these Questions
- THE SOLID STATEBOOK - VMC MODULES ENGLISHCHAPTER - THE SOLID STATEEXERCISE - IMPECCABLE
49 Videos
- THE SOLID STATEBOOK - VMC MODULES ENGLISHCHAPTER - THE SOLID STATEEXERCISE - ILLUSTRATIONS
24 Videos
- THE SOLID STATEBOOK - VMC MODULES ENGLISHCHAPTER - THE SOLID STATEEXERCISE - ENABLE
50 Videos
- SURFACE CHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - SURFACE CHEMISTRYEXERCISE - PRACTICE EXERCISE
9 Videos
- THEORY OF SOLUTIONSBOOK - VMC MODULES ENGLISHCHAPTER - THEORY OF SOLUTIONSEXERCISE - JEE Advanced (Archive)
31 Videos
Similar Questions
Knowledge Check
Cubic close packing arrangement is also known as :
AHexagonal close packingBFace centered cubicCBody centered cubicDNone of theseCalculate the efficiency of packing in case of metal crystal for simple cubic (with the assumption that atoms are touching each other.
A0.524B0.624C0.544D0.724
Identical spheres are undergoing two-dimensinal packing in squre close packing and hexagonal close packing. Which is correct regarding the spheres?
View SolutionAssertion: Hexagonal close packing in more light than cubic close packing.
Reason: Hexagonal close packing has C.N of 12 whereas cubic close packing has C.N. of 8.
View SolutionIn which of the following circuits is the current maximum just after the switch S is closed?

View SolutionAssuming that atoms are touching each other, calculate the packing efficiency in case of a crystal of simple cubic metal.
View SolutionThe packing efficiency of a simple cubic crystal with an interstitial atom exactly fitting at the body center is :
View SolutionAssertion In the figure, just after closing the switch the potential drop across inductor is maximum.
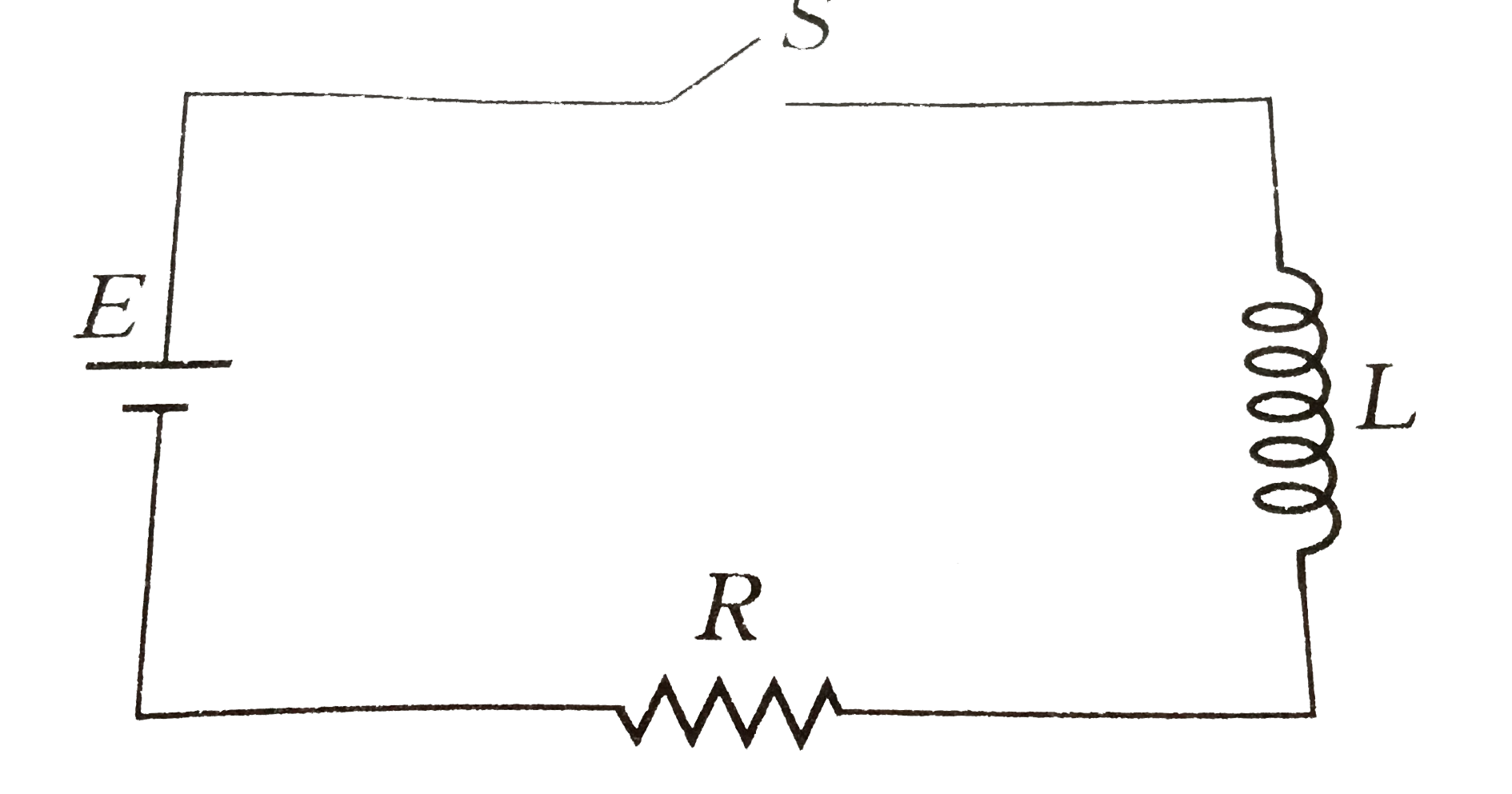
Reason The rate of change of current just after closing the switch is maximum.
View Solution

