Which of these is true regarding an α particle with mass 'm' projected towards the nucleus with velocity 'v ?
When the particle stops at distance of closest approach its kinetic energy converts in to electrostatic potential energy
number of α particles that strike the screen is directly proportional to the square of nuclear charge
higher the kinetic energy of the α particle lesser the deflection
all the above statement are true
The correct Answer is:D
To solve the question regarding the properties of an alpha particle with mass 'm' projected towards a nucleus with velocity 'v', we can analyze the statements one by one.
1. Understanding Kinetic Energy and Potential Energy:
- When an alpha particle is projected towards a nucleus, it possesses kinetic energy given by the formula:
KE=12mv2
- As the alpha particle approaches the nucleus, it experiences an electrostatic force due to the positive charge of the nucleus. As it gets closer, its speed decreases until it comes to a stop at the closest approach distance.
2. Conversion of Energy:
- At the closest approach, the kinetic energy of the alpha particle is completely converted into electrostatic potential energy. The potential energy (PE) between two charged particles can be expressed as:
PE=k⋅q1⋅q2r
- Here, k is Coulomb's constant, q1 and q2 are the charges of the alpha particle and the nucleus, and r is the distance of closest approach.
3. Statement Evaluation:
- Option A: "When the particle stops at a distance of closest approach, its kinetic energy converts to electrostatic potential energy."
- This statement is true as discussed above.
4. Number of Alpha Particles and Nuclear Charge:
- The number of alpha particles that strike a target is proportional to the square of the nuclear charge. This is based on the idea that a higher nuclear charge increases the likelihood of interaction with the alpha particles.
- Option B: "Number of alpha particles that strike the screen is directly proportional to the square of nuclear charge."
- This statement is also true.
5. Kinetic Energy and Deflection:
- According to Rutherford's model, a higher kinetic energy of an alpha particle results in lesser deflection when it interacts with the nucleus. This is because a faster-moving particle has less time to be influenced by the electrostatic force of the nucleus.
- Option C: "Higher the kinetic energy of a particle, lesser the deflection."
- This statement is true as well.
6. Conclusion:
- All three statements regarding the alpha particle are correct. Therefore, the answer to the question is that all the statements are true.
Final Answer:
All the statements regarding the alpha particle are true.
- When an alpha particle is projected towards a nucleus, it possesses kinetic energy given by the formula:
KE=12mv2
- As the alpha particle approaches the nucleus, it experiences an electrostatic force due to the positive charge of the nucleus. As it gets closer, its speed decreases until it comes to a stop at the closest approach distance.
- At the closest approach, the kinetic energy of the alpha particle is completely converted into electrostatic potential energy. The potential energy (PE) between two charged particles can be expressed as:
PE=k⋅q1⋅q2r
- Here, k is Coulomb's constant, q1 and q2 are the charges of the alpha particle and the nucleus, and r is the distance of closest approach.
- Option A: "When the particle stops at a distance of closest approach, its kinetic energy converts to electrostatic potential energy."
- This statement is true as discussed above.
- The number of alpha particles that strike a target is proportional to the square of the nuclear charge. This is based on the idea that a higher nuclear charge increases the likelihood of interaction with the alpha particles.
- Option B: "Number of alpha particles that strike the screen is directly proportional to the square of nuclear charge."
- This statement is also true.
- According to Rutherford's model, a higher kinetic energy of an alpha particle results in lesser deflection when it interacts with the nucleus. This is because a faster-moving particle has less time to be influenced by the electrostatic force of the nucleus.
- Option C: "Higher the kinetic energy of a particle, lesser the deflection."
- This statement is true as well.
- All three statements regarding the alpha particle are correct. Therefore, the answer to the question is that all the statements are true.
All the statements regarding the alpha particle are true.
Topper's Solved these Questions
- STRUCTURE OF ATOMBOOK - VMC MODULES ENGLISHCHAPTER - STRUCTURE OF ATOMEXERCISE - IMPECCABLE
49 Videos
- STRUCTURE OF ATOMBOOK - VMC MODULES ENGLISHCHAPTER - STRUCTURE OF ATOMEXERCISE - Illustration
29 Videos
- STRUCTURE OF ATOMBOOK - VMC MODULES ENGLISHCHAPTER - STRUCTURE OF ATOMEXERCISE - ENABLE
50 Videos
- STOICHIOMETRY-IIBOOK - VMC MODULES ENGLISHCHAPTER - STOICHIOMETRY-IIEXERCISE - JEE Advanced (Archive)
43 Videos
- SURFACE CHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - SURFACE CHEMISTRYEXERCISE - PRACTICE EXERCISE
9 Videos
Similar Questions
A particle of mass m carrying charge q is projected with velocity v from point P toward aninfinite line of charge from a distance a . Its speed reduces to zero momentarily at point Q, which is at a distance a/2 from the line of charge. If another particle with mass m and charge −q is projected with the same velosity v from P toward the line of charge, what will be its speed at Q ?
View SolutionA satellite orbiting close to the surface of earth does not fall down because the gravitational pull of earth :
View Solution
Knowledge Check
The escape velocity of a particle of mass 'm'
Avaries as m2Bvaries as mCvaries as m−1Dis independent of mass
Two spheres of masses mand2m are separated by distance d. A particle of mass m5 is projected straight from 2m towards m with a velocity v0. Which of the following statements is correct ?
View SolutionConsider the situation described in the previous example. A particle of mass m having a charge q is placed at a distance d from the metal sheet and is projected towards it. Find the maximum velocity of projection for which the particle does not hit the sheet.
View SolutionRutherford model: The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α-scattering experiment. If an α-particle is projected from infinity with speed v towards the nucleus having Z protons, then the α-particle which is reflected back or which is deflected by 180∘ must have approached closest to the nucleus .It can be approximated that α particle collides with the nucleus and gets back. Now if we apply the energy conservation equation at initial point and collision point then:
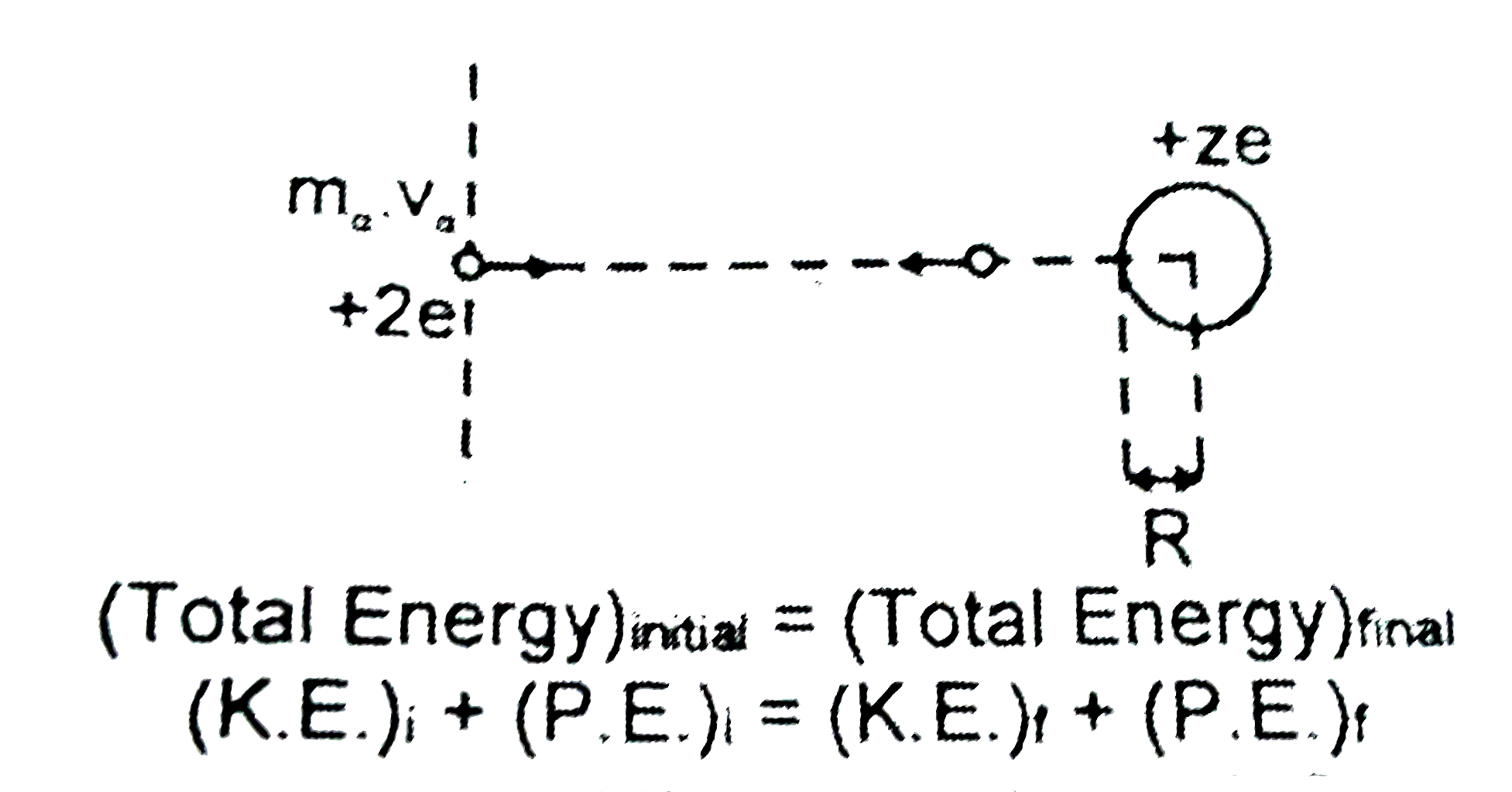
(P.E.)i=0, since P.E. of two charge system separated by infinite distance is zero. Finally the particle stops and then starts coming back.
12mαv2α+0=0+Kq1q2R⇒12mαv2α=K2e×ZeR⇒R=4KZe2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it
An α-particle with initial speed v0 is projected from infinity and it approaches up to r0 distance from a nuclie. Then, the initial speed of α-particle, which approaches upto 2r0 distance from the nucleus is :
View SolutionA uniform magnetic field ¯¯¯B=B0ˆj exists in a space. A particle of mass m and charge q is projected towards negative x-axis with speed v from the a point (d,0,0) The maximum value v for which the particle does not hit y−z plane is .
View SolutionRutherford model: The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α-scattering experiment. If an α-particle is projected from infinity with speed v towards the nucleus having Z protons, then the α-particle which is reflected back or which is deflected by 180∘ must have approached closest to the nucleus .It can be approximated that α particle collides with the nucleus and gets back. Now if we apply the energy conservation equation at initial point and collision point then:
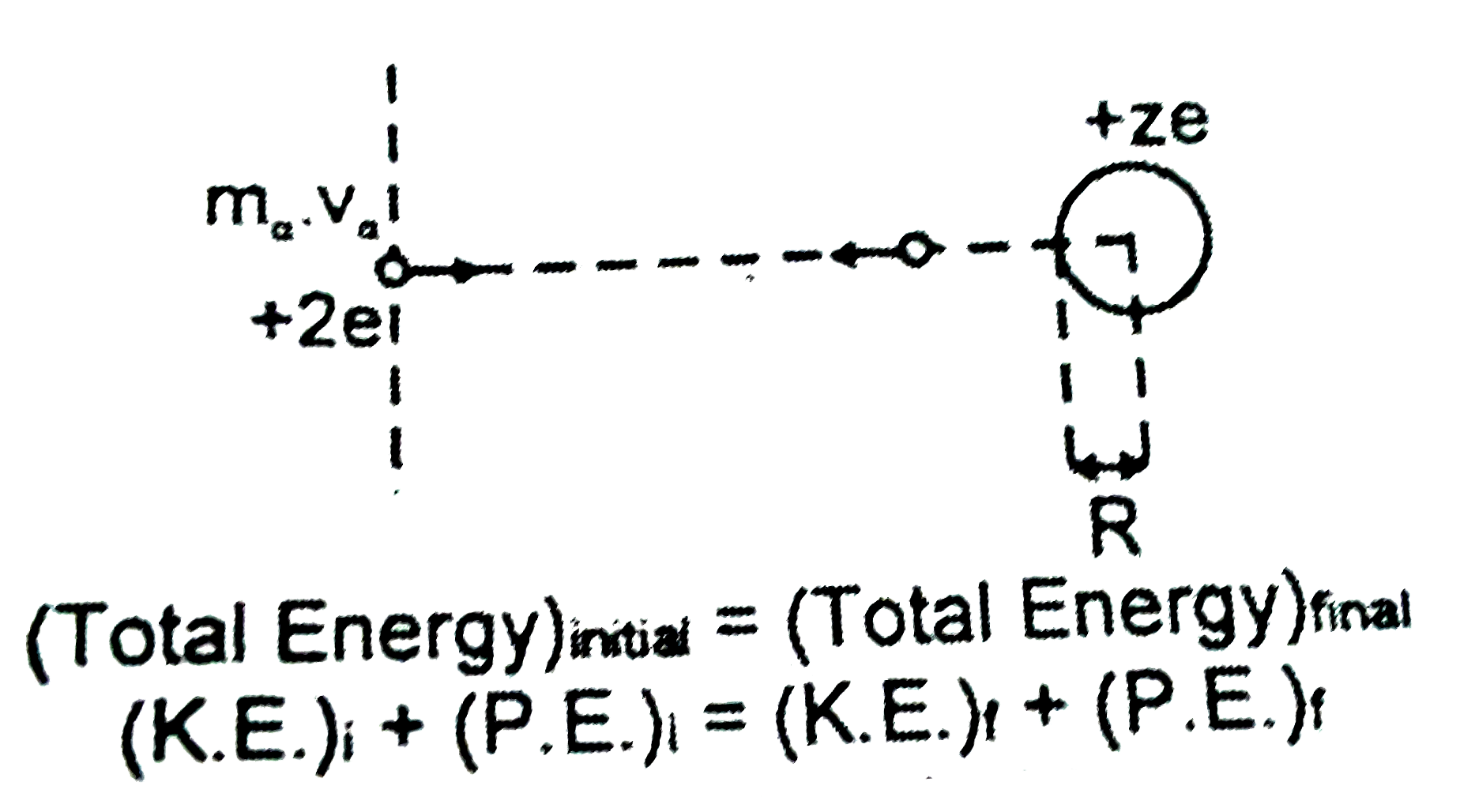
(P.E.)i=0, since P.E. of two charge system separated by infinite distance is zero. Finally the particle stops and then starts coming back.
12mαv2α+0=0+Kq1q2R⇒12mαv2α=K2e×ZeR⇒R=4KZe2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it
Radius of a particular nucleus is calculated by the projection of α-particle from infinity at a particular speed. Let this radius is the true radius . If the radius calculation for the same nucleus is made by another α-particle with half of the earlier speed, then the percentage error involved in the radius calculation is :
View SolutionA nucleus .mnX emits one α−particle and two β−particles. The resulting nucleus is
View SolutionWhen an α−particle of mass 'm' moving with velocity 'v' bombards on a heavy nucleus of charge 'Ze' its distance of closest approach from the nucleus depends on m as :
View Solution
