Sulphur exists in more than one solid form. The stable form at room temperature is rhombic sulphur. But above room temperature the following reaction occurs :
S (rhombic) → S (monoclinic)
If ΔH=276.144 J at 298 K and 1 atm and ΔG=75.312J
Assume that ΔH and ΔS do not vary significantly with temperature, calculate Teq, the temperature at which rhombic and monoclinic sulphur exist in equilibrium with each other.
To find the equilibrium temperature Teq at which rhombic and monoclinic sulfur exist in equilibrium, we can use the Gibbs free energy equation under equilibrium conditions.
1. Understanding the Gibbs Free Energy Equation:
At equilibrium, the change in Gibbs free energy (ΔG) is zero. The relationship between ΔG, ΔH (enthalpy change), and ΔS (entropy change) is given by:
ΔG=ΔH−TΔS
At equilibrium, we set ΔG=0:
0=ΔH−TeqΔS
2. Rearranging the Equation:
Rearranging the equation gives us:
Teq=ΔHΔS
3. Finding ΔS:
We know that:
ΔG=ΔH−TΔS
Rearranging this for ΔS gives:
ΔS=ΔH−ΔGT
Substituting the known values:
- ΔH=276.144J
- ΔG=75.312J
- T=298K
We can calculate ΔS:
ΔS=276.144−75.312298
ΔS=200.832298≈0.674J/K
4. Calculating Teq:
Now, we can substitute ΔH and ΔS back into the equation for Teq:
Teq=276.1440.674
Teq≈409.7K
Final Answer:
The temperature at which rhombic and monoclinic sulfur exist in equilibrium is approximately 409.7K.
---
At equilibrium, the change in Gibbs free energy (ΔG) is zero. The relationship between ΔG, ΔH (enthalpy change), and ΔS (entropy change) is given by:
ΔG=ΔH−TΔS
At equilibrium, we set ΔG=0:
0=ΔH−TeqΔS
Rearranging the equation gives us:
Teq=ΔHΔS
We know that:
ΔG=ΔH−TΔS
Rearranging this for ΔS gives:
ΔS=ΔH−ΔGT
Substituting the known values:
- ΔH=276.144J
- ΔG=75.312J
- T=298K
ΔS=276.144−75.312298
ΔS=200.832298≈0.674J/K
Now, we can substitute ΔH and ΔS back into the equation for Teq:
Teq=276.1440.674
Teq≈409.7K
The temperature at which rhombic and monoclinic sulfur exist in equilibrium is approximately 409.7K.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL THERMODYNAMICS EXERCISE - SOLVED EXAMPLES19videos
CHEMICAL THERMODYNAMICS
- BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL THERMODYNAMICS EXERCISE - PRACTICE EXERCISE22videos
CHEMICAL THERMODYNAMICS
- BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL KINETICS EXERCISE - JEE Advanced (Archive)52videos
CHEMICAL KINETICS
- BOOK - VMC MODULES ENGLISHCHAPTER - CLASSIFICATION OF ELEMENTS AND PERIODICITY IN PROPERTIES EXERCISE - JEE ADVANCE (ARCHIVE)30videos
CLASSIFICATION OF ELEMENTS AND PERIODICITY IN PROPERTIES
Similar Questions
Sulphur exists is more than one solid form. The stable form at room temperature is rhombic sulphur. But above room temperature the following reaction occurs.
S(rhomic)→S(monoclinic)
If ΔHΘ=−276.144Jat298K and 1atm and ΔGΘ=75.312J
a. Calculate ΔSΘ at 298K.View SolutionSulphur exists in more than one solid form. The stable form at room temperature is rhombic sulphur. But above room temperature the following reaction occurs.
S(rhombic)→S(monoclinic)
If ΔHΘ=276.144Jat298K and 1atm and ΔGΘ=75.312J
a. Calculate ΔSΘ at 298K.
b. Assume that ΔHΘ and ΔSΘ do not vary significantly with temperature, calculate Teq, the temperature at which rhombic and monoclinic sulphur exist in equilibrium with each other.View Solution
Knowledge Check
- Question 1 - Select One
For a given reaction, ΔH=35.5kJmol−1 and ΔS=83.6JK−1mol−1. The reaction is spontaneous at (Assume that ΔHandΔS do not vary with temperature)
AT lt 425 KBTgt 425 KCAll temperaturesDTgt 298 K
Rhombic and monoclinic sulphur are _____.
View SolutionMost stable allotropic form of sulphur is rhombic sulphur. Explain
View SolutionWhich allotropic form of sulphur is most stable at room temperature?
View SolutionWhich allotropic form of sulphur is thermodynamically stable at room temperature ?
View SolutionFor a given reaction, ΔH=35.5kJmol−1 and ΔS=83.6JK−1mol(−1). The reaction is spontaneous at : (Assume that ΔHandΔS do not vary with temperature)
View SolutionWhich of the following reactions is ΔH less than ΔE ?
View SolutionAssuming ΔH0andΔS0 do not change with temperature the boiling point of liquid ''A'' (the thermodynamics data given below) is
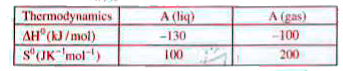 View Solution
View Solution

