Compute the Gibbs energy change of the reaction at 27∘C for the combustion of methane.
CH4(g)+2O2(g)→CO2(g)+2H2O(l)ΔfHΘ(in kh mol1) −74.8 − −393.5 −285.8SΘm(in J K^(-1)mol−1) 186 205 214 70
To compute the Gibbs energy change (ΔG°) for the combustion of methane at 27°C, we will follow these steps:
Step 1: Write the Reaction
The balanced chemical reaction for the combustion of methane is:
CH4(g)+2O2(g)→CO2(g)+2H2O(l)
Step 2: Gather Data
From the problem, we have the following data:
- Standard enthalpy of formation (ΔfH° in kJ/mol):
- CH₄(g): -74.8 kJ/mol
- O₂(g): 0 kJ/mol (as it is a standard state)
- CO₂(g): -393.5 kJ/mol
- H₂O(l): -285.8 kJ/mol
- Standard entropy (S° in J/K·mol):
- CH₄(g): 186 J/K·mol
- O₂(g): 205 J/K·mol
- CO₂(g): 214 J/K·mol
- H₂O(l): 70 J/K·mol
Step 3: Calculate Standard Enthalpy Change (ΔH°)
The standard enthalpy change for the reaction can be calculated using the formula:
ΔH°=∑(ΔfH° of products)−∑(ΔfH° of reactants)
Calculating:
ΔH°=((−393.5)+2×(−285.8))−((−74.8)+2×(0))
=(−393.5−571.6)−(−74.8)
=−965.1+74.8=−890.3 kJ/mol
Step 4: Calculate Standard Entropy Change (ΔS°)
The standard entropy change for the reaction can be calculated using the formula:
ΔS°=∑(S° of products)−∑(S° of reactants)
Calculating:
ΔS°=((214)+2×(70))−((186)+2×(205))
=(214+140)−(186+410)
=354−596=−242 J/K⋅mol
Step 5: Convert ΔS° to kJ
To convert ΔS° from J/K·mol to kJ/K·mol, we divide by 1000:
ΔS°=−242 J/K⋅mol÷1000=−0.242 kJ/K⋅mol
Step 6: Calculate Gibbs Energy Change (ΔG°)
Using the Gibbs free energy equation:
ΔG°=ΔH°−TΔS°
Where T is in Kelvin. Convert 27°C to Kelvin:
T=27+273=300 K
Now substituting the values:
ΔG°=−890.3 kJ/mol−(300 K×−0.242 kJ/K⋅mol)
=−890.3+72.6=−817.7 kJ/mol
Final Answer
The Gibbs energy change for the combustion of methane at 27°C is approximately:
ΔG°≈−817.7 kJ/mol
The balanced chemical reaction for the combustion of methane is:
CH4(g)+2O2(g)→CO2(g)+2H2O(l)
From the problem, we have the following data:
- Standard enthalpy of formation (ΔfH° in kJ/mol):
- CH₄(g): -74.8 kJ/mol
- O₂(g): 0 kJ/mol (as it is a standard state)
- CO₂(g): -393.5 kJ/mol
- H₂O(l): -285.8 kJ/mol
- CH₄(g): 186 J/K·mol
- O₂(g): 205 J/K·mol
- CO₂(g): 214 J/K·mol
- H₂O(l): 70 J/K·mol
The standard enthalpy change for the reaction can be calculated using the formula:
ΔH°=∑(ΔfH° of products)−∑(ΔfH° of reactants)
ΔH°=((−393.5)+2×(−285.8))−((−74.8)+2×(0))
=(−393.5−571.6)−(−74.8)
=−965.1+74.8=−890.3 kJ/mol
The standard entropy change for the reaction can be calculated using the formula:
ΔS°=∑(S° of products)−∑(S° of reactants)
ΔS°=((214)+2×(70))−((186)+2×(205))
=(214+140)−(186+410)
=354−596=−242 J/K⋅mol
To convert ΔS° from J/K·mol to kJ/K·mol, we divide by 1000:
ΔS°=−242 J/K⋅mol÷1000=−0.242 kJ/K⋅mol
Using the Gibbs free energy equation:
ΔG°=ΔH°−TΔS°
T=27+273=300 K
ΔG°=−890.3 kJ/mol−(300 K×−0.242 kJ/K⋅mol)
=−890.3+72.6=−817.7 kJ/mol
The Gibbs energy change for the combustion of methane at 27°C is approximately:
ΔG°≈−817.7 kJ/mol
Topper's Solved these Questions
- CHEMICAL THERMODYNAMICS BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL THERMODYNAMICS EXERCISE - SOLVED EXAMPLES
19 Videos
- CHEMICAL THERMODYNAMICS BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL THERMODYNAMICS EXERCISE - PRACTICE EXERCISE
22 Videos
- CHEMICAL KINETICS BOOK - VMC MODULES ENGLISHCHAPTER - CHEMICAL KINETICS EXERCISE - JEE Advanced (Archive)
52 Videos
- CLASSIFICATION OF ELEMENTS AND PERIODICITY IN PROPERTIES BOOK - VMC MODULES ENGLISHCHAPTER - CLASSIFICATION OF ELEMENTS AND PERIODICITY IN PROPERTIES EXERCISE - JEE ADVANCE (ARCHIVE)
30 Videos
Similar Questions
Compute the standard free enegry of the reaction at 27∘C for the combustion fo methane using the give data:
CH4(g)+2O2(g)→CO2(g)+2H2O(l)
SpeciesCH4O2CO22H2O(l)ΔfHΘ(kJmol−1)−74.8−−393.5−285.8SΘ(JK−1mol−1)18620521470
View SolutionAt 298K, the enthalpy of combustion of CH4 corresponds to the reaction
CH4(g)+2O2(g)→CO2(g)+2H2O(g)
View SolutionCompute the standard free energy of the reaction at 27∘C for the combustion of methane
CH4(g)+2O2(g)→CO2(g)+2H2O(l)
using the following data :
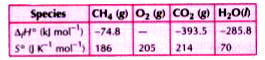
Also comment on the feasibility of the reaction.
View SolutionSelect correct statement(s) for the reaction H2O(g)+CO(g)→H2(g)+CO2(g) substance
CO(g) CO2(g) H2O(g) H2(g)
ΔfH∘400(kcal mol−1) -25 -95 -55 0
S∘400(cal mol−1K−1) 45 50 40 30
View SolutionCalculate the heat of combustion of eithene
CH2=CH2(g)+3O2(g)→2CO2(g)+2H2O(I)
The bond energy data are given below
C = C = 619 kJ mol−1
C - H = 414 kJ mol−1
O = O = 499 kJ mol−1
C = O = 724 kJ mol−1
O - H = 460 kJ mol−1
View SolutionCalculate the heat of combustion of eithene
CH2=CH2(g)+3O2(g)→2CO2(g)+2H2O(I)
The bond energy data are given below
C = C = 619 kJ mol−1
C - H = 414 kJ mol−1
O = O = 499 kJ mol−1
C = O = 724 kJ mol−1
O - H = 460 kJ mol−1
View SolutionFor the reaction : C2H5OH(l)+3O2(g)→2CO2(g)+3H2O(g)
if ΔU∘=−1373kJmol−1 at 298K. Calculate ΔH∘
View SolutionCalculate the standard free energy change for the formation of methane at 300K:
C(graphite)+2H2(g)→CH4(g)
The following data are given:
ΔfHΘ(kJmol−1):CH4(g)=−74.81
ΔfSΘ(JK−1mol−1):C(graphite)=5.70,H2(g)=130.7CH4(g)=186.3
View SolutionThe internal energy change (ΔU) for the reaction CH4(g)+2O2(g)→CO2(g)+2H2O(l) is −885kJmol−1 at 298K. What is ΔH at 398 K?
View SolutionFor the equilibrium reaction:
2H2(g)+O2(g)⇔2H2O(l)at298K
ΔGΘ=−474.78kJmol−1. Calculate log K for it.
(R=8.314JK−1mol−1).
View Solution
