If an atom is present in the centre of the cube, the participation of that atom per unit cell is:
14
1
12
18
The correct Answer is:B
To determine the participation of an atom located at the center of a cube (body-centered cubic structure) per unit cell, we can follow these steps:
Step 1: Understand the Structure
In a body-centered cubic (BCC) structure, there is one atom located at the center of the cube and additional atoms located at the corners of the cube.
Step 2: Contribution of the Atom at the Center
The atom at the center of the cube is fully contained within that unit cell. Therefore, its contribution to the unit cell is considered as 1 whole atom.
Step 3: Contribution of Corner Atoms
For completeness, we can also consider the contribution of the corner atoms. Each corner atom is shared among eight adjacent unit cells, contributing only 1/8 of an atom to each unit cell. Since there are 8 corners in a cube, the total contribution from the corner atoms would be:
Contribution from corners=8×18=1 atom
Step 4: Total Contribution per Unit Cell
In a body-centered cubic unit cell, the total number of atoms per unit cell is:
- 1 atom from the center
- 1 atom from the corners
Thus, the total number of atoms per unit cell in a BCC structure is:
Total atoms per unit cell=1+1=2 atoms
Step 5: Final Answer
However, the question specifically asks for the participation of the atom at the center of the cube per unit cell, which is simply:
Participation of the atom at the center=1
Therefore, the correct answer is:
Option 2: 1
---
In a body-centered cubic (BCC) structure, there is one atom located at the center of the cube and additional atoms located at the corners of the cube.
The atom at the center of the cube is fully contained within that unit cell. Therefore, its contribution to the unit cell is considered as 1 whole atom.
For completeness, we can also consider the contribution of the corner atoms. Each corner atom is shared among eight adjacent unit cells, contributing only 1/8 of an atom to each unit cell. Since there are 8 corners in a cube, the total contribution from the corner atoms would be:
Contribution from corners=8×18=1 atom
In a body-centered cubic unit cell, the total number of atoms per unit cell is:
- 1 atom from the center
- 1 atom from the corners
Total atoms per unit cell=1+1=2 atoms
However, the question specifically asks for the participation of the atom at the center of the cube per unit cell, which is simply:
Participation of the atom at the center=1
Option 2: 1
Topper's Solved these Questions
- THE SOLID STATEBOOK - VMC MODULES ENGLISHCHAPTER - THE SOLID STATEEXERCISE - EXERCISE-H
10 Videos
- THE SOLID STATEBOOK - VMC MODULES ENGLISHCHAPTER - THE SOLID STATEEXERCISE - EXERCISE-I
10 Videos
- THE SOLID STATEBOOK - VMC MODULES ENGLISHCHAPTER - THE SOLID STATEEXERCISE - EXERCISE-F
10 Videos
- SURFACE CHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - SURFACE CHEMISTRYEXERCISE - PRACTICE EXERCISE
9 Videos
- THEORY OF SOLUTIONSBOOK - VMC MODULES ENGLISHCHAPTER - THEORY OF SOLUTIONSEXERCISE - JEE Advanced (Archive)
31 Videos
Similar Questions
A cubic solid has two different atoms P & Q. Atoms P are present at comers and Q at the centre of the cube. What is the formal of unit cell ?
View Solutionpacking fraction of a unit cell is drfined as the fraction of the total volume of the unit cell occupied by the atom(s).
P.E=Volume of the atoms(s) present in a unit cellVolume of unit cell=Z×43πr3a3
and % of empty space = 100−P.F.×100
where Z= effective number of stoms in s cube .
r= radius of a an atoms
a = edge lenght of the cube
Packing fraction in face centered cubic unit cell is :
View SolutionThree atoms A, B and C crystallize in a cubic solid lattice where A atoms are present at the body centre, B atoms are present at the edge centre as well as at the corners of the cube and C atoms are present at the face centres of the cube. Now if all the atoms are removed from the two 4-fold axis and the one 2-fold axis passing through the cube, then the formula of the compound is
View SolutionA cubic solid is made up of two atoms A and B. Atoms A are present at the corners and B at the centre of the body.
What is the formula of the unit cell ?
View SolutionIn a face centred cubic (f.c.c.) arrangement, the number of atoms per unit cell is
View SolutionIn sc, bcc and fcc the ratio of number of atoms per unit cell is given by
View SolutionA unit cell consists of a cube in which X atoms at the corners and Y atoms are at the face centres. If two atoms are missing from two corners of the unit cell , what is the formula of the compound ?
View SolutionIf three elements P, Q and R crystallise in a cubic unit cell with P atoms at the corners, Q atoms at the cubic centre and R atoms at the centre of each face of the cube, then write the formula of the compound.
View SolutionIf three elements P, Q and R crystallise in a cubic unit cell with P atoms at the corners, Q atoms at the cubic centre and R atoms at the centre of each face of the cube, then write the formula of the compound.
View SolutionThe figure below shows a unit cell of the mineral Perovskite ( the titanium atom is at the centre of the cube ). Which of the following is a correct chemical formula for this mineral ?
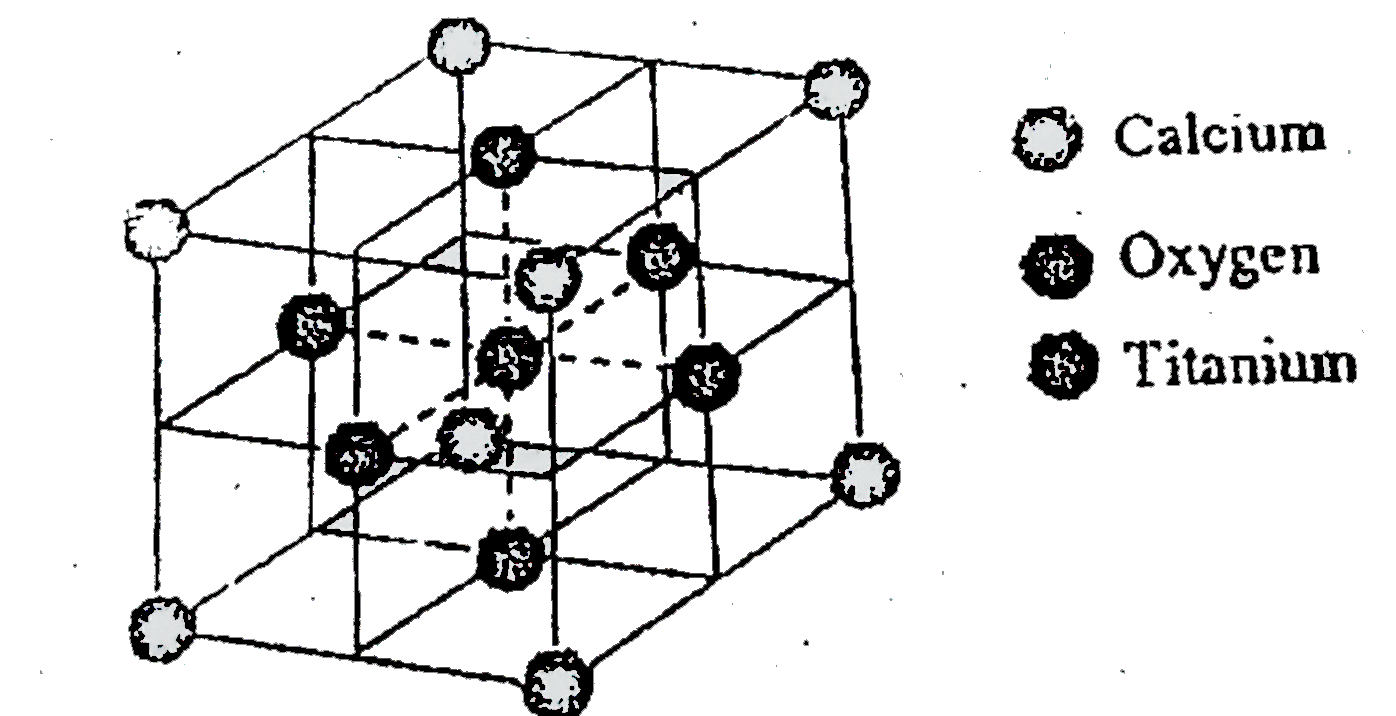
View Solution
