A long solenoid has a radius a and number of turns per unit length is n. If it carries a current i, then the magnetic field on its axis is directly proportional to
ani
ni
nia
n2i
The correct Answer is:2
To solve the problem, we need to determine how the magnetic field B on the axis of a long solenoid is related to its parameters: the number of turns per unit length n and the current i flowing through it.
1. Understanding the Solenoid:
A long solenoid is a coil of wire that is tightly wound in a helical shape. The magnetic field inside a long solenoid is uniform and parallel to the axis of the solenoid.
2. Formula for Magnetic Field:
The magnetic field B inside a long solenoid is given by the formula:
B=μ0ni
where:
- B is the magnetic field,
- μ0 is the permeability of free space (a constant),
- n is the number of turns per unit length of the solenoid,
- i is the current flowing through the solenoid.
3. Identifying Proportionality:
From the formula B=μ0ni, we can see that the magnetic field B is directly proportional to both n and i. This means:
B∝nandB∝i
Therefore, we can conclude:
B∝ni
4. Conclusion:
The magnetic field on the axis of the solenoid is directly proportional to the product of the number of turns per unit length n and the current i.
Final Answer:
The magnetic field on the axis of the solenoid is directly proportional to ni.
---
A long solenoid is a coil of wire that is tightly wound in a helical shape. The magnetic field inside a long solenoid is uniform and parallel to the axis of the solenoid.
The magnetic field B inside a long solenoid is given by the formula:
B=μ0ni
where:
- B is the magnetic field,
- μ0 is the permeability of free space (a constant),
- n is the number of turns per unit length of the solenoid,
- i is the current flowing through the solenoid.
From the formula B=μ0ni, we can see that the magnetic field B is directly proportional to both n and i. This means:
B∝nandB∝i
Therefore, we can conclude:
B∝ni
The magnetic field on the axis of the solenoid is directly proportional to the product of the number of turns per unit length n and the current i.
The magnetic field on the axis of the solenoid is directly proportional to ni.
Topper's Solved these Questions
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-D
12 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-E
10 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-B
10 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - MCQ
2 Videos
- PROPERTIES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced (Archive) Level - 2 (MATRIX MATCH TYPE)
1 Videos
Similar Questions
A tightly wound solenoid of radius 'a' and length 'l' has n turns per unit length. It carries an electric current i. Magnetic field at a distance l4 from one of the end (inside the solenoid on its axis) is B=μ0ni(√5+3)√K for l=4a. Then find the value of K.
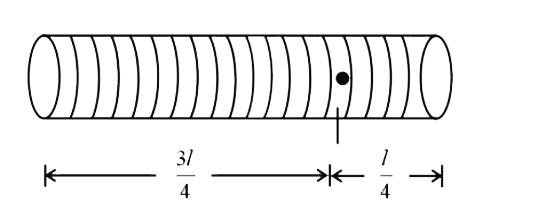
View SolutionA tightly wound solenoid of radius 'a' and length 'l' has n turns per unit length. It carries an electric current i. Magnetic field at a distance l4 from one of the end (inside the solenoid on its axis) is B=μ0ni(√5+3)√K for l=4a. Then find the value of K.

View Solution
Knowledge Check
A solenoid of length 0.6 m has a radius of 2 cm and is made up of 600 turns If it carries a current of 4 A, then the magnitude of the magnetic field inside the solenoid is
A6.024×10−3TB8.024×10−3TC5.024×10−3TD7.024×10−3T
A thin solenoid of length 0.4m and having 500 turns of wire carries a current 1A,then find the magnetic field on the axis inside the solenoid.
View SolutionA long solenoid of length L has a mean diameter D. It has n layers of windings of N turns each. If it carries a current ‘i’ the magnetic field at its centre will be
View SolutionA wire is wound on a long rod of material of relative permeability μr=4000 to make a solenoid. If the current through the wire is 5 A and number of turns per unit length is 1000 per metre, then the magnetic field inside the solenoid is
View SolutionA tightly- wound solenoid of radius a and length l has n turns per unit length. It carries an electric current i. Consider a length dx of the solenoid at a distance x from one end. This contains n dx turns and may be approximated as a circular current I n dx. (a) Write the magnetic field at the center of the solenoid due to this circular current. Integrate this expression under proper limits to find the magnetic field at the center of the solenoid. (b) Verify that if l>>a, the field tends to (B=(μ0ni) and if a>>1, the field tends toB=((μ0)nil2a) . Interpret these results.
View SolutionA tightly-wound, long solenoid has n turns per unit length, a radius r and carries a current i. A particle having charge q and mass m is projected from a point on the axis in a direction perpendicular to the axis. What can be the maximum speed for which the particle does not strike the solenoid?
View SolutionA tightly-wound, long solenoid has n turns per unit length, a radius r and carries a current i. A particle having charge q and mass m is projected from a point on the axis in a direction perpendicular to the axis. What can be the maximum speed for which the particle does not strike the solenoid?
View SolutionA 25cm long solenoid has radius 2cm and 500 total number of turns. It carries as current of 15A.If it is equivalent to a magnet of the same size and magnetization ¯¯¯¯M (magnetic moment//volume) then ∣∣¯¯¯¯M∣∣ is:
View Solution
