A certain amount of current when flowing in a properly set tangent galvanoment, produces a deflection of 45∘. If the current be reduced by a factor of √3, the deflection would
decrease by 30∘.
decrease by 15∘.
increase by 15∘.
increase by 30∘.
The correct Answer is:2
To solve the problem, we will follow these steps:
Step 1: Understand the relationship between current and deflection
In a tangent galvanometer, the current (I) flowing through it is directly proportional to the tangent of the angle of deflection (θ). Mathematically, this can be expressed as:
I∝tan(θ)
Step 2: Define the initial conditions
Let the initial current be I1 and the initial deflection be θ1=45∘.
Step 3: Determine the new current
The current is reduced by a factor of √3:
I2=I1√3
Step 4: Set up the ratio of currents and tangents of angles
Using the proportionality established earlier, we can write:
I2I1=tan(θ2)tan(θ1)
Step 5: Substitute the known values
Substituting I2 and θ1 into the equation:
I1√3I1=tan(θ2)tan(45∘)
Since tan(45∘)=1, we have:
1√3=tan(θ2)
Step 6: Solve for the new angle of deflection
To find θ2, we take the inverse tangent:
θ2=tan−1(1√3)
Step 7: Calculate θ2
The angle whose tangent is 1√3 is:
θ2=30∘
Step 8: Determine the change in deflection
Now we find the change in deflection:
Change=θ1−θ2=45∘−30∘=15∘
Conclusion
The deflection decreases by 15∘.
Final Answer
The deflection would decrease by 15 degrees.
---
In a tangent galvanometer, the current (I) flowing through it is directly proportional to the tangent of the angle of deflection (θ). Mathematically, this can be expressed as:
I∝tan(θ)
Let the initial current be I1 and the initial deflection be θ1=45∘.
The current is reduced by a factor of √3:
I2=I1√3
Using the proportionality established earlier, we can write:
I2I1=tan(θ2)tan(θ1)
Substituting I2 and θ1 into the equation:
I1√3I1=tan(θ2)tan(45∘)
Since tan(45∘)=1, we have:
1√3=tan(θ2)
To find θ2, we take the inverse tangent:
θ2=tan−1(1√3)
The angle whose tangent is 1√3 is:
θ2=30∘
Now we find the change in deflection:
Change=θ1−θ2=45∘−30∘=15∘
The deflection decreases by 15∘.
The deflection would decrease by 15 degrees.
Topper's Solved these Questions
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-I
10 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-J
10 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-G
10 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - MCQ
2 Videos
- PROPERTIES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced (Archive) Level - 2 (MATRIX MATCH TYPE)
1 Videos
Similar Questions
When 2 amperes current is passed through a tangent galvanometer, it gives a deflection of 30∘. For 60∘ deflection, the current must be
View SolutionWhen a current of 1A passes through a tangent galvanometer, angle of defection is deg 60. When current is reduced to 0.5A, then the new angle of deflection will be
View SolutionIf in a moving coil galvanometer, a current I produces a deflection θ, then
View SolutionA current or 0.5 A produces a deflection of 60° in a tangent galvanometer. The cunent that produces a deflection of 30° in the same galvanometer is
View SolutionThe current that must flow through the coil of a galvanometre so as to produce a deflection of one division on its scale is called
View SolutionA leaf electroscope is a simple apparatus to detect any charge on a body. It consist of two metal leaves OA and OB, free to rotate about O. Initially both are very slighty separted. When a charged object is touched to the metal knob at the top of the conduction re, charge flows from knob to the top of the conduction rod, charge flows from knob to the leaves through the conducting rod, charge flows from knob to the leaves through the conduction rod. As the leaves are now charged similarly, they start repelling each other and tet separted (deflected by certain angle).
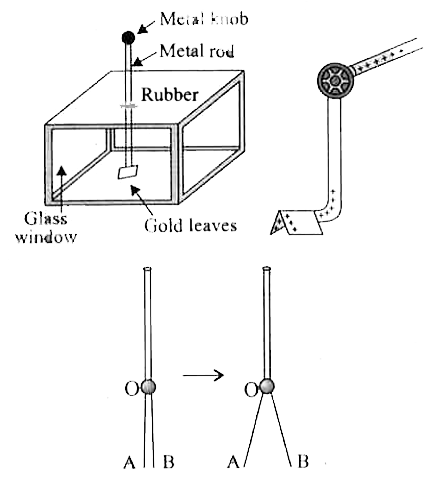
The angle of deflection in static equilibrium is an indicator of the amount of charge on the charged boy.
When a+20C rod is touched to the knob, the deflection of leaves was 5∘, and when an identical rod of −40C is touched, the deflection was found to be 9∘. If an identical rod of +30C is touched, then the deflection may be:
View SolutionIn a tangent galvanometer, when a current of 10mA is passed, the deflection is 31∘. By what percentage, the current has to be increased, so at to produce a deflection of 42∘?
View SolutionWhen a current is passed in moving coil galvanometer, the coil gets deflected because,
View SolutionIn a circuit for finding the resistance of a galvanometer by half deflection method, a 6 V battery and a high resistance of 11 kΩ are used. The figure of merit of the galvanometer is 60 μA/division. In the absence of shunt resistance, the galvanometer produces a deflection of θ=9 divisions when current flows in the circuit. The value of the shunt resistance that can cause the deflection of θ/2, is closet to :
View SolutionTwo tangent galvanometers having coils of the same radius are connected in series. A current flowing in them produces deflections of 60∘ and 45∘ respectively. The ratio of the number of turns in the coils is
View Solution
