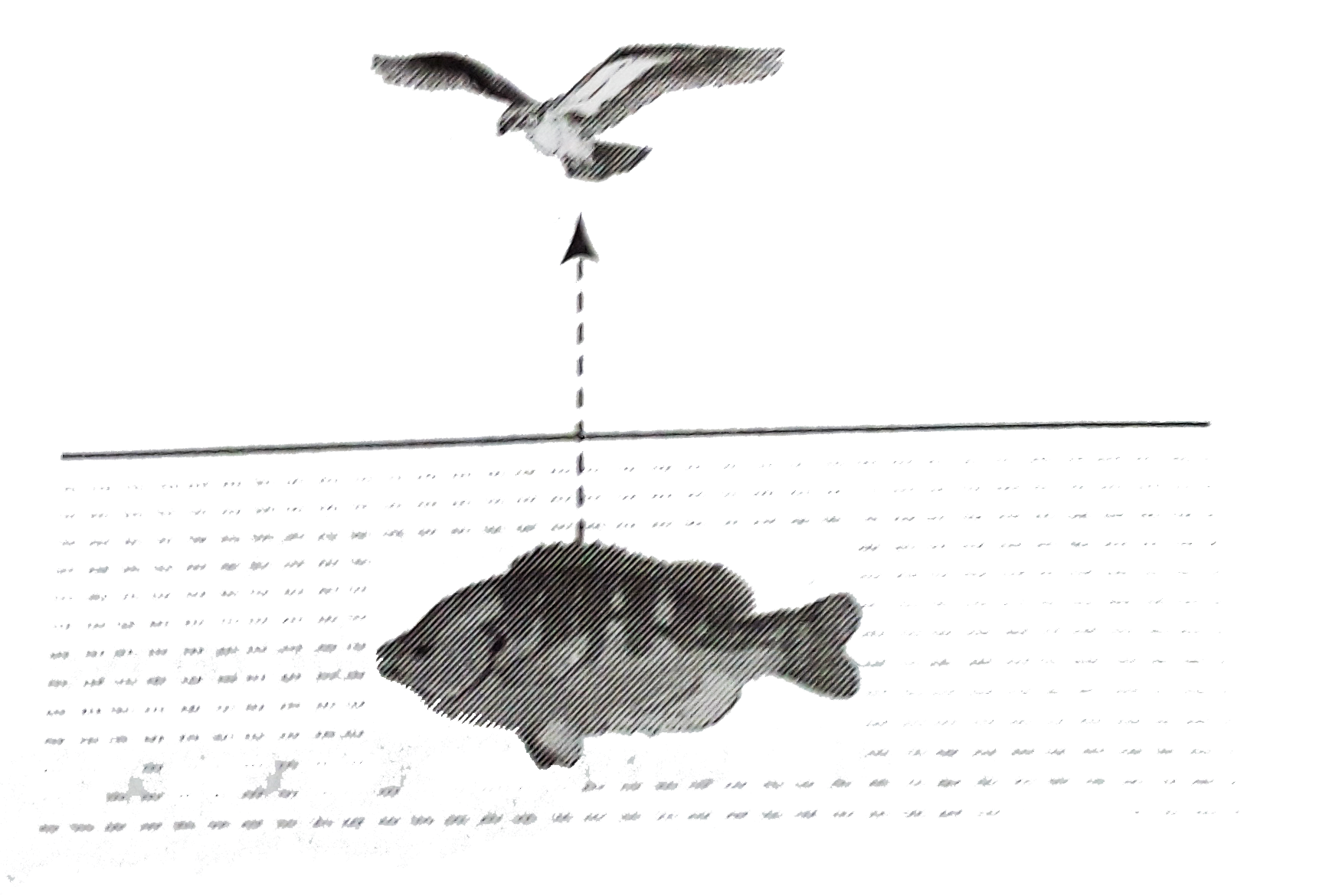
In a lake, a fish rising vertically to the surface of water uniformly at the rate of 3 m/s, observes a bird diving vertically towards the water at the rate of 9 m/s. The actual velocity of the dive of the bird is (given, refractive index of water = 4/3)
To solve the problem, we need to find the actual velocity of the bird diving into the water, given the apparent velocity observed by the fish and the refractive index of water.
1. Understanding the Situation:
- The fish is rising vertically at a speed of vf=3m/s.
- The bird is diving vertically towards the water, and the fish observes the bird diving at an apparent speed of vapparent=9m/s.
- The refractive index of water is given as μ=43.
2. Setting Up the Relationship:
- Let vb be the actual velocity of the bird diving into the water.
- The apparent height of the bird with respect to the fish can be expressed as:
happarent=h2+μh1
- Here, h2 is the height of the fish above the water surface, and h1 is the height of the bird above the water surface.
3. Differentiating the Apparent Height:
- By differentiating the equation with respect to time t:
dhapparentdt=dh2dt+μdh1dt
- Since the fish is moving upwards, dh2dt=3m/s (positive) and dhapparentdt=−9m/s (negative because the apparent height is decreasing).
4. Substituting Values:
- Substitute the known values into the differentiated equation:
−9=3+43dh1dt
5. Solving for dh1dt:
- Rearranging the equation gives:
−9−3=43dh1dt
−12=43dh1dt
- Now, multiply both sides by 34:
dh1dt=−12⋅34=−9m/s
6. Finding the Actual Velocity of the Bird:
- The actual velocity of the bird diving into the water, vb, can be calculated as:
vb=dh1dt+vf
- Substituting the values:
vb=−9+3=−6m/s
- The negative sign indicates that the bird is moving downwards.
7. Final Result:
- The actual velocity of the bird diving into the water is 6m/s downward.
Summary:
The actual velocity of the bird diving into the water is 6m/s downward.
- The fish is rising vertically at a speed of vf=3m/s.
- The bird is diving vertically towards the water, and the fish observes the bird diving at an apparent speed of vapparent=9m/s.
- The refractive index of water is given as μ=43.
- Let vb be the actual velocity of the bird diving into the water.
- The apparent height of the bird with respect to the fish can be expressed as:
happarent=h2+μh1
- Here, h2 is the height of the fish above the water surface, and h1 is the height of the bird above the water surface.
- By differentiating the equation with respect to time t:
dhapparentdt=dh2dt+μdh1dt
- Since the fish is moving upwards, dh2dt=3m/s (positive) and dhapparentdt=−9m/s (negative because the apparent height is decreasing).
- Substitute the known values into the differentiated equation:
−9=3+43dh1dt
- Rearranging the equation gives:
−9−3=43dh1dt
−12=43dh1dt
- Now, multiply both sides by 34:
dh1dt=−12⋅34=−9m/s
- The actual velocity of the bird diving into the water, vb, can be calculated as:
vb=dh1dt+vf
- Substituting the values:
vb=−9+3=−6m/s
- The negative sign indicates that the bird is moving downwards.
- The actual velocity of the bird diving into the water is 6m/s downward.
The actual velocity of the bird diving into the water is 6m/s downward.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS EXERCISE - Example21videos
RAY OPTICS
- BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS EXERCISE - PRACTICE EXERCISE-11videos
RAY OPTICS
- BOOK - VMC MODULES ENGLISHCHAPTER - QUIZEXERCISE - PHYSICS30videos
QUIZ
- BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - IMPECCABLE58videos
RAY OPTICS AND WAVE OPTICS
Similar Questions
A fish rising vertically to the surface of water in a lake uniformly at the rate of 3ms−1 observes a bird diving vertically towards the water at a rate of 9ms−1 vertically above it. If the refractive index of water is 4/3 the actual velocity of the dive of the bird is (in ms−1)
View SolutionA fish rising up vertically toward the surface of water with speed 3ms−1 observes a bird diving down vertically towards it with speed 9ms−1 . The actual velocity of bird is
 View Solution
View SolutionA fish rising up vertically toward the surface of water with speed 3ms−1 observes a bird diving down vertically towards it with speed 9ms−1 . The actual velocity of bird is
 View Solution
View SolutionA fish is rising up veritcally inside a pond with velocity 4m/s, and notices a bird, which is dividing downward and its velocity appear to be 16m/s (to fish). What is the real velocity of the diving bird, if refractive index of water is 4/3?
View SolutionThe escape velocity at the surface of Earth is approximately 8 km/s. What is the escape velocity for a planet whose radius is 4 times and whose mass is 100 times that of Earth?
View SolutionA point source of light is placed at the bottom of a water lake. If the area of the illuminated circle on the surface is equal to 3 times the square of the depth of the lake. The refractive index of water is
View SolutionA fish is vertically below a flying bird moving vertically down toward water surface. The bird will appear to the fish to be
 View Solution
View SolutionAn under water swimmer is at a depth of 12 m below the surface of water. A bird is at a height of 18 m from the surface of water, directly above his eyes. For the swimmer the bird appears to be at a distance from the surface of water equal to (Refractive Index of water is 4/3)
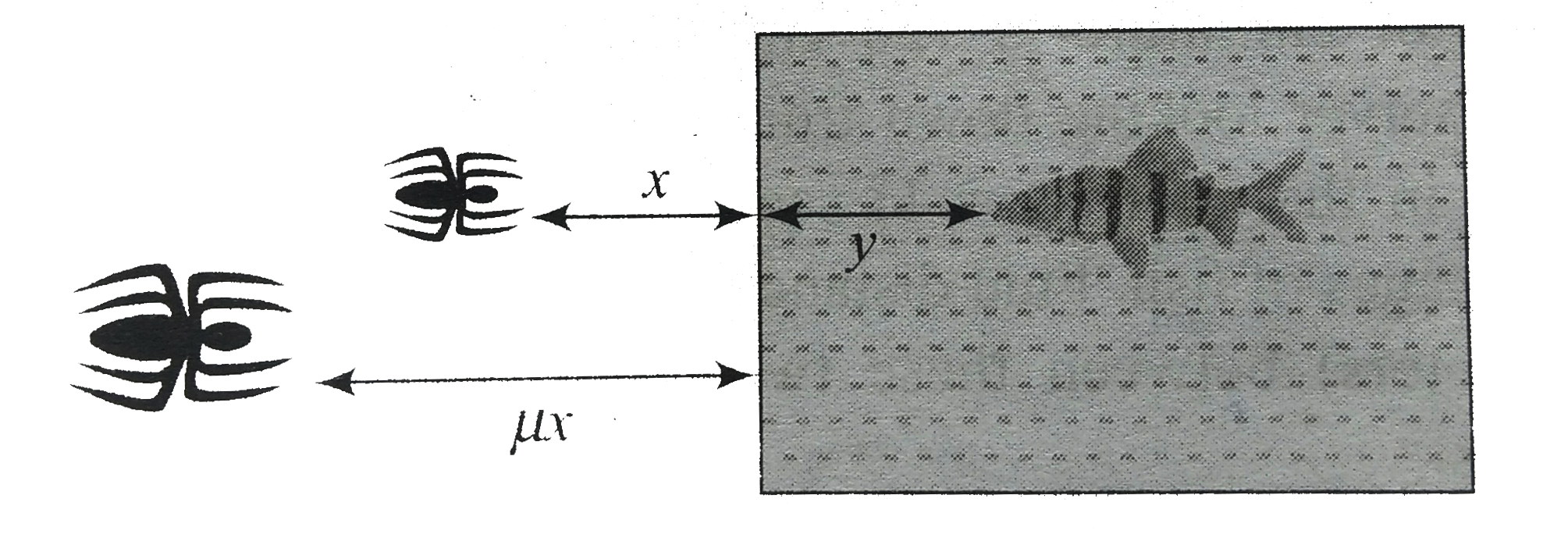
View SolutionA fish in an aquarium approaches the left wall at a rate of 3ms−1 observes a fly approaching it at 8ms−1. If the refractive index of water is (4/3), find the actual velocity of the fly.
 View Solution
View SolutionA fish in an aquarium approaches the left wall at a rate of 3ms−1 observes a fly approaching it at 8ms−1. If the refractive index of water is (4/3), find the actual velocity of the fly.
 View Solution
View Solution

