An equiconvex lens of refractive index 1.6 has power 4D in air. Its power in water is:
(a)1.5 D
(b)2.0 D
(c)1.3 D
(d)3.2 D
The correct Answer is:3
To find the power of the equiconvex lens in water, we can follow these steps:
Step 1: Understand the given information
We know:
- The refractive index of the lens (μ) = 1.6
- The power of the lens in air (P) = 4D
- The refractive index of water (n_water) = 1.33
Step 2: Use the lens maker's formula
The power (P) of a lens is given by the formula:
P=(μ−1)(1R1−1R2)
Where:
- μ is the refractive index of the lens
- R1 and R2 are the radii of curvature of the lens surfaces.
Step 3: Calculate the mean refractive index when the lens is in water
The mean refractive index of the lens in water (μ') can be calculated as:
μ′=μnwater=1.61.33
Step 4: Calculate the power of the lens in water
Using the modified lens maker's formula for the power in water:
P′=(μ′−1)(1R1−1R2)
We can express this in terms of the power in air (P):
P′=P⋅μ′−1μ−1
Step 5: Substitute the values
From the previous steps:
1. Calculate μ':
μ′=1.61.33≈1.202
2. Calculate (μ' - 1):
μ′−1≈1.202−1=0.202
3. Calculate (μ - 1):
μ−1=1.6−1=0.6
Now substitute these values into the power formula:
P′=4D⋅0.2020.6
Step 6: Calculate P'
P′=4D⋅0.3367≈1.347D
Final Result
The power of the lens in water is approximately 1.35 D.
We know:
- The refractive index of the lens (μ) = 1.6
- The power of the lens in air (P) = 4D
- The refractive index of water (n_water) = 1.33
The power (P) of a lens is given by the formula:
P=(μ−1)(1R1−1R2)
Where:
- μ is the refractive index of the lens
- R1 and R2 are the radii of curvature of the lens surfaces.
The mean refractive index of the lens in water (μ') can be calculated as:
μ′=μnwater=1.61.33
Using the modified lens maker's formula for the power in water:
P′=(μ′−1)(1R1−1R2)
We can express this in terms of the power in air (P):
P′=P⋅μ′−1μ−1
From the previous steps:
1. Calculate μ':
μ′=1.61.33≈1.202
2. Calculate (μ' - 1):
μ′−1≈1.202−1=0.202
3. Calculate (μ - 1):
μ−1=1.6−1=0.6
P′=4D⋅0.2020.6
P′=4D⋅0.3367≈1.347D
The power of the lens in water is approximately 1.35 D.
Topper's Solved these Questions
- RAY OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS EXERCISE - PRACTICE EXERCISE-
6 Videos
- RAY OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS EXERCISE - PRACTICE EXERCISE-8
6 Videos
- RAY OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS EXERCISE - PRACTICE EXERCISE-6
5 Videos
- QUIZBOOK - VMC MODULES ENGLISHCHAPTER - QUIZEXERCISE - PHYSICS
30 Videos
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - IMPECCABLE
58 Videos
Similar Questions
A convex lens of refractive index 3/2 has a power of 2.5∘. If it is placed in a liqud of refractive index 2,the new power of the lens is
View SolutionA convex lens (refractive index μ = 1.5) has a power P. If it is immersed in a liquid (μ = 4/3), then its power will become/remain
View SolutionA thin convex lens of refractive index 1.5cm has 20cm focal length in air. If the lens in completely immersed in a liquid of refractive index. 1.6, then its focal length will be
View SolutionA thin equiconvex lens of refractive index 3/2 is placed on a horizontal plane mirror as shown in figure. The space between the lens and the mirror is filled with a liquid of refractive index 4/3 . It is found that when a point object is placed 15 cm above the lens on its priincipal axis, the object coincides with its own image.
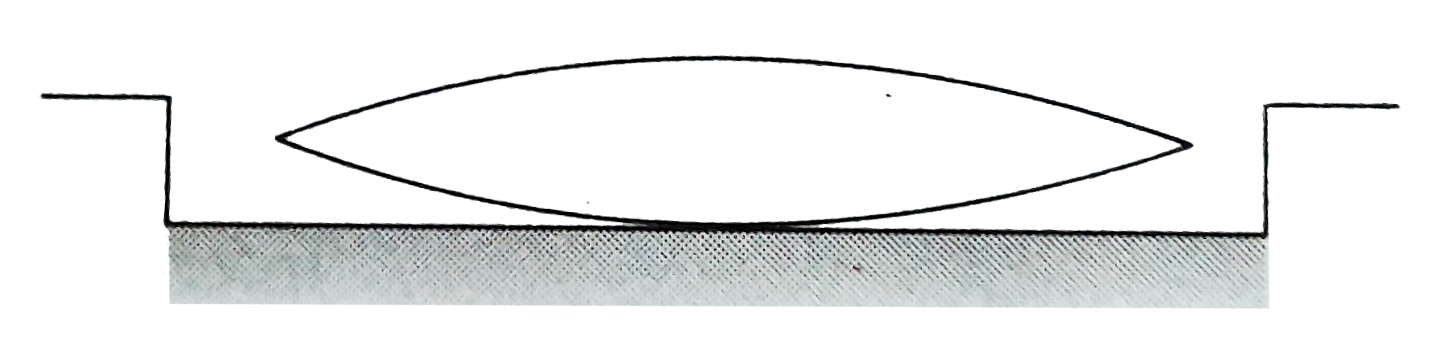
Q. If another liquid is filled instead of water, the object and the image coincide at a distance 25 cm from the lens.
Calculate the refractive index of the liquid.
View SolutionAn equiconvex lens of refractive index n2 is placed such that the refractive index of the surrouding media is as shown. The lens :
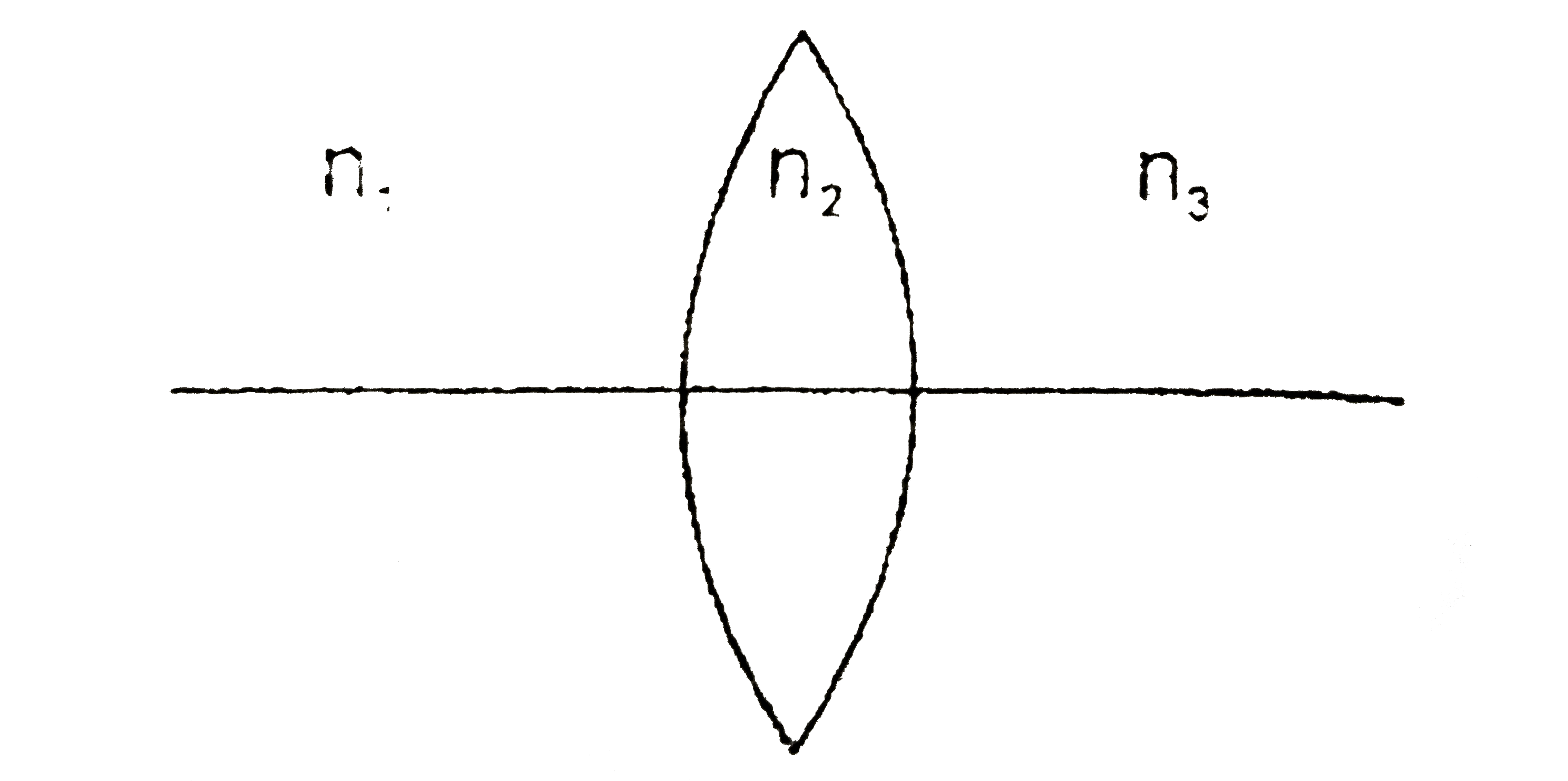
View SolutionA convex lens of refractive index 1.5 has a focal length of 20 cm in air. Calculate the change in its focal length when it is immersed in water of refractive index 4/3.
View SolutionA thin lens of refractive index 1.5 has focal length of 15cm in air. When the lens is placed is a medium of refractive index (4)/(3), its focal length will become …..cm.
View SolutionA thin glass (refractive index 1.5) lens has optical power of −5D in air. Its optical power in a liquid medium with refractive index 1.6 will be
View SolutionA thin glass (refractive index 1.5) lens has optical power of −5D in air. Its optical power in a liquid medium with refractive index 1.6 will be
View SolutionA double convex lens of refractive index m1 is immersed in a liquid of refractive index m2. This lens will act as:
View Solution
