Pressure difference between two cross sections of a venturimeter, of area 40cm2 & 20cm2 is 480 Pascals.Find the rate of flow of water in the ventrurimeter. ( Density of water =1000Kgm−3 )
1200cm3/s
1600√2cm3/s
1600cm3/s
1200√2cm3/s
The correct Answer is:B
To find the rate of flow of water in the venturimeter, we will use the principle of Bernoulli's theorem and the equation for a venturimeter.
1. Identify Given Values:
- Area of larger cross-section, A1=40cm2=40×10−4m2
- Area of smaller cross-section, A2=20cm2=20×10−4m2
- Pressure difference, ΔP=P1−P2=480Pa
- Density of water, ρ=1000kg/m3
2. Use the Venturimeter Equation:
The equation relating pressure difference to flow velocity is given by:
P1−P2ρ=v212(A21A22−1)
Rearranging gives:
v21=2(P1−P2)ρ(A22A21)
3. Calculate the Area Ratios:
A21A22=(40×10−420×10−4)2=(2)2=4
Therefore,
A22A21=14
4. Substitute Values into the Equation:
v21=2×4801000(4−1)
v21=9601000×3=0.96×3=2.88
5. Calculate v1:
v1=√2.88=2√25m/s
6. Calculate the Rate of Flow Q:
The rate of flow Q is given by:
Q=A1×v1
Substituting the values:
Q=40×10−4×2√25=80√25×10−4=16√2×10−4m3/s
7. Convert to cm3/s:
Q=16√2×10−4×106=1600√2cm3/s
Final Answer:
The rate of flow of water in the venturimeter is 1600√2cm3/s.
- Area of larger cross-section, A1=40cm2=40×10−4m2
- Area of smaller cross-section, A2=20cm2=20×10−4m2
- Pressure difference, ΔP=P1−P2=480Pa
- Density of water, ρ=1000kg/m3
The equation relating pressure difference to flow velocity is given by:
P1−P2ρ=v212(A21A22−1)
Rearranging gives:
v21=2(P1−P2)ρ(A22A21)
A21A22=(40×10−420×10−4)2=(2)2=4
Therefore,
A22A21=14
v21=2×4801000(4−1)
v21=9601000×3=0.96×3=2.88
v1=√2.88=2√25m/s
The rate of flow Q is given by:
Q=A1×v1
Substituting the values:
Q=40×10−4×2√25=80√25×10−4=16√2×10−4m3/s
Q=16√2×10−4×106=1600√2cm3/s
The rate of flow of water in the venturimeter is 1600√2cm3/s.
Topper's Solved these Questions
- MOCK TEST 13BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 13EXERCISE - PHYSICS ( SECTION -2)
5 Videos
- MOCK TEST 12BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 12EXERCISE - PHYSICS (SECTION 2)
5 Videos
- MOCK TEST 2BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 2EXERCISE - PART I : PHYSICS (SECTION - 2)
10 Videos
Similar Questions
A stream of water flowing horizontally with a speed of 15ms−1 pushes out of a tube of cross sectional area 10−2m2 and hits a vertical wall near by what is the force exerted on the wall by the impact of water assuming.that it does not rebound? (Density of water =1000kgm3)
View SolutionWater flows in a horizontal tube (see figure). The pressure of water changes by 700 Nm−2 between A and B where the area of cross section are 40cm2 and 20cm2, respectively. Find the rate of flow of water through the tube.

View Solution
Knowledge Check
A stream of water flowing horizontally with a speed of 15ms−1 pushes out of a tube of cross sectional area 10−2m2 and hits a vertical wall nearby. What is the force exerted on the wall by the impact of water assuming that it does not rebound? (Density of water =1000kgm−3)
A1.25×103NB2.25×103NC3.25×103ND4.25×103N
Water flows through the tube shown in figure. The areas of cross section of the wide and the narrow portions of the tube are 5cm2and2cm2 respectively. The rate of flow of water through the tube is 500cm3s−1 . Find the difference of mercury levels in the U-tube.

View SolutionWater flows through the tube shown in figure. The areas of cross section of the wide and the narrow portions of the tube are 5cm2and2cm2 respectively. The rate of flow of water through the tube is 500cm3s−1 . Find the difference of mercury levels in the U-tube.

View SolutionWater flows through a horizontal tube as shown in figure. If the difference of height of water column in the vertical tubes in 2cm and the areas of corss-section at A and B are 4cm2 and 2cm2 respectively. Find the rate of flow of water across any section.
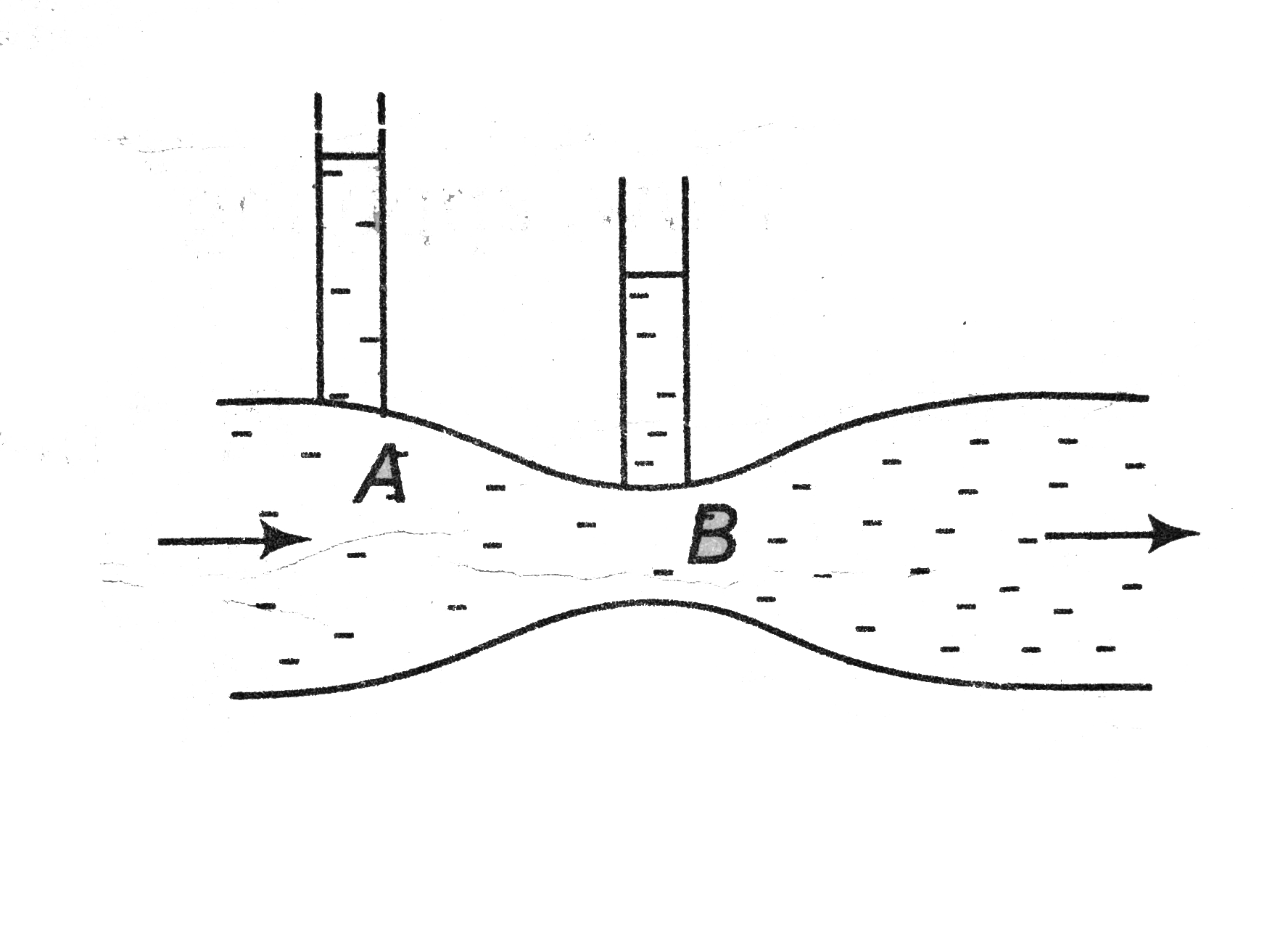 .
.
View SolutionTwo water pipes of diameters 2 cm and 4 cm are connected with the main supply line. The velocity of flow of water in the pipe of 2 cm
View SolutionA 700 g solid cube having an edge of length 10 cm floats in water. How much volume of the cube is outside the water? Density of water =1000kgm−3
View SolutionFlow rate of blood through a capillary of cross - sectional are of 0.25m2 is 100cm3/s. The velocity of flow of blood iis
View SolutionThe area of cross-section of the wider tube shown in fig., is 800cm2 . If a mass of 12 kg is placed on the massless piston, what is the difference in the level of water in two tubes.

View Solution
