The weight W of a certain stock of fish is given by W = nw, where n is the size of stock and w is the average weight of a fish. If n and w change with time t as n = 2t2+3 and w=t2−t+2, then the rate of change of W with respect to t at t = 1, is
1
13
5
8
The correct Answer is:B
To solve the problem step by step, we need to find the rate of change of the weight W of a certain stock of fish with respect to time t. The weight W is given by the product of the size of the stock n and the average weight of a fish w.
Step 1: Define the Variables
Given:
- W=n⋅w
- n=2t2+3
- w=t2−t+2
Step 2: Differentiate W with Respect to t
Using the product rule of differentiation, we have:
dWdt=n⋅dwdt+w⋅dndt
Step 3: Calculate dwdt and dndt
1. Differentiate w:
w=t2−t+2⟹dwdt=2t−1
2. Differentiate n:
n=2t2+3⟹dndt=4t
Step 4: Substitute n, w, dwdt, and dndt into the equation
Now substituting these values into the equation for dWdt:
dWdt=(2t2+3)(2t−1)+(t2−t+2)(4t)
Step 5: Simplify the Expression
1. Expand (2t2+3)(2t−1):
=4t3−2t2+6t−3
2. Expand (t2−t+2)(4t):
=4t3−4t2+8t
3. Combine the two expansions:
dWdt=(4t3−2t2+6t−3)+(4t3−4t2+8t)
=8t3−6t2+14t−3
Step 6: Evaluate at t=1
Now, substitute t=1 into dWdt:
dWdt∣∣∣t=1=8(1)3−6(1)2+14(1)−3
=8−6+14−3=13
Final Answer
The rate of change of W with respect to t at t=1 is 13.
Given:
- W=n⋅w
- n=2t2+3
- w=t2−t+2
Using the product rule of differentiation, we have:
dWdt=n⋅dwdt+w⋅dndt
1. Differentiate w:
w=t2−t+2⟹dwdt=2t−1
n=2t2+3⟹dndt=4t
Now substituting these values into the equation for dWdt:
dWdt=(2t2+3)(2t−1)+(t2−t+2)(4t)
1. Expand (2t2+3)(2t−1):
=4t3−2t2+6t−3
=4t3−4t2+8t
dWdt=(4t3−2t2+6t−3)+(4t3−4t2+8t)
=8t3−6t2+14t−3
Now, substitute t=1 into dWdt:
dWdt∣∣∣t=1=8(1)3−6(1)2+14(1)−3
=8−6+14−3=13
The rate of change of W with respect to t at t=1 is 13.
Topper's Solved these Questions
- DERIVATIVE AS A RATE MEASURER BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DERIVATIVE AS A RATE MEASURER EXERCISE - Exercise
26 Videos
- DERIVATIVE AS A RATE MEASURER BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DERIVATIVE AS A RATE MEASURER EXERCISE - Exercise
26 Videos
- DEFINITE INTEGRALSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DEFINITE INTEGRALSEXERCISE - Chapter Test 2
56 Videos
- DIFFERENTIAL EQUATIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Chapter Test
30 Videos
Similar Questions
the angular velocity omega of a particle varies with time t as ω=5t2+25rads. the angular acceleration of the particle att=1s is
View SolutionCurrent in an ac circuit is given by I=3sinωt+4cosωt, then
View SolutionThe displacement vector of a particle of mass m is given by r (t) = ˆiAcosωt+ˆjBsinωt.
(a) Show that the trajectory is an ellipse.
(b) Show that F = −mω2r.
View SolutionThe displacement vector of a particle of mass m is given by r (t) = ˆiAcosωt+ˆjBsinωt.
(a) Show that the trajectory is an ellipse.
(b) Show that F = −mω2r.
View SolutionIn the expression y=asin(ωt+θ), y is the displacement and t is the time . Write the dimension ofa,ωand θ .
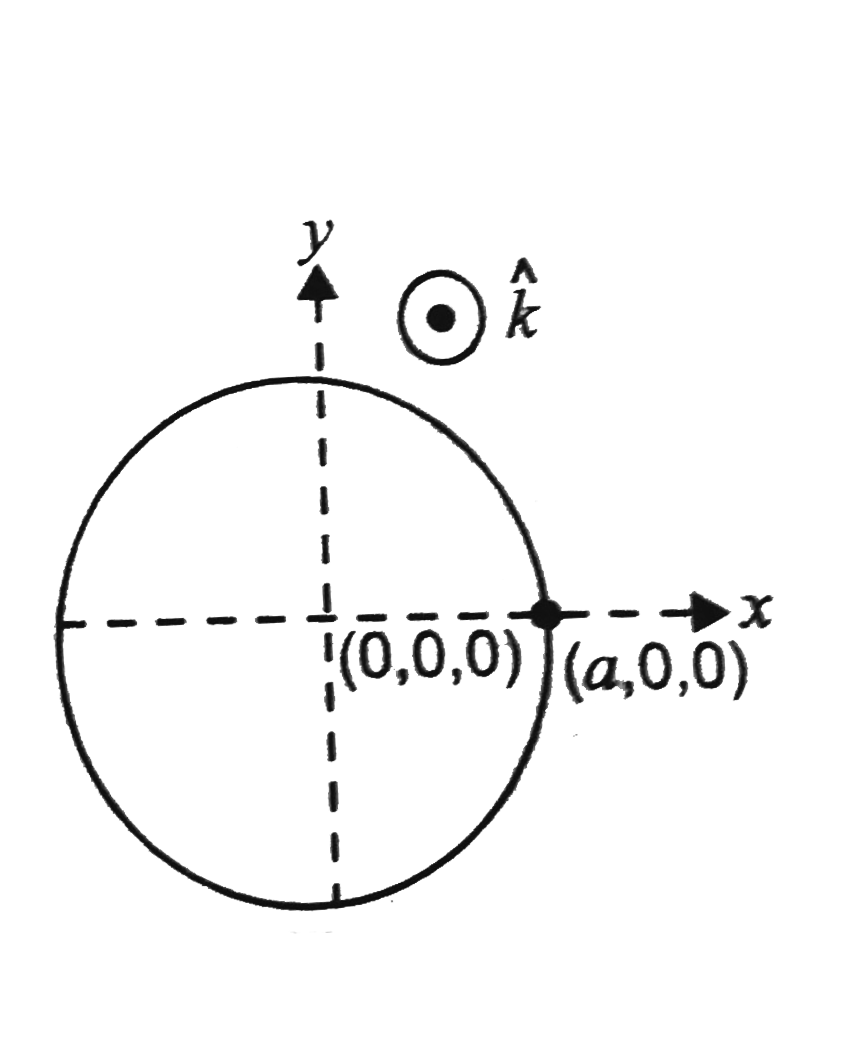
View SolutionA magnetic field in a certain region is given by B=B0cos(ωt)ˆk and a coil of radius a with resistance R is placed in the x-y plane with its centre at the origin in the magnetic field, Fig. Find the magnitude and the direction of the current at (a,0,0) att=π/2ω,t=π/ω and t=3π/2ω.
View SolutionThe SHM of a particle is given by the equation x=2sinωt+4cosωt. Its amplitude of oscillation is
View SolutionA particle moves so that its position vector is given by →r=cosωtˆx+sinωtˆy, where ω is a constant which of the following is true ?
View SolutionA particle moves so that its position vector is given by →r=cosωtˆx+sinωtˆy, where ω is a constant which of the following is true ?
View SolutionPosition vector of a particle moving in x−y plane at time t is r=a(1−cosωt)ˆi+asinωtˆj. The path of the particle is
View Solution
