The number of 6-digit numbers that can be formed using the three digits 0,1 and 2, is
36
2×35
35
none of these
The correct Answer is:C
Since 0 cannot be at the first place and the remaining each place can be filled in 3 ways.
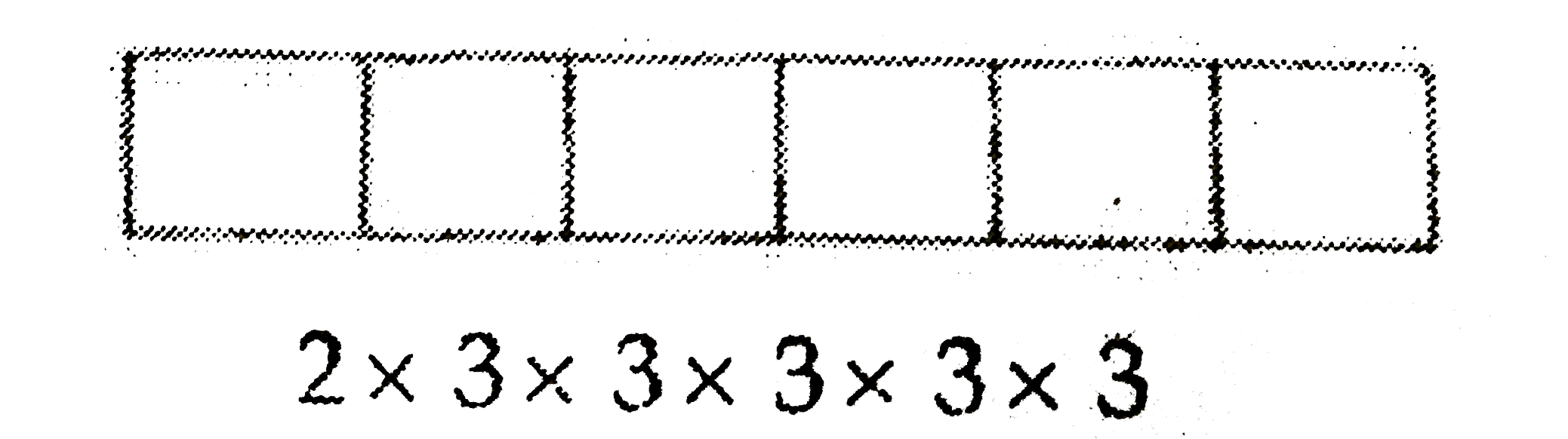
Hence, required number of numbers =2×35.
Topper's Solved these Questions
- PERMUTATIONS AND COMBINATIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Section II - Assertion Reason Type
9 Videos
- PERMUTATIONS AND COMBINATIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Exercise
136 Videos
- PERMUTATIONS AND COMBINATIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Chapter Test
60 Videos
- PARABOLABOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PARABOLAEXERCISE - Chapter Test
30 Videos
- PROBABILITY BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PROBABILITY EXERCISE - Chapter Test
45 Videos
Similar Questions
Knowledge Check
The number of six digit numbers that can be formed by using the digits 1,2,1,2,0,2 is
A50B60C110D10The number of 4 digit numbers that can be formed with the digits 2,3,4,7 and using each digit only once is
A120B96C24D100
Find the number of 4-digit numbers that can be formed using the digits 1, 2, 3, 4, 5 if no digit is repeated. How many of these will be even?
View SolutionFind the numbers of 4-digit numbers that can be formed using the digits, 1,2,3,4,5 if no digit is repeated ? How many of these will be even?
View SolutionThe number of 5-digit number that can be made using the digits 1 and 2 and in which at laest one digits is different, is
View SolutionThe number of 6 digit numbers that can be made with the digits 0,1,2,3,4 and 5 so that even digits occupy odd places is
View SolutionThe number of four-digit numbers that can be formed by using the digits 1,2,3,4,5,6,7,8 and 9 such that the least digit used is 4, when repetition of digits is allowed is
View SolutionFind the number of 4 digit numbers that can be formed using the digits 1,2,3,4,5 if no digit is repeated. How many of these will be even?
View Solution
