If A and B are two independent events such that P(¯¯¯A)=710,P(¯¯¯B)=α andP(A∪B)=810, then α, is
2/7
5/7
1
none of these
The correct Answer is:A
To solve the problem, we need to find the value of α given the probabilities of independent events A and B.
1. Understanding the Given Information:
- We have P(¯¯¯¯A)=710
- We have P(¯¯¯¯B)=α
- We have P(A∪B)=810
2. Finding P(A) and P(B):
- Since P(¯¯¯¯A)=710, we can find P(A):
P(A)=1−P(¯¯¯¯A)=1−710=310
- For P(B), since P(¯¯¯¯B)=α:
P(B)=1−P(¯¯¯¯B)=1−α
3. Using the Formula for the Union of Two Independent Events:
- For independent events, the probability of the union is given by:
P(A∪B)=P(A)+P(B)−P(A)P(B)
- Substituting the known values:
810=P(A)+P(B)−P(A)P(B)
- Substitute P(A)=310 and P(B)=1−α:
810=310+(1−α)−(310(1−α))
4. Simplifying the Equation:
- Rewrite the equation:
810=310+1−α−310+3α10
- Combine like terms:
810=1−α+3α10
- Rearranging gives:
810=1−α+3α10
- Multiply everything by 10 to eliminate the fraction:
8=10−10α+3α
- This simplifies to:
8=10−7α
5. Solving for α:
- Rearranging gives:
7α=10−8
7α=2
α=27
Final Answer:
Thus, the value of α is 27.
- We have P(¯¯¯¯A)=710
- We have P(¯¯¯¯B)=α
- We have P(A∪B)=810
- Since P(¯¯¯¯A)=710, we can find P(A):
P(A)=1−P(¯¯¯¯A)=1−710=310
- For P(B), since P(¯¯¯¯B)=α:
P(B)=1−P(¯¯¯¯B)=1−α
- For independent events, the probability of the union is given by:
P(A∪B)=P(A)+P(B)−P(A)P(B)
- Substituting the known values:
810=P(A)+P(B)−P(A)P(B)
- Substitute P(A)=310 and P(B)=1−α:
810=310+(1−α)−(310(1−α))
- Rewrite the equation:
810=310+1−α−310+3α10
- Combine like terms:
810=1−α+3α10
- Rearranging gives:
810=1−α+3α10
- Multiply everything by 10 to eliminate the fraction:
8=10−10α+3α
- This simplifies to:
8=10−7α
- Rearranging gives:
7α=10−8
7α=2
α=27
Thus, the value of α is 27.
Topper's Solved these Questions
- PROBABILITY BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PROBABILITY EXERCISE - Section- II (Assertion -Reason Types MCQs)
15 Videos
- PROBABILITY BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PROBABILITY EXERCISE - Section I - Mcqs
89 Videos
- PROBABILITY BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PROBABILITY EXERCISE - Chapter Test
45 Videos
- PERMUTATIONS AND COMBINATIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Chapter Test
60 Videos
- QUADRATIC EXPRESSIONS AND EQUATIONS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - QUADRATIC EXPRESSIONS AND EQUATIONS EXERCISE - Chapter Test
50 Videos
Similar Questions
If A and B are two independent events, such that P(A)=13andP(B)=14.
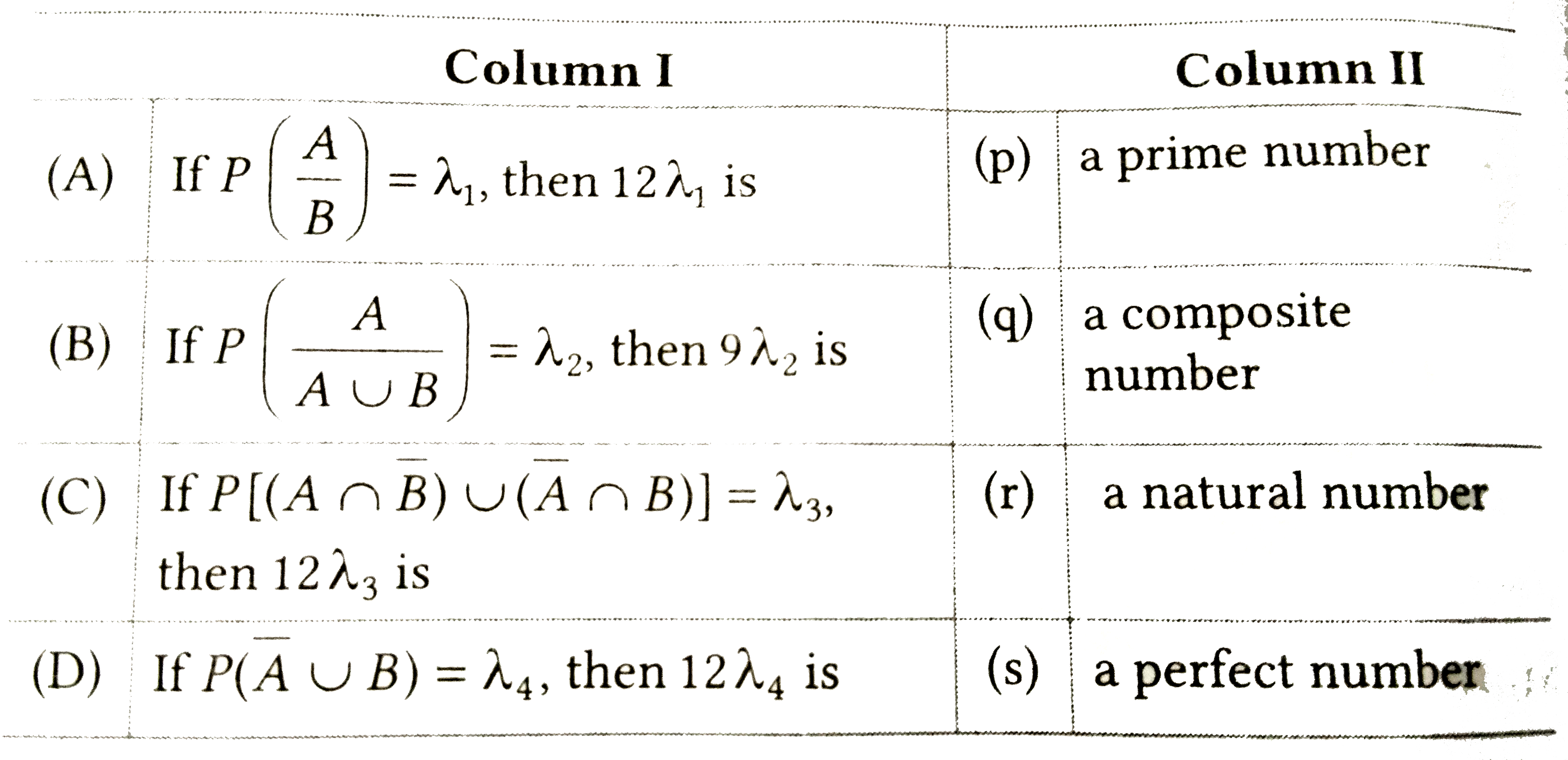
View SolutionIf A and B are two independent events such that P (A) =1/2,P(B)=1/5, then
View SolutionIf A and B are two independent events such that P(¯¯¯A∩B)=2/15 and P(A∩¯¯¯B)=1/6, then P(B), is
View SolutionA and B are two independent events such that P(A)=15,P(A∪B)=710. Then ,P(¯¯¯B)=
View SolutionIf A and B are independent events such that P(A)>0,P(B)>0, then
View SolutionIf A and B are two independent events such that P(A)=710, P(B')=α, P(A∪B)=810, then α =
View SolutionIf P(A)=14,P(¯¯¯B)=12 and P(A∪B)=59, then P(A/B) is
View SolutionIf A and B are independent events such that P(A)>0,P(B)>0, then
View SolutionIf A and B are two independent events, then P( A and B)=P(A)⋅P(B)
View SolutionIf A and B are two independent events such that P(B) = 27,P(A∪Bc)=0.8, then find P(A).
View Solution
