Among the workers in a factory only 30% receive bonus and among those receiving bonus only 20% are skilled. The probability that a randomly selected worker is skilled and is receiving bonus, is
0.03
0.02
0.06
0.015
The correct Answer is:C
To find the probability that a randomly selected worker is both skilled and receiving a bonus, we can follow these steps:
Step 1: Determine the probability of receiving a bonus
According to the problem, only 30% of the workers receive a bonus. This can be expressed as a fraction:
P(Bonus)=0.30=310
Step 2: Determine the probability of being skilled among those who receive a bonus
Among the workers who receive a bonus, only 20% are skilled. This can also be expressed as a fraction:
P(Skilled|Bonus)=0.20=210
Step 3: Calculate the probability of being skilled and receiving a bonus
To find the probability that a worker is both skilled and receiving a bonus, we use the formula for conditional probability:
P(Skilled and Bonus)=P(Bonus)×P(Skilled|Bonus)
Substituting the values we found:
P(Skilled and Bonus)=310×210=6100
Step 4: Simplify the probability
The fraction 6100 can be simplified to:
P(Skilled and Bonus)=0.06
Final Answer
The probability that a randomly selected worker is skilled and receiving a bonus is:
0.06
According to the problem, only 30% of the workers receive a bonus. This can be expressed as a fraction:
P(Bonus)=0.30=310
Among the workers who receive a bonus, only 20% are skilled. This can also be expressed as a fraction:
P(Skilled|Bonus)=0.20=210
To find the probability that a worker is both skilled and receiving a bonus, we use the formula for conditional probability:
P(Skilled and Bonus)=P(Bonus)×P(Skilled|Bonus)
Substituting the values we found:
P(Skilled and Bonus)=310×210=6100
The fraction 6100 can be simplified to:
P(Skilled and Bonus)=0.06
The probability that a randomly selected worker is skilled and receiving a bonus is:
0.06
Topper's Solved these Questions
- PROBABILITY BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PROBABILITY EXERCISE - Section II - Assertion Reason Type
22 Videos
- PERMUTATIONS AND COMBINATIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Chapter Test
60 Videos
- QUADRATIC EXPRESSIONS AND EQUATIONS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - QUADRATIC EXPRESSIONS AND EQUATIONS EXERCISE - Chapter Test
50 Videos
Similar Questions
10 mangoes are to be distributed among 5 persons. The probability that at least one of them will receive none, is
View SolutionThere are 60% students in Maths and 30% in Biology. If 10% students are in both subjects. Find the probability that a randomly selected student has Maths or Biology.
View Solution
Knowledge Check
Of all the articles in a box, 80% are satisfactory, while 20% are not. The probability of
obtaining exactly five good items out of eight randomly selected articles isA0.003B0.013C0.132D0.147Of all the articles in a box, 80% are satisfactory, while 20% are not. The probability of
obtaining exactly five good items out of eight randomly selected articles isA0.003B0.013C0.132D0.147A research study was conducted to determine whether a certain electrical implant, I, is successful in improving hearing . From a large population of people with hearing loss, 400 participants were selected at random. Half were randomly selected to receive the implant and the other half did not receive the implant . The resulting data showed that people who received the implant had significantly improved hearing compared to those who did not receive the implant.
Based on the study , which of the following is an appropriate conclusion ?AElectrical implant I is likely to improve the hearing of people who have hearing loss.BElectrical implant I is likely to improve the hearing of all people who have the implant.CElectrical implant I is better than other treatments for hearing loss.DElectrical implant I will cause a substantial improvement in hearing.
If m things are distributed among a men and b women. Then the probability that number of things received by men is odd is
View Solution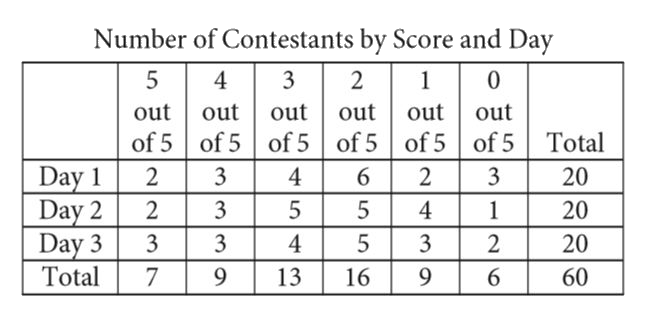
The same 20 contestants, on each of 3 days, answered 5 questions in order to win a prize. Each contestant received 1 point for each correct answer. The number of contestants receiving a given score on each day is shown in the table above.
No contestant received the same score on two different days. If a contestant is selected at random, what is the probability that the selected contestant received a score of 5 on Day 2 or Day 3, given that the contestant received a score of 5 on one of the three days?
View SolutionThere are only two women among 20 persons taking part in a pleasure trip. The 20 persons are divided into two groups, each group consisting of 10 person. Then the probability that the two women will be in the same group is a. 9/19 b. 9/38 c. 9/35 d. none
View SolutionA real estate man has eight master keys to open several new homes. Only in master key will open any given house. If 40% of these homes are usually left unlocked, what is the probability that the real estate man can get into a specific home if he selects three master keys at random before leaving the office?
View SolutionA real estate man has eight master keys to open several new homes. Only in master key will open any given hour. If 40% of these homes are usually left unlocked, what is the probability that the real estate man can get into a specific home if he selects three master keys at random before leaving the office?
View Solution
