In a rhombus ABCD the diagonals AC and BD intersect at the point (3,4) . If the point A is (1,2) the diagonal BD has the equation
x - y - 1 = 0
x + y - 1 = 0
x - y + 1 = 0
x + y - 7 =0
The correct Answer is:D
To find the equation of the diagonal BD in the rhombus ABCD, we will follow these steps:
Step 1: Identify the given points
We are given:
- The intersection point of the diagonals (which is also the midpoint of both diagonals) is (3,4).
- The coordinates of point A are (1,2).
Step 2: Calculate the slope of diagonal AC
The slope m1 of line AC can be calculated using the formula for the slope between two points:
m1=y2−y1x2−x1
Here, we can take point A as (x1,y1)=(1,2) and the intersection point as (x2,y2)=(3,4):
m1=4−23−1=22=1
Step 3: Determine the slope of diagonal BD
Since the diagonals of a rhombus are perpendicular to each other, the slope m2 of diagonal BD can be found using the relationship:
m1⋅m2=−1
Substituting m1=1:
1⋅m2=−1⟹m2=−1
Step 4: Use the point-slope form to find the equation of line BD
The point-slope form of a line is given by:
y−y1=m(x−x1)
Substituting m=−1 and the point (3,4):
y−4=−1(x−3)
Expanding this:
y−4=−x+3
Rearranging gives:
x+y−7=0
Step 5: Final equation of diagonal BD
Thus, the equation of diagonal BD is:
x+y−7=0
We are given:
- The intersection point of the diagonals (which is also the midpoint of both diagonals) is (3,4).
- The coordinates of point A are (1,2).
The slope m1 of line AC can be calculated using the formula for the slope between two points:
m1=y2−y1x2−x1
Here, we can take point A as (x1,y1)=(1,2) and the intersection point as (x2,y2)=(3,4):
m1=4−23−1=22=1
Since the diagonals of a rhombus are perpendicular to each other, the slope m2 of diagonal BD can be found using the relationship:
m1⋅m2=−1
Substituting m1=1:
1⋅m2=−1⟹m2=−1
The point-slope form of a line is given by:
y−y1=m(x−x1)
Substituting m=−1 and the point (3,4):
y−4=−1(x−3)
Expanding this:
y−4=−x+3
Rearranging gives:
x+y−7=0
Thus, the equation of diagonal BD is:
x+y−7=0
Topper's Solved these Questions
- STRAIGHT LINESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Chapter Test
34 Videos
- STRAIGHT LINESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - SECTION - II (ASSERTION - REASON TYPE MCQs
1 Video
- SETSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SETSEXERCISE - Chapter Test
30 Videos
Similar Questions
In rhombus ABCD, diagonals AC and BD intersect each other at point O.
If cosine of angle CAB is 0.6 and OB = 8 cm, find the lengths of the side and the diagonals of the rhombus.
View SolutionABCD is a rhombus. Its diagonals ACandBD intersect at the point M and satisfy BD=2AC.If he point DandM represent the complex numbers 1+iand2−i respectively, then A represents the complex number......or......
View SolutionThe diagonals AC and BD of a rhombus intersect at (5,6). If A=(3,2), then find the equation of diagonal BD.
View SolutionIn quadrilateral ABCD, the diagonals AC and BD intersect each other at point O.
If AO = 2CO and BO = 2DO, show that:
ΔAOB is similar to ΔCOD.
View SolutionIf the diagonals AC and BD intersect at point P in the cube in Figure what is the degree measure of angle APB ?
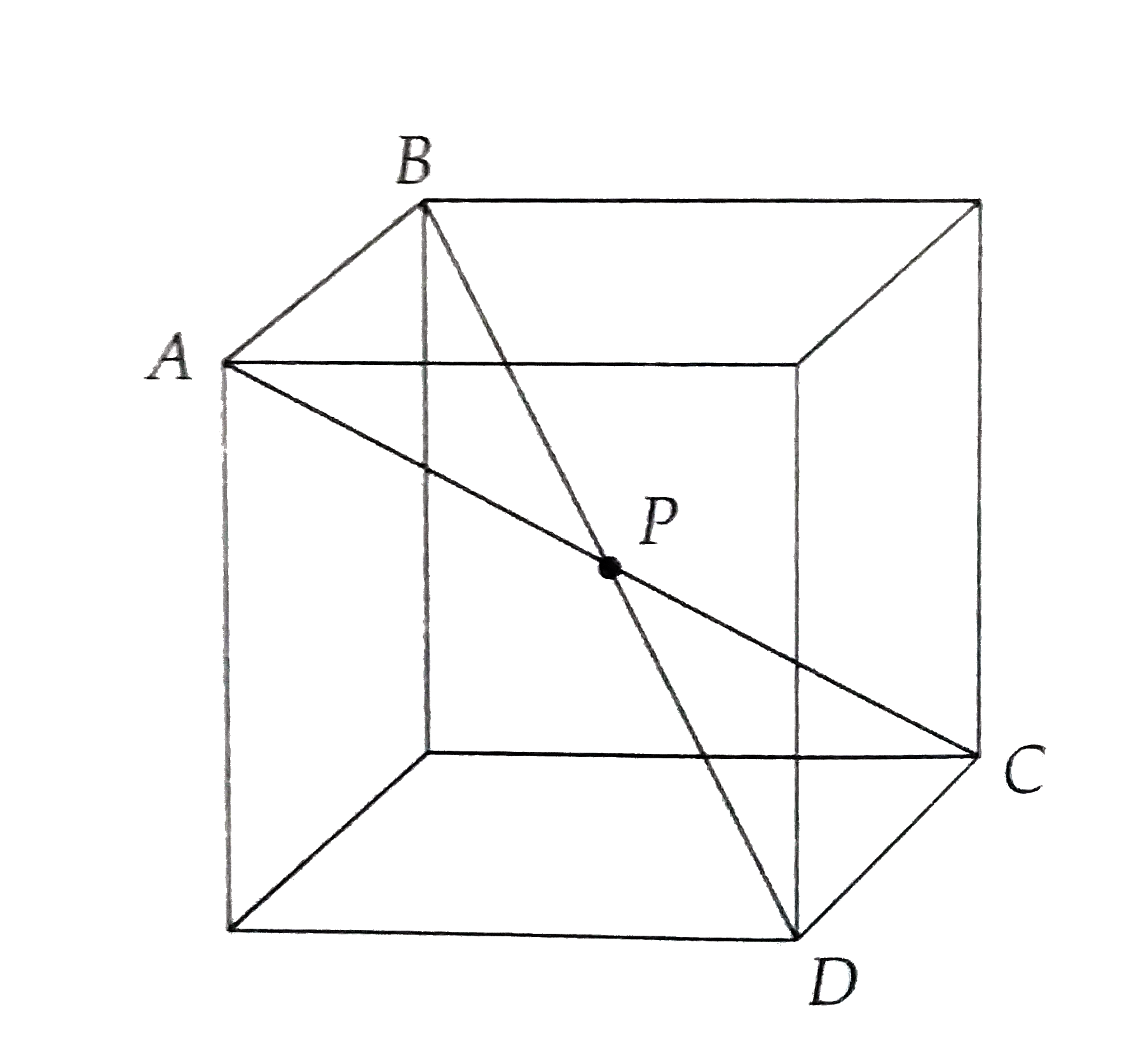
View SolutionIn quadrilateral ABCD, the diagonals AC and BD intersect each other at point O.
If AO = 2CO and BO = 2DO, show that:
OA×OD=OB×OC.
View SolutionThe diagonals AC and BD of a rhombus intersect at (5,6). If A≡(3,2), then find the equation of diagonal BD.
View SolutionIn trapezium ABCD, side AB is parallel to side DC. Diagonals AC and BD intersect at point P. Prove that triangles APD and BPC are equal in area.
View SolutionTwo sides of a rhombus ABCD are parallel to the lines y = x + 2 and y = 7x + 3 If the diagonals of the rhombus intersect at the point (1, 2) and the vertex A is on the y-axis, then vertex A can be
View SolutionTwo sides of a rhombus ABCD are parallel to the lines y = x + 2 and y = 7x + 3 If the diagonals of the rhombus intersect at the point (1, 2) and the vertex A is on the y-axis, then vertex A can be
View Solution