Natural numbers are written as 1, (2,3), (4,5,6)..
Show that the sum of number in the nth group is .
62525
65255
56255
55625
The correct Answer is:A
Step by step video & image solution for Natural numbers are written as 1, (2,3), (4,5,6).. Show that the sum of number in the nth group is (n)/(2) (n +1) . by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SEQUENCES AND SERIESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise
129 Videos
- QUADRATIC EXPRESSIONS AND EQUATIONS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - QUADRATIC EXPRESSIONS AND EQUATIONS EXERCISE - Chapter Test
50 Videos
- SETSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SETSEXERCISE - Chapter Test
30 Videos
Similar Questions
The sequence of odd natural numbers is divided into groups 1,3,5,7,9,11,…. and so on. Show that the sum of the numbers in nth group is n3.
View SolutionThe sequence N of natural numbers is divided into classes as follows. Show that the sum of the numbers in nth row is (2n2+1)
View SolutionThe series of natural numbers is divided into groups:(1);(2,3,4);(5,6,7,8,9)... and so on. The sum of numbers in the nth group is
View SolutionThe series of natural numbers is divided into groups:(1);(2,3,4);(5,6,7,8,9)... and so on. The sum of numbers in the nth group is
View SolutionNatural numbers have been divided into groups in the following way: 91,3),(5,7,9,11),(13,15,….,23),…….. Show that the sum of numbers in the nth group is 4n3
View SolutionThe cubes of the natural numbers are grouped as 13,(23,33),(43,53,63),........, the the sum of the number in the nth group, is
View SolutionSum to n terms the series whose nth terms is 14n2−1
View SolutionThe natural numbers arearranged innthe form given below
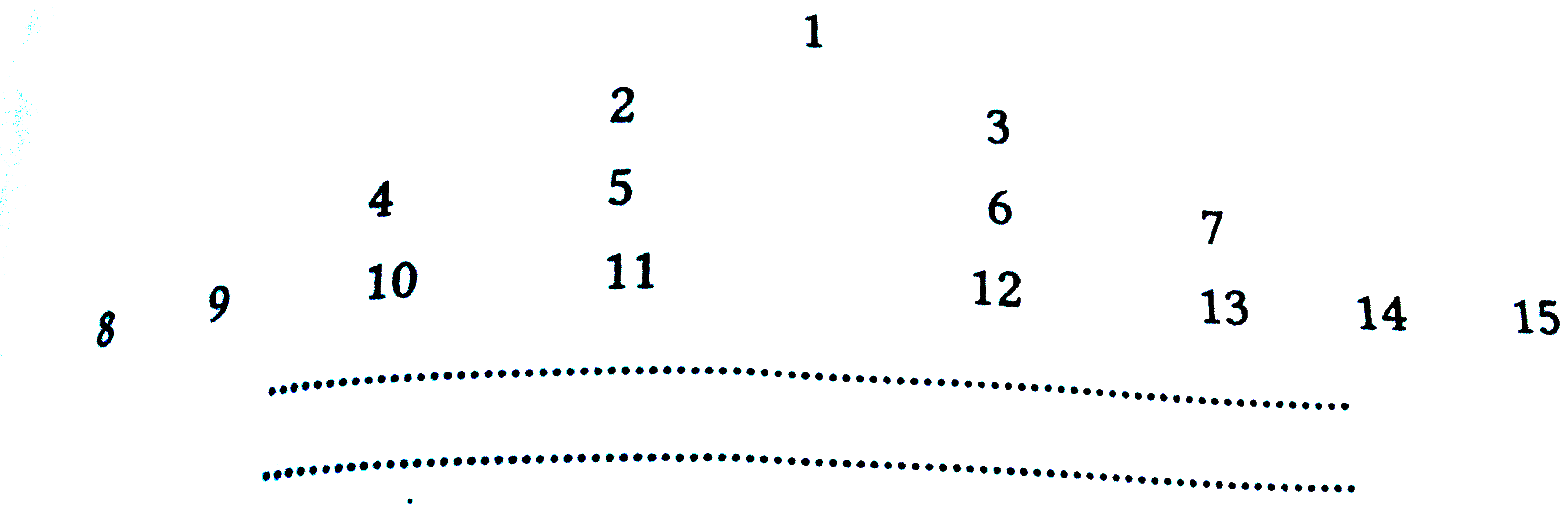
The rth group containing 2r−1 numbers. Prove that sum of the numbers in the nth group is 2n−2[2n+2n−1−1].
View SolutionTwo consecutive number p,p + 1 are removed from natural numbers. 1,2,3, 4……….., n−1, n such that arithmetic mean of these number reduces by 1, then (n-p) is equal to.
View SolutionThe total number of ways of selecting two numbers from the set {1,2,3,4,........3n} so that their sum is divisible by 3 is equal to a. 2n2−n2 b. 3n2−n2 c. 2n2−n d. 3n2−n
View Solution
