The area enclosed between the curves $y = x and y = 2 x - x^{2}$ (in square units), is

A

$\frac{1}{2}$

B

$\frac{1}{6}$

C

$\frac{1}{3}$

D

$\frac{1}{4}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Clearly,
Shaded Area $= 1 \int 0 {(2 x - x^{2}) - x} d x$
$\Rightarrow Shaded Area = 1 \int 0 (x - x^{2}) d x = {[\frac{x^{2}}{2} - \frac{x^{3}}{2}]}_{0}^{1} = \frac{1}{6}$

|

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

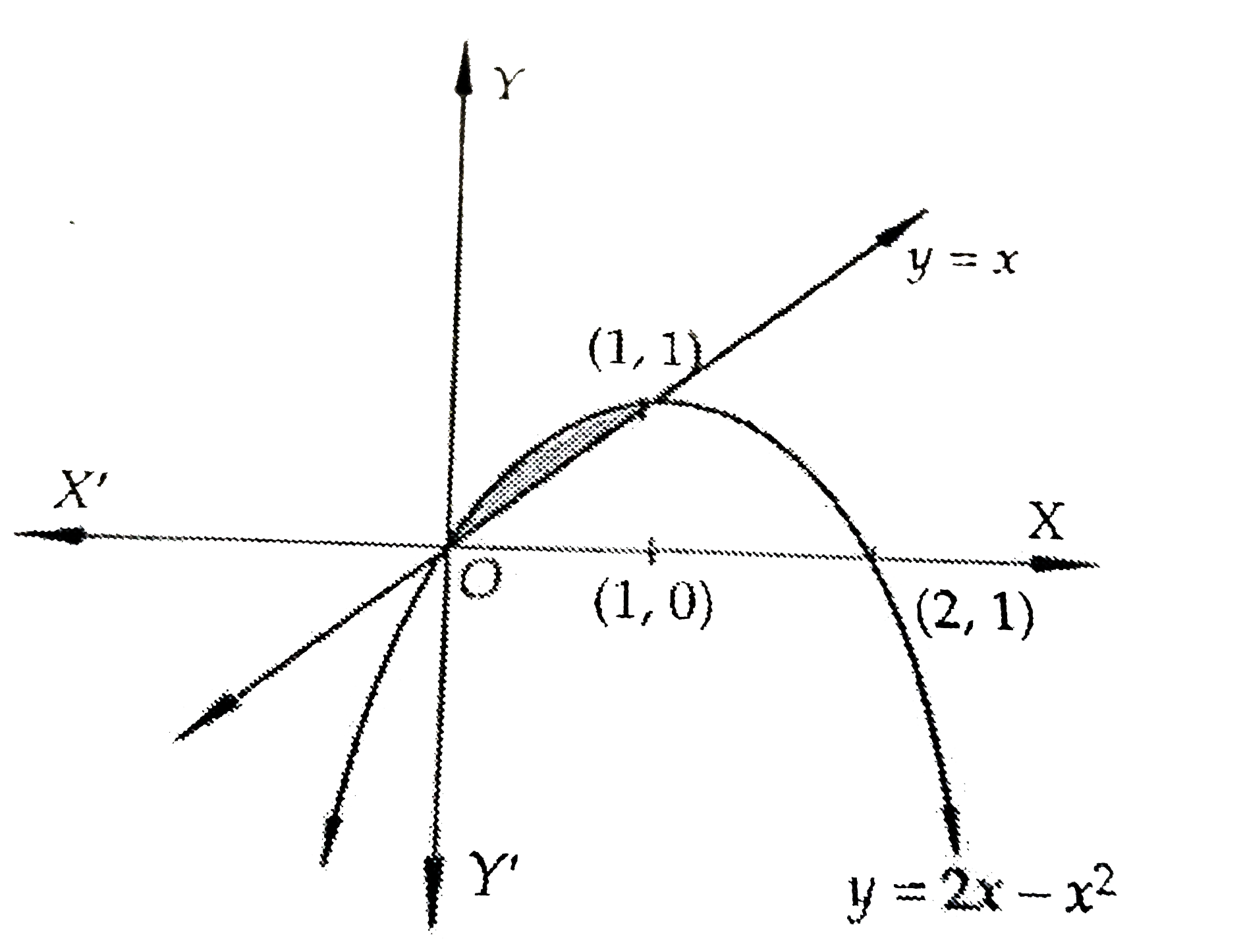
Topper's Solved these Questions

BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Exercise
61videos
AREAS OF BOUNDED REGIONS
BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Chapter Test
60videos
AREAS OF BOUNDED REGIONS
BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ALGEBRAIC INEQUATIONSEXERCISE - Exercise
39videos
ALGEBRAIC INEQUATIONS
BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test
30videos
CARTESIAN PRODUCT OF SETS AND RELATIONS

Similar Questions

OBJECTIVE RD SHARMA ENGLISH-AREAS OF BOUNDED REGIONS-Chapter Test

Home
Ask Doubt
Profile