The area of the region bounded by the curve y=x3 , and the lines , y=8 and x=0, is
16
8
10
12
The correct Answer is:D
Required area =8∫0xdy
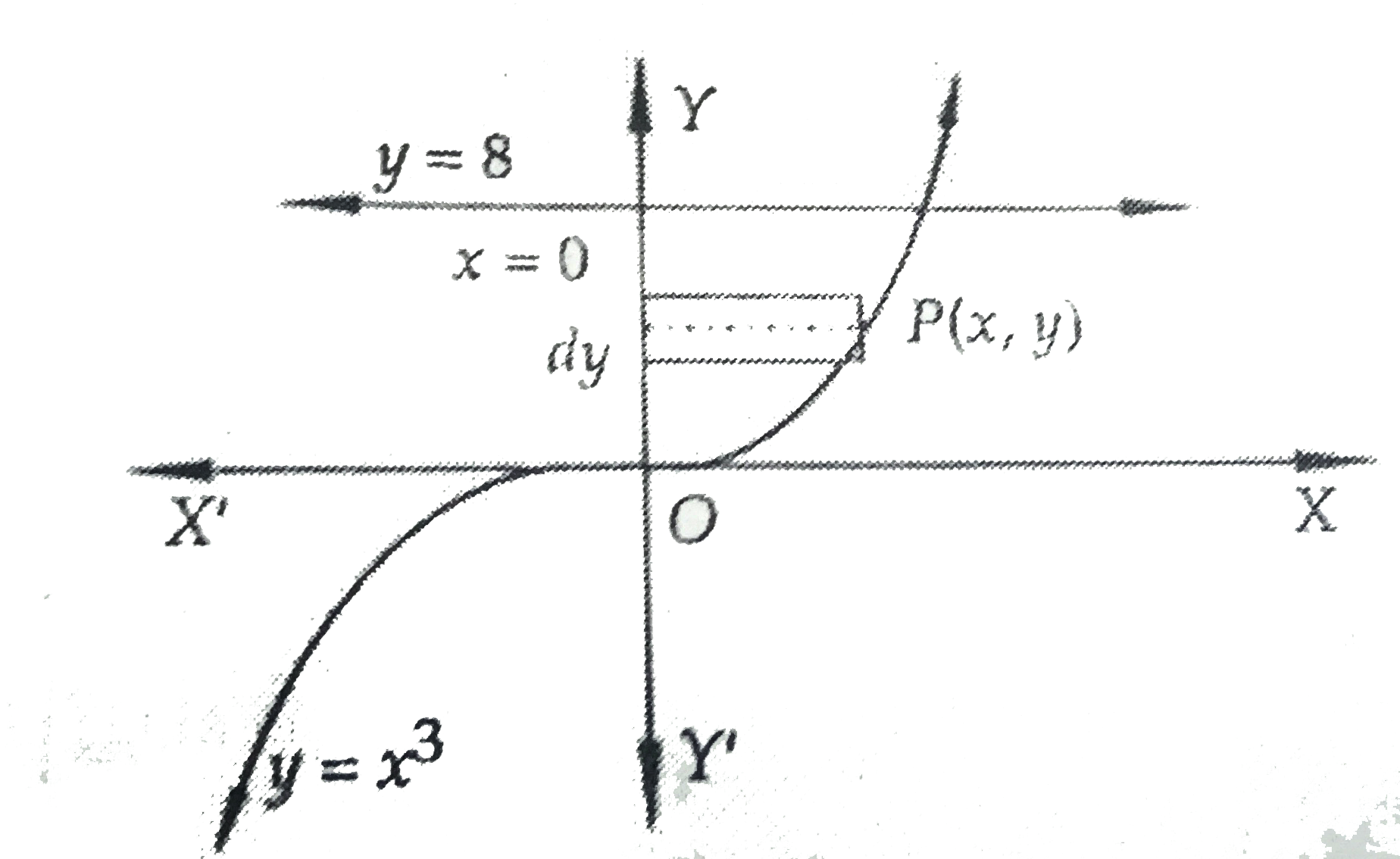
=8y01/4dy
=34[y4/3]80
=34×(84/3−0)=12
Topper's Solved these Questions
- AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Exercise
61 Videos
- AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Chapter Test
60 Videos
- ALGEBRAIC INEQUATIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ALGEBRAIC INEQUATIONSEXERCISE - Exercise
39 Videos
- CARTESIAN PRODUCT OF SETS AND RELATIONS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test
30 Videos
Similar Questions
Find the area of the region bounded by the curves y=x3 and the lines y=x+6 and y=0.
View SolutionThe area of the region bounded by the curve x=2y+3 and the lines y=1,y=−1 is
View SolutionFind the area of the region bounded by the curve y=x3 and the lines y = x + 6 and y =0.
View SolutionThe area of the region bounded by the curve y=ex and lines x=0 and y=e is
View SolutionThe area of the region bounded by the curve y=ex and lines x=0 and y=e is
View SolutionThe area of the region bounded by the curve y = x + 1 and the lines x=2,x=3, is
View SolutionFind the area of the region bounded by the curve y2=4xand the line x=3.
View SolutionThe area of the region bounded by the curve y=x3, X-axis and the ordinates x = 1, x = 4 is
View SolutionFind the area of the region bounded by the curve y=x2 and the line y = 4 .
View SolutionThe area of the region bounded by the curves y=xex,y=ex and the lines x=±1, is equal to
View Solution
