Let −−→AD be the angle bisector of the angle A of ΔABC, then −−→AD=α−−→AB+β−−→AC, where
α=∣∣∣−−→AB∣∣∣∣∣∣−−→AB∣∣∣+∣∣∣−−→AC∣∣∣,β=∣∣∣−−→AC∣∣∣∣∣∣−−→AB∣∣∣+∣∣∣−−→AC∣∣∣
α=∣∣∣−−→AB∣∣∣+∣∣∣−−→AC∣∣∣∣∣∣−−→AB∣∣∣,β=∣∣∣−−→AB∣∣∣+∣∣∣−−→AC∣∣∣∣∣∣−−→AC∣∣∣
α=∣∣∣−−→AC∣∣∣∣∣∣−−→AB∣∣∣+∣∣∣−−→AC∣∣∣,β=∣∣∣−−→AB∣∣∣∣∣∣−−→AB∣∣∣+∣∣∣−−→AC∣∣∣
α=∣∣∣−−→AB∣∣∣∣∣∣−−→AC∣∣∣,β=∣∣∣−−→AC∣∣∣∣∣∣−−→AB∣∣∣
The correct Answer is:C
Clearly, AD divides BC in the ratio AB : AC.
∴−−→AD=∣∣∣−−→AB∣∣∣−−→AC+∣∣∣−−→AC∣∣∣−−→AB∣∣∣−−→AB∣∣∣+∣∣∣−−→AC∣∣∣
⇒−−→AD=α−−→AB+β−−→AC, where
α=∣∣∣−−→AC∣∣∣∣∣∣−−→AB∣∣∣+∣∣∣−−→AC∣∣∣andβ=∣∣∣−−→AB∣∣∣∣∣∣−−→AB∣∣∣+∣∣∣−−→AC∣∣∣
Topper's Solved these Questions
- ALGEBRA OF VECTORSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ALGEBRA OF VECTORSEXERCISE - Exercise
30 Videos
- ALGEBRA OF VECTORSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ALGEBRA OF VECTORSEXERCISE - Chapter Test
30 Videos
- ALGEBRAIC INEQUATIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ALGEBRAIC INEQUATIONSEXERCISE - Exercise
39 Videos
Similar Questions
Let →AD be the angle bisector of ∠A of ΔABC such that →AD=α→AB+β→AC, then
View SolutionLet G be the centroid of Δ ABC , If −−→AB=→a,−−→AC=→b, then the −−→AG, in terms of →aand→b, is
View SolutionIf →a=7ˆi−4ˆj−4ˆk and →b=−2ˆi−ˆj+2ˆk, determine vector →c along the internal bisector of the angle between of the angle between vectors →aand→b such that |→c|=5√6
View SolutionE and F are the interior points on the sides BC and CD of a parallelogram ABCD. Let −−→BE=4−−→ECand−−→CF=4−−→FD. If the line EF meets the diagonal AC in G, then −−→AG=λ−−→AC, where λ is equal to :
View Solution−−→ACand−−→BD are the diagonals of a parallelogram ABCD. Prove that
(i)−−→AC+−−→BD−2−−→BC
(ii) −−→AC−−−→BD−2−−→AB
View SolutionIf ABCDEF is a regualr hexagon, then −−→AC+−−→AD+−−→EA+−−→FA=
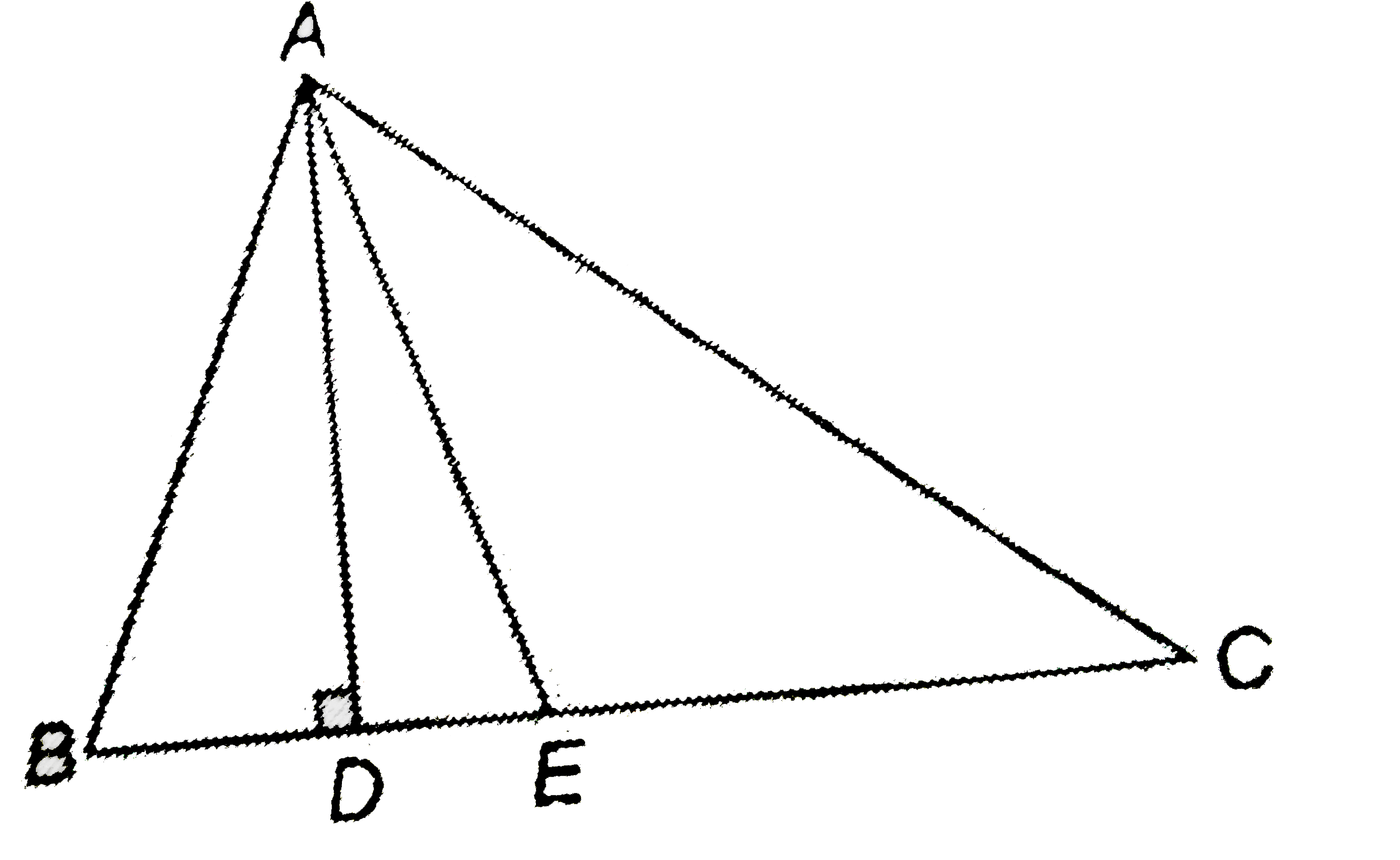
View SolutionIn the given figure, AD is altitude and AE is bisector of angle BAC of ΔABC. Show that ΔDAE=12(∠B−∠C)
View SolutionWhat is the angle be →a×→band→b×→a?
View SolutionLet triangle ABC be an isosceles with AB=AC. Suppose that the angle bisector of its angle B meets the side AC at a point D and that BC=BD+AD. Measure of the angle A in degrees, is :
View SolutionIf AD,BEandCF be the median of a △ABC, prove that −−→AD+−−→BE+−−→CF=0
View Solution
