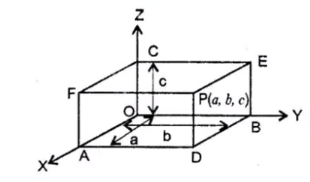
In fig. 28.7 if the coordinates of point P are (a,b,c) then Write the coordinates of points A, B, C, D, E and F. Write the coordinates of the feet of the perpendiculars from the point P to the coordinate axes. Write the coordinates of the feet of the perpendicular from the point P on the coordinate planes XY, YZ and ZX. Find the perpendicular distances of point P from XY,YZ and ZX− planes. Find the perpendicular distances of the point P fro the coordinate axes. Find the coordinates of the reflection of P are (a,b,c) . Therefore OA=a, OB=b nd OC=⋅
√a2+b2,√b2+c2,√c2+a2
√b2+c2,√c2+a2,√a2+b2
√c2+a2,√a2+b2,√b2+c2
a,b,c
The correct Answer is:B
PA,PB and PC are the perpendicular distances of point P from OX,OY and OZ respectively.
In right angled triangle ADP, we have
AP2=AD2+DP2
⇒PA=√AD2+DP2
⇒PA=√b2+c2[∵AD=OB=b and PD=OC=c]
In right angled triangle PDB right angled at D, we have
PB2=BD2+PD2
⇒PB=√BD2+PD2
=PB=√a2+c2[∵BD=OA=a and PD=OC=c]
In right angled triangle PCF right angled at F, we have
PC2=PF2+CF2
⇒PC=√PF2+CF2
⇒PC=√b2+a2[∵PF=AD=OB=b and CF=OA=a]
⇒PC=√a2+b2
Topper's Solved these Questions
- THREE DIMENSIONAL COORDINATE SYSTEM BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEM EXERCISE - Section I - Solved Mcqs
16 Videos
- THREE DIMENSIONAL COORDINATE SYSTEM BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEM EXERCISE - Exercise
20 Videos
- TANGENTS AND NORMALSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - TANGENTS AND NORMALSEXERCISE - Chapter Test
25 Videos
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Chapter Test
60 Videos
Similar Questions
Knowledge Check
The coordinate of the foot of the perpendicular from the point (a,b,c) on z-axis is
A(a,0,0)B(0,b,0)C(0,0,c)D(a,b,0)
In the adoining figure if the coordinates of point P are (a,b,c) write the coordinates of A,B,C,D,E and F.
View SolutionFind the equation of the perpendicular drawn from the point P(-2,3) to the line x-4y+7=0. Also, find the coordinates of the foot of the perpendicular.
View Solution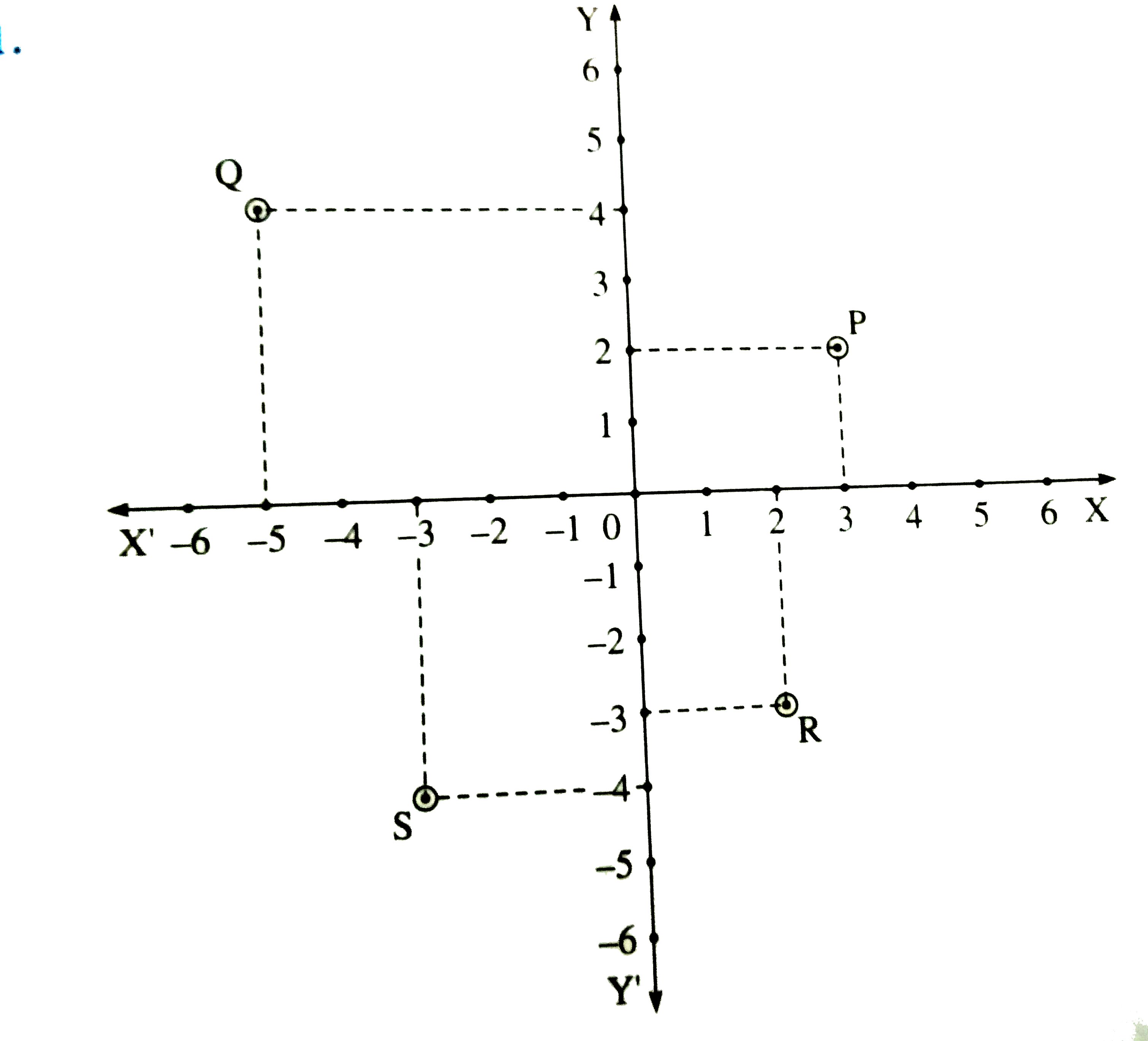
Observe the figure and write the coordinates of points P,Q,R and S.
View SolutionWrite the coordinates of the point which is the reflection of the point (a,b,c) in the xz-plane.
View SolutionFind the equation of the perpendicular drawn from the point P(2, 4, −1) to the line x+51=y+34=z−6−9 Also, write down the coordinates of the foot of the perpendicular from P.
View SolutionThe coordinates ofi as point P are (1,2,3). Find the coordinates for the seven points such that the absolute values of their coordinates are the same as those of coordinates of P.
View SolutionA point is on the x axis what are its y coordinate and z coordinates
View Solution

