Prove that the maximum number of points with rational coordinates on a circle whose center is (√3,0) is two.
one
two
four
infinite
The correct Answer is:B
Step by step video & image solution for Prove that the maximum number of points with rational coordinates on a circle whose center is (sqrt(3),0) is two. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCLESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise
132 Videos
- CARTESIAN PRODUCT OF SETS AND RELATIONS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test
30 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - COMPLEX NUMBERS EXERCISE - Chapter Test
58 Videos
Similar Questions
Knowledge Check
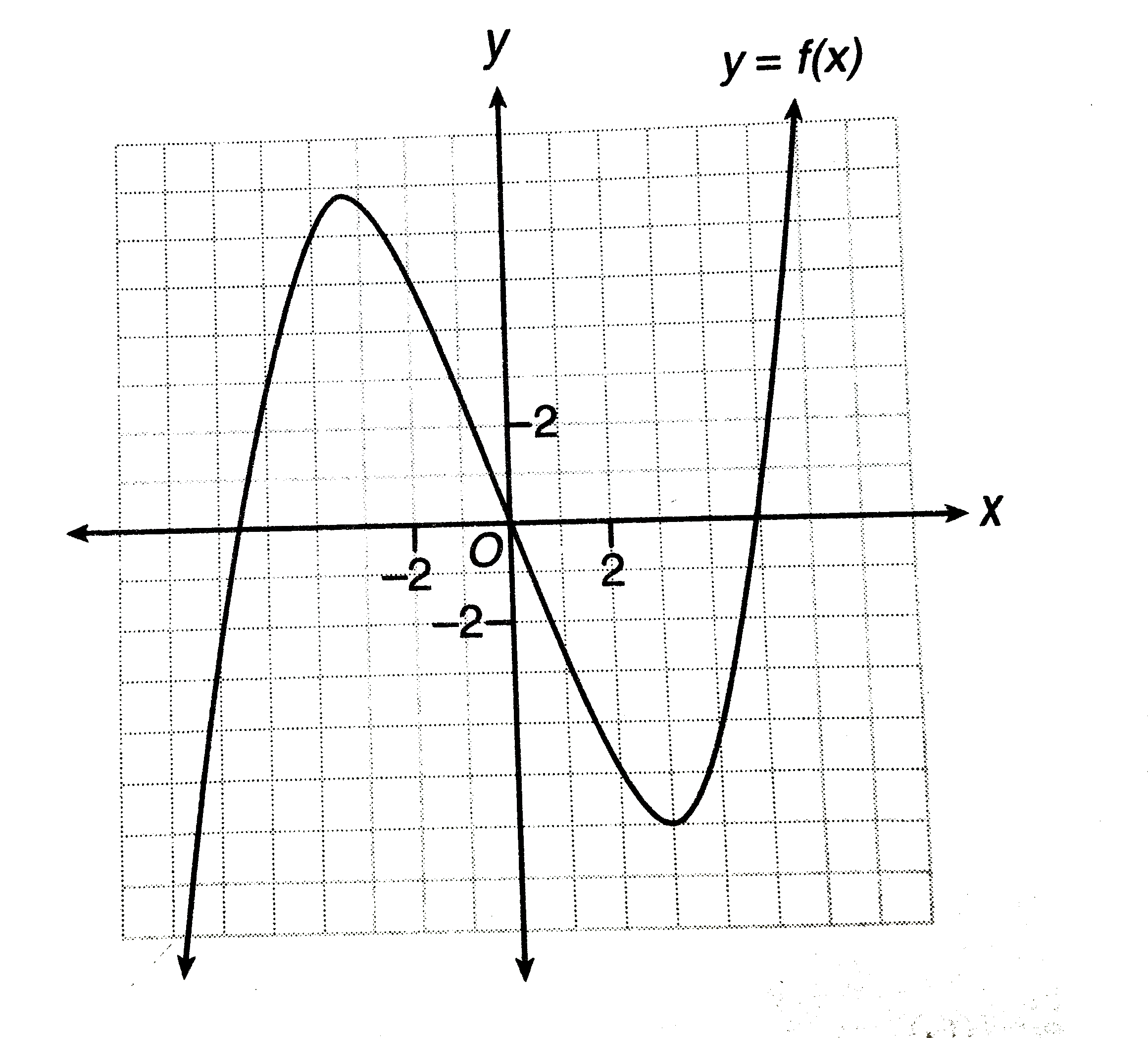
16-18 The graph of polynomial function f is shown above.
Q. What is the maximum number of points a circle whose center is at the origin can intersect the graph of y=f(x)?A2B3C4D6How many points with integer coordinates lie on or inside a circle of radius three units centered at the origin?
A16B28C29D30
Le n be the number of points having rational coordinates equidistant from the point (0,√3), the
View SolutionFind the coordinates of a point A, where AB is the diameter of a circle whose centre is (2, 3) and B is (1, 4).
View SolutionFind the polar coordinates of the points whose cartesian coordinates are
(−3,4)
View SolutionThe quadratic equation with rational coefficients whose one root is 3+√2 is
View SolutionFind the coordinates of a point A , here AB is a diameter of the circle whose centre is (2, −3) and B(1, 4) .
View SolutionProve that √n is not a rational number. if n is not a perfect square.
View Solution
