Twenty metres of wire is available for fencing off a flower-bed in the form of a circular sector. Then the maximum area (in sq.m) of the flower-bed is: (1) 25 (2) 30 (3) 12.5 (4) 10
12.5
10
25
30
The correct Answer is:C
Let r be the radius and θ ( in radians ) be the sector angle of the circular sector. Further let A be the area of the sector . Then
A=12r2θ

If is given that perimeter of the sector of the of OAB is 20 meters
∴r+rθ=20⇒=20−2rr
From (i) and (ii) we obtain
A=12r2(20−2rr)≠10r−r2
⇒dAdr=10−2randd2Adr2=−2
For maximum and minimum values of A we must have
dAdr=0⇒10−2r−0⇒=5
Putting r=5 in (ii) we obtain θ=2
∴A=12×52×2=25m2
Topper's Solved these Questions
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Section I - Solved Mcqs
72 Videos
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Section II - Assertion Reason Type
7 Videos
- MATHEMATICAL REASONING BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MATHEMATICAL REASONING EXERCISE - Chapter Test
20 Videos
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Chapter Test
21 Videos
Similar Questions
A wire of length 20m is available to fence off a flower bed in the form of a sector of a circle. What must be the radius of the circle, if we wish to have a flower bed with the greatest possible area?
View SolutionIn Fig. 19, the radius of quarter circular plot taken is 2 m and radius of the flower bed is 2 m. Find the area of the remaining field.
View SolutionFind maximum possible area that can be enclosed by a wire of length 20cm by bending it in form of a circular sector. 10 (b) 25 (c) 30 (d) 20
View SolutionIf one of the diameters of the circle, given by the equation, x2+y2−4x+6y−12=0 , is a chord of a circle S, whose centre is at (−3,2) , then the radius of S is : (1) 5√2 (2) 5√3 (3) 5 (4) 10
View SolutionThe shaded region of the given diagram represents the lawn in the form of a house. On the three sides of the lawn there are flower-beds having a uniform width of 2 m.
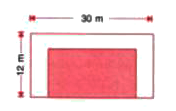
Hence, or otherwise, find the area of the flower- beds.
View SolutionMaximum possible area that can be enclosed by a wire of length 20 cm by bending it in form of a circular sector is 10 b. 25 c. 30 d. 20
View SolutionIf one side of a rhombus has endpoints (4, 5) and (1, 1), then the maximum area of the rhombus is 50 sq. units (b) 25 sq. units 30 sq. units (d) 20 sq. units
View SolutionIf one side of a rhombus has endpoints (4, 5) and (1, 1), then the maximum area of the rhombus is 50 sq. units (b) 25 sq. units 30 sq. units (d) 20 sq. units
View SolutionIf the mid-point of a chord of the ellipse x216+y225=1 (0, 3), then length of the chord is (1) 325 (2) 16 (3) 45 12 (4) 32
View SolutionA circular flower bed is surrounded by a path 4m wide. The diameter of the flower bed is 66m. What is the area of this path ? (π=3.14)
View Solution
