If z is a complex number having least absolute value and ∣z−2+2i=1,thenz= (2−1/√2)(1−i) b. (2−1/√2)(1+i) c. (2+1/√2)(1−i) d. (2+1/√2)(1+i)
(2−1√2)(1−i)
(2−1√2)(1+i)
(2+1√2)(1−i)
(2+1√2)(1+i)
The correct Answer is:A
We have,
|z−2+2i|=1
⇒|z−(2−2i)|=1
⇒ z lies on a circle having center at (2,−2) and radius 1.
It is evident from the figure that the required complex number z s given by the point P. We find that OP makes an angle π/4 with OX and
OP=OC-CP = √22+22−1=2√2−1
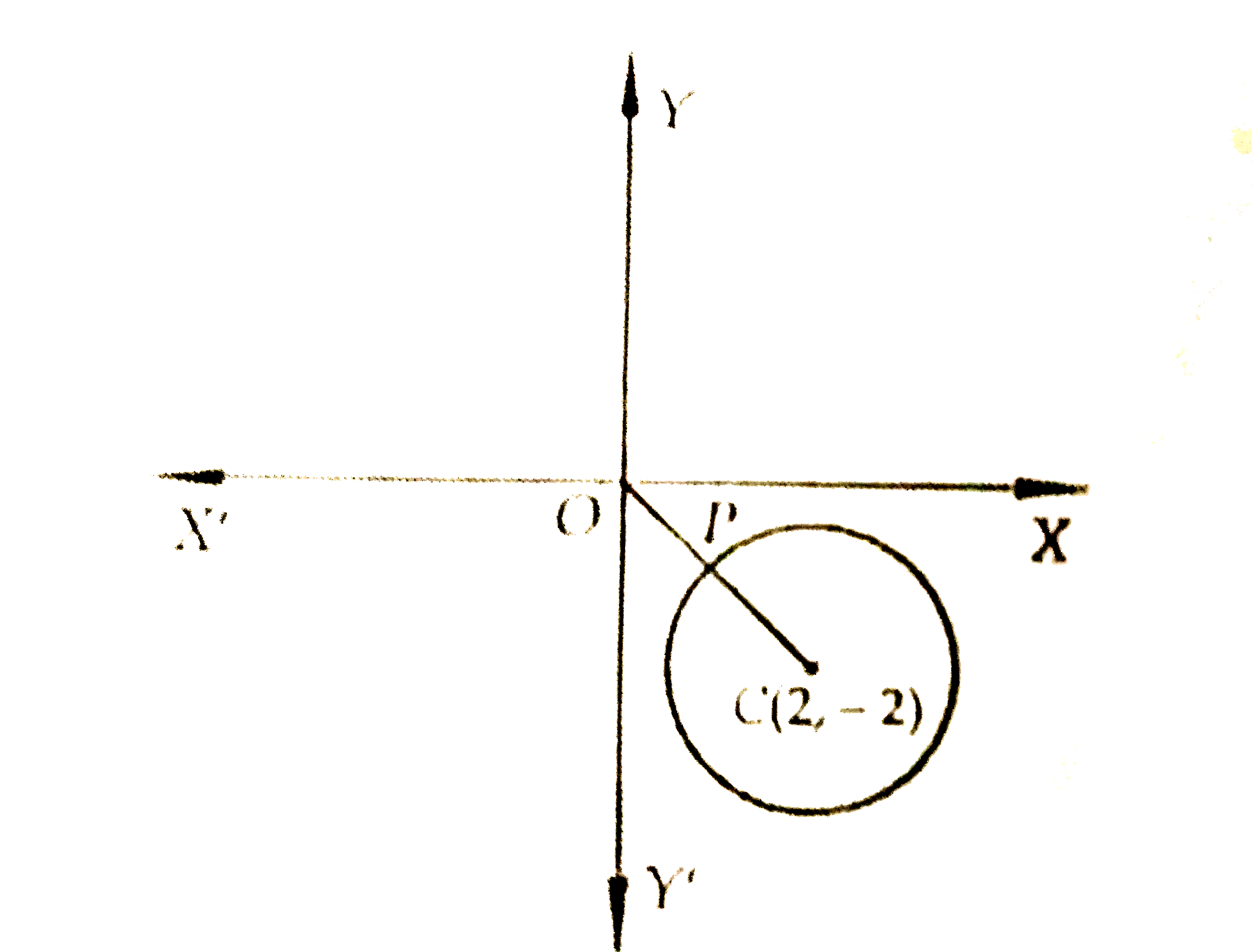
So, coordinate of P are
(2√2−1)cosπ4,−(2√2−1)sinπ4)
i.e., (2−1√2),−(2−1√2)
Hence, z=(2−1√2)+{−(2−1√2)}i=(2−1√2)1=i
Topper's Solved these Questions
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - COMPLEX NUMBERS EXERCISE - Section I - Solved Mcqs
141 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - COMPLEX NUMBERS EXERCISE - Section II - Assertion Reason Type
15 Videos
- CIRCLESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CIRCLESEXERCISE - Chapter Test
53 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise
87 Videos
Similar Questions
If z is a complex number having least absolute value and |z−2+2i|=1,then z= (2−1/√2)(1−i) b. (2−1/√2)(1+i) c. (2+1/√2)(1−i) d. (2+1/√2)(1+i)
View SolutionValues (s)(−i)13 is/are √3−i2 b. √3+i2 c. −√3−i2 d. −√3+i2
View SolutionFind a complex number z satisfying the equation z+√2|z+1|+i=0.
View SolutionFind a complex number z satisfying the equation z+√2|z+1|+i=0.
View SolutionProve that : (i) √i=1+i√2 (ii) √−i=1−i√2 (iii) √i+√−i=√2
View SolutionIf z is a complex number such that |z|=4 and arg(z)=5π6 , then z is equal to −2√3+2i b. 2√3+i c. 2√3−2i d. −√3+i
View SolutionIf z is a complex number such that |z|=4 and arg(z)=5π6 , then z is equal to −2√3+2i b. 2√3+i c. 2√3−2i d. −√3+i
View SolutionWhich term of the G.P.: √2,1√2,12√2,14√2,is1512√2?
View SolutionMaximum value of |z+1+i|, where z∈S is
(a)√2
(b) 2
(c) 2√2
(d) 3√2
View SolutionExpress the following complex numbers in the standard form a+ib:5+√2i1−√2i
View Solution
