An isosceles triangle of wood of base 2a and height h is placed with its base on the ground vertex directly above. The triangle faces the sun whose altitude is 30∘. Then, the tangent of the angle at the apex of the shadow is
2ah√3
2√3ah3h2−a2
a2+h22√3
2√3ah3h2+a2
The correct Answer is:B
Let ABC be the isosceles triangle with base BC=2a and height AD=h.
Let BCE be the shadow of △ABC such that E is the apex of the shadow.
Clearly, DE is the height of the triangular shadow.
Let 2α be the angle at the apex E of the shadow. Then,
tanα=aDE

Since the altitude of the sun is 30∘ . Therefore,
tan30∘=ADDE⇒1√3=hDE⇒DE=√3h
∴tanα=a√3h
Now,
tan2α=2tanα1−tan2α⇒tan2α=2a/√3h1−a23h2=2√3ah3h2−a2
Topper's Solved these Questions
- HEIGHTS AND DISTANCESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - HEIGHTS AND DISTANCESEXERCISE - Exercise
45 Videos
- EXPONENTIAL AND LOGARITHMIC SERIESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - EXPONENTIAL AND LOGARITHMIC SERIESEXERCISE - Chapter Test
20 Videos
- INCREASING AND DECREASING FUNCTIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - INCREASING AND DECREASING FUNCTIONSEXERCISE - Chapter Test
18 Videos
Similar Questions
An isosceles triangle of wood of base 10 feet and height 8√3 feet is placed vertically with its base on the ground and vertex directly above. The triangle faces the sun whose altitude is 30∘. Then, the tangent of the angle at the apex of the shadow is
View SolutionAn isosceles triangle made of wood of base 10 feet and height 8 feet is placed vertically with its base on the ground and vertex directly above it. If the triangle faces the sun whose altitude is 30∘, then the tangent of the angle at the apex of the shadow is
View SolutionIn an isosceles triangle, if the vertex angle is twice the sum of the base angles, calculate the angles of the triangle.
View SolutionThe vertical angle of an isosceles triangle is twice the sum if its base angles. Find each angle of the triangle.
View SolutionABC is an isosceles triangle. If the coordinates of the base are B(1, 3) and C(-2, 7). The coordinates of vertex A can be
View SolutionConstruct an isosceles triangle whose base is 6 cm and altitude is 4 cm.
View SolutionThe figure shows an isosceles triangle plate of mass M and base L.
The angle at the apex is 90∘. The apex lies at the origin and the base is parallel to X−axis.
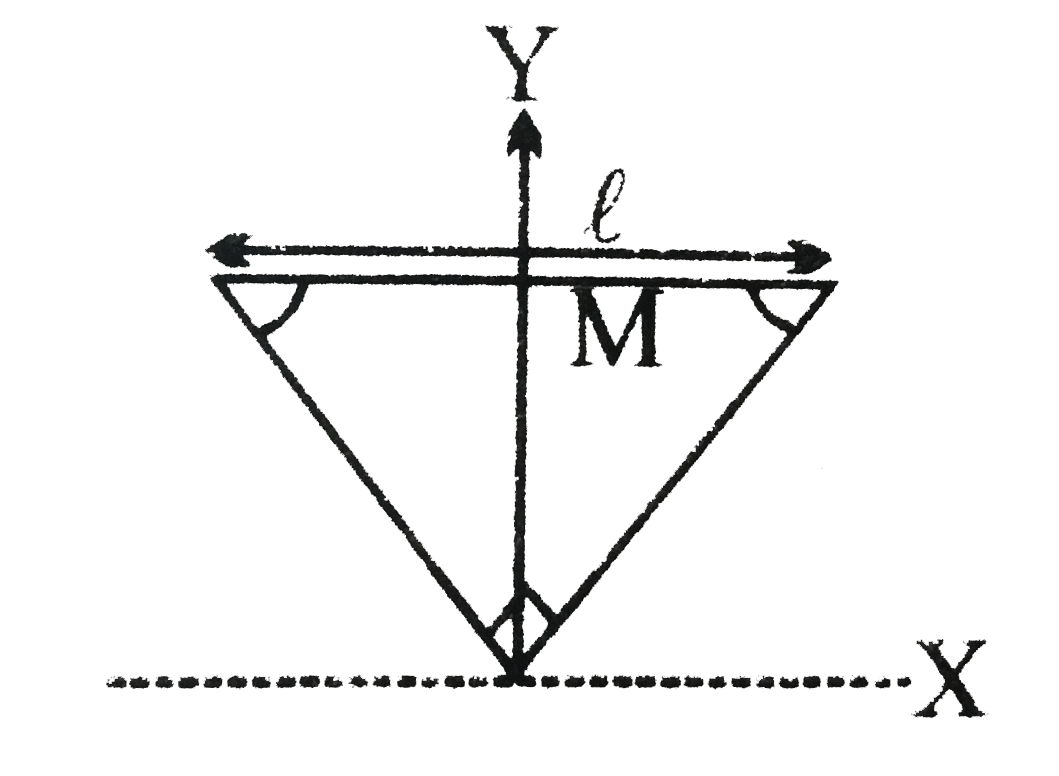
The moment of inertia of the plate about its base parallel to the x−axis is
View SolutionFind the locus of the vertex of a triangle whose, sum of base angles is fixed.
View SolutionThe base and the heigth of a triangle are in the ratio 5:3 and its are is 43.2m2 . Find the base and the height of the triangle .
View SolutionABC is an isosceles triangle. If the coordinates of the base are B(1,3) and C(−2,7) , the coordinates of vertex A
View Solution
