State True or False
Mean, Median and Mode may be the same for some data.
To determine whether the statement "Mean, Median and Mode may be the same for some data" is true or false, we can analyze the definitions of mean, median, and mode, and provide examples to illustrate the concept.
1. Understanding the Terms:
- Mean: This is the average of a set of numbers. It is calculated by adding all the numbers together and dividing by the total count of numbers.
- Median: This is the middle value of a data set when the numbers are arranged in order. If there is an odd number of observations, the median is the middle number. If there is an even number of observations, it is the average of the two middle numbers.
- Mode: This is the number that appears most frequently in a data set.
2. Example with Different Values:
- Consider the data set: 1, 2, 3, 4, 4.
- Mean: (1 + 2 + 3 + 4 + 4) / 5 = 14 / 5 = 2.8
- Median: The middle value (3rd term) in the ordered list (1, 2, 3, 4, 4) is 3.
- Mode: The number that appears most frequently is 4.
- In this case, Mean = 2.8, Median = 3, Mode = 4. They are not the same.
3. Example with Same Values:
- Now consider the data set: 4, 4, 4, 4, 4.
- Mean: (4 + 4 + 4 + 4 + 4) / 5 = 20 / 5 = 4
- Median: The middle value (3rd term) in the ordered list (4, 4, 4, 4, 4) is 4.
- Mode: The number that appears most frequently is 4.
- In this case, Mean = 4, Median = 4, Mode = 4. They are the same.
4. Conclusion:
- From the examples, we can see that there are cases where the mean, median, and mode can be the same (as in the second example) and cases where they are different (as in the first example).
- Therefore, the statement "Mean, Median and Mode may be the same for some data" is True.
Final Answer:
True
- Mean: This is the average of a set of numbers. It is calculated by adding all the numbers together and dividing by the total count of numbers.
- Median: This is the middle value of a data set when the numbers are arranged in order. If there is an odd number of observations, the median is the middle number. If there is an even number of observations, it is the average of the two middle numbers.
- Mode: This is the number that appears most frequently in a data set.
- Consider the data set: 1, 2, 3, 4, 4.
- Mean: (1 + 2 + 3 + 4 + 4) / 5 = 14 / 5 = 2.8
- Median: The middle value (3rd term) in the ordered list (1, 2, 3, 4, 4) is 3.
- Mode: The number that appears most frequently is 4.
- In this case, Mean = 2.8, Median = 3, Mode = 4. They are not the same.
- Now consider the data set: 4, 4, 4, 4, 4.
- Mean: (4 + 4 + 4 + 4 + 4) / 5 = 20 / 5 = 4
- Median: The middle value (3rd term) in the ordered list (4, 4, 4, 4, 4) is 4.
- Mode: The number that appears most frequently is 4.
- In this case, Mean = 4, Median = 4, Mode = 4. They are the same.
- From the examples, we can see that there are cases where the mean, median, and mode can be the same (as in the second example) and cases where they are different (as in the first example).
- Therefore, the statement "Mean, Median and Mode may be the same for some data" is True.
True
Topper's Solved these Questions
- DATA HANDLINGBOOK - NCERT EXEMPLARCHAPTER - DATA HANDLINGEXERCISE - EXERCISE
42 Videos
- DATA HANDLINGBOOK - NCERT EXEMPLARCHAPTER - DATA HANDLINGEXERCISE - THINK AND DISCUSS
3 Videos
- DATA HANDLINGBOOK - NCERT EXEMPLARCHAPTER - DATA HANDLINGEXERCISE - EXERCISE(FILL IN THE BLANKS)
15 Videos
- COMPARING QUANTITIESBOOK - NCERT EXEMPLARCHAPTER - COMPARING QUANTITIESEXERCISE - THINK AND DISCUSS
24 Videos
- EXPONENTS AND POWERSBOOK - NCERT EXEMPLARCHAPTER - EXPONENTS AND POWERSEXERCISE - EXERCISE
136 Videos
Similar Questions
State True or False:
Median of the data may or may not be from the given data.
View SolutionFind the mean, median and mode of the following data.
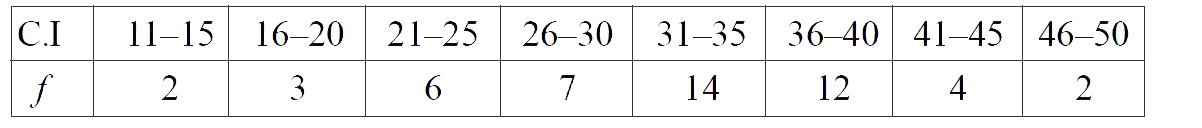
View SolutionFind the mean, median and mode of the following data :
View SolutionFind the mean, median and mode of the following data :

View SolutionFind the mean, median and mode of the following data
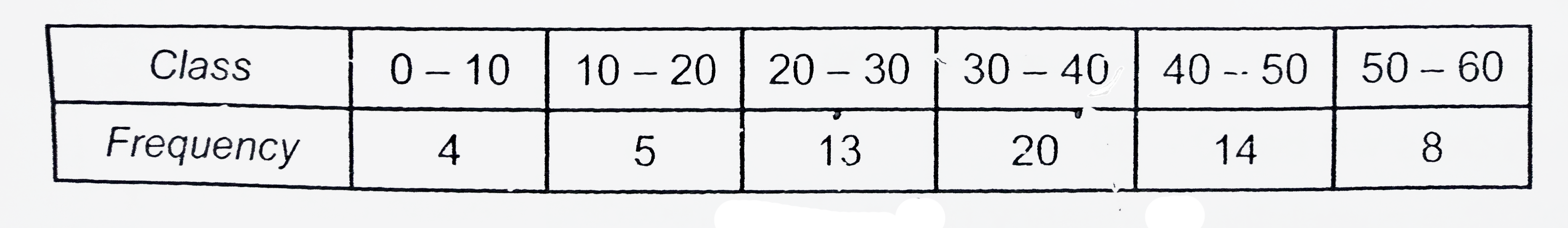
View SolutionFind the mean, median, and mode for the follow ing data.

View SolutionFind the mean, median and mode of the following data:
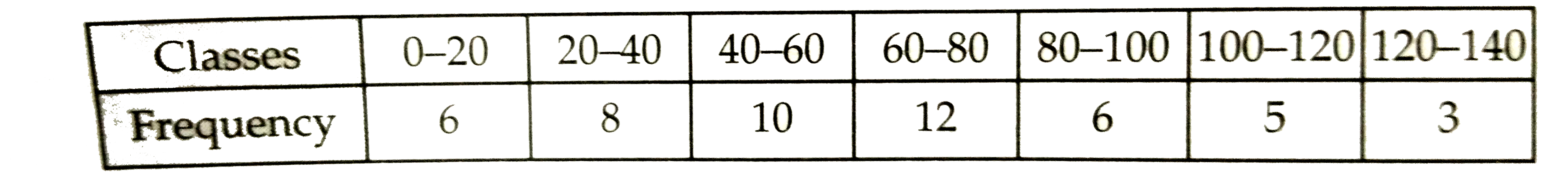
View SolutionState True or False:
Mean of the data is always from the given data.
View SolutionWrite the relationship among Mean, Median and Mode. If the mean of data is 27 and Medianis is 33, then find the mode.
View SolutionState True or False:
Range of the data is always from the data.
View Solution
