The area of a circle is given by the expression πx2+6πx+9π. Find the radius of the circle.
Step by step video & image solution for The area of a circle is given by the expression pi x ^(2) + 6pi x + 9pi. Find the radius of the circle. by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The radius of a circle is greater than the radius of other circle by 3 m. The sum of their areas is 89πm2 . Find the radius of each circle.
View SolutionThe area of a circle is given by A=πr2, where r is the radius. Calculate the rate of increase of area w.r.t radius.
View SolutionFind the area of the segment of a circle, given that the angle of the sector is 120o and the radius of the circle is 21 cm. (Take π=22/7)
View SolutionFind the area of a circle whose circumference is 8π
View SolutionThe area of a square is equal to the area of a circle. The ratio between the side of the square and the radius of the circle is (a) √π:1 (b) 1:√π (c) 1:π (d) π:1
View SolutionIf the area of a circle increases from 9π to 16π then what will be the ratio of the circumference of the first circle to the second circle
View SolutionAn arc of a circle is a length 5πcm and the sector it bounds has an area of 20πcm2 . Find the radius of the circle.
View Solution(i) Find the area of a circle of radius 21 cm. [Takeπ=227]
(ii) Find the area of a circle of radius 10 cm. [Takeπ=3.14]
View SolutionIn the figure, if O is the centre of the circle, PQ is chord. ∠POQ=90∘ area of shaded region is 114cm2 find the radius of the circle
(π=3.14)
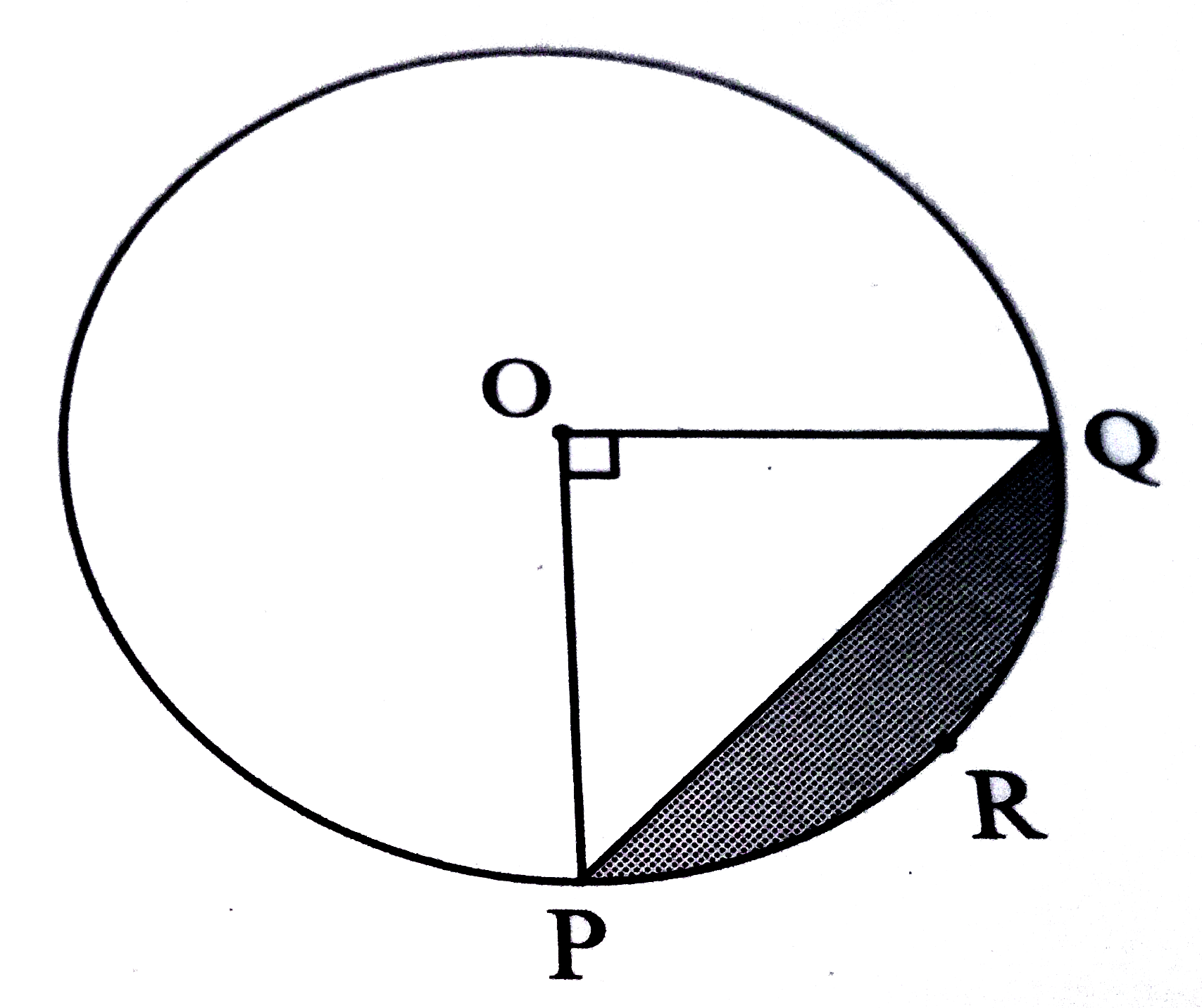
View SolutionTwo circles touch internally. The sum of their areas is 116π cm2 and distance between their centres is 6 cm. Find the radii of the circles.
View Solution
